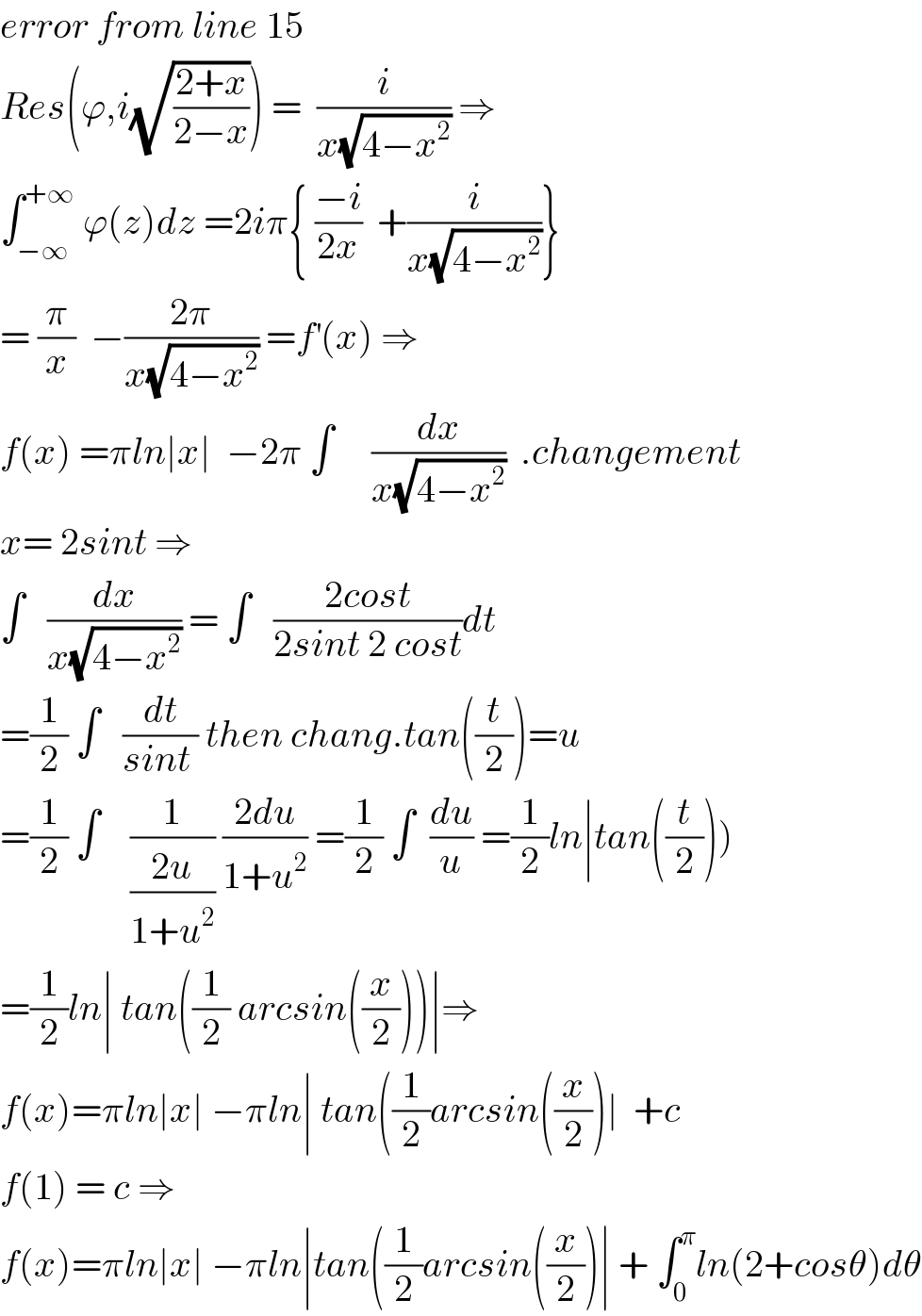Question Number 38718 by maxmathsup by imad last updated on 28/Jun/18
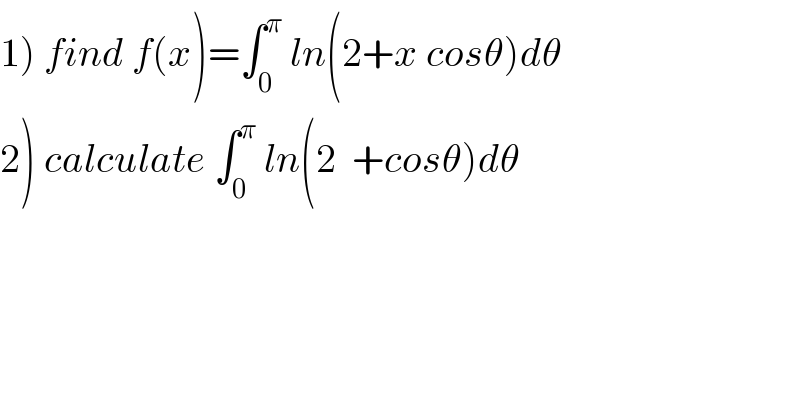
Commented by math khazana by abdo last updated on 30/Jun/18
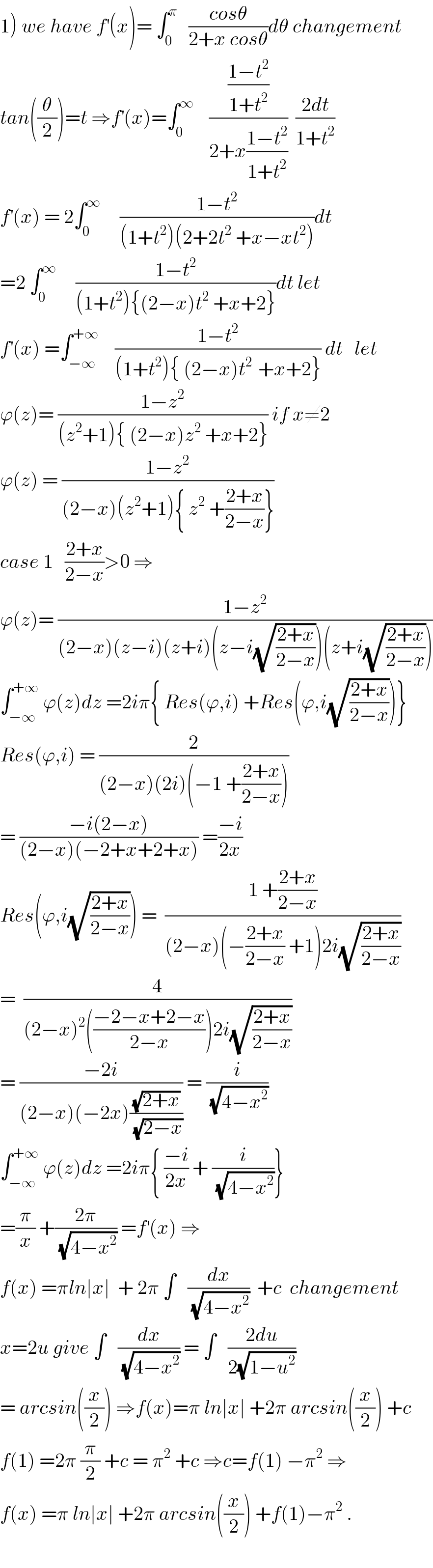
Commented by math khazana by abdo last updated on 30/Jun/18
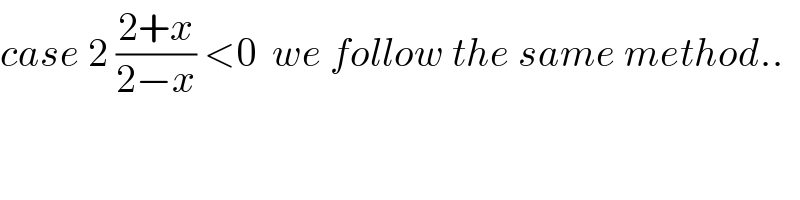
Commented by math khazana by abdo last updated on 30/Jun/18