Question Number 125056 by benjo_mathlover last updated on 08/Dec/20
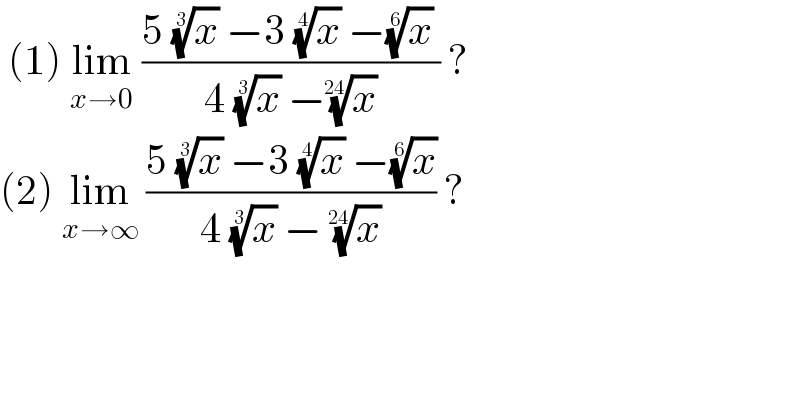
$$\:\left(\mathrm{1}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{5}\:\sqrt[{\mathrm{3}}]{{x}}\:−\mathrm{3}\:\sqrt[{\mathrm{4}}]{{x}}\:−\sqrt[{\mathrm{6}}]{{x}}\:}{\mathrm{4}\:\sqrt[{\mathrm{3}}]{{x}}\:−\sqrt[{\mathrm{24}}]{{x}}}\:? \\ $$$$\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\mathrm{5}\:\sqrt[{\mathrm{3}}]{{x}}\:−\mathrm{3}\:\sqrt[{\mathrm{4}}]{{x}}\:−\sqrt[{\mathrm{6}}]{{x}}}{\mathrm{4}\:\sqrt[{\mathrm{3}}]{{x}}\:−\:\sqrt[{\mathrm{24}}]{{x}}}\:?\: \\ $$
Answered by bramlexs22 last updated on 08/Dec/20
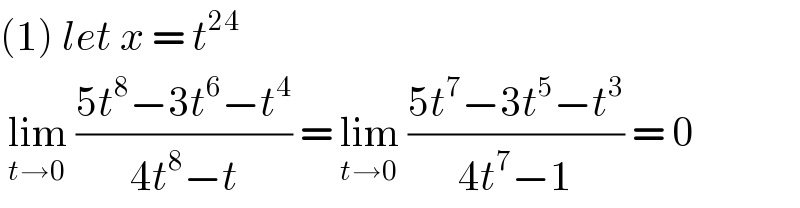
$$\left(\mathrm{1}\right)\:{let}\:{x}\:=\:{t}^{\mathrm{24}} \\ $$$$\:\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{5}{t}^{\mathrm{8}} −\mathrm{3}{t}^{\mathrm{6}} −{t}^{\mathrm{4}} }{\mathrm{4}{t}^{\mathrm{8}} −{t}}\:=\:\underset{{t}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{5}{t}^{\mathrm{7}} −\mathrm{3}{t}^{\mathrm{5}} −{t}^{\mathrm{3}} }{\mathrm{4}{t}^{\mathrm{7}} −\mathrm{1}}\:=\:\mathrm{0} \\ $$
Commented by bramlexs22 last updated on 08/Dec/20
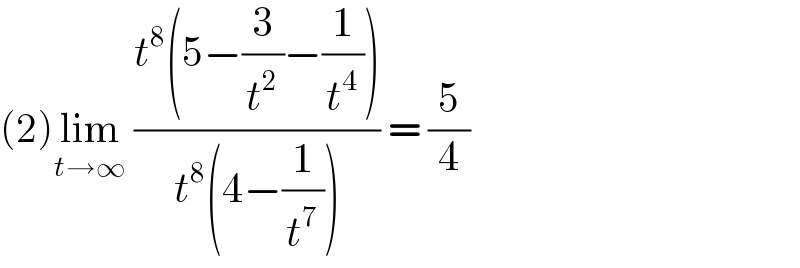
$$\left(\mathrm{2}\right)\underset{{t}\rightarrow\infty} {\mathrm{lim}}\:\frac{{t}^{\mathrm{8}} \left(\mathrm{5}−\frac{\mathrm{3}}{{t}^{\mathrm{2}} }−\frac{\mathrm{1}}{{t}^{\mathrm{4}} }\right)}{{t}^{\mathrm{8}} \left(\mathrm{4}−\frac{\mathrm{1}}{{t}^{\mathrm{7}} }\right)}\:=\:\frac{\mathrm{5}}{\mathrm{4}} \\ $$
