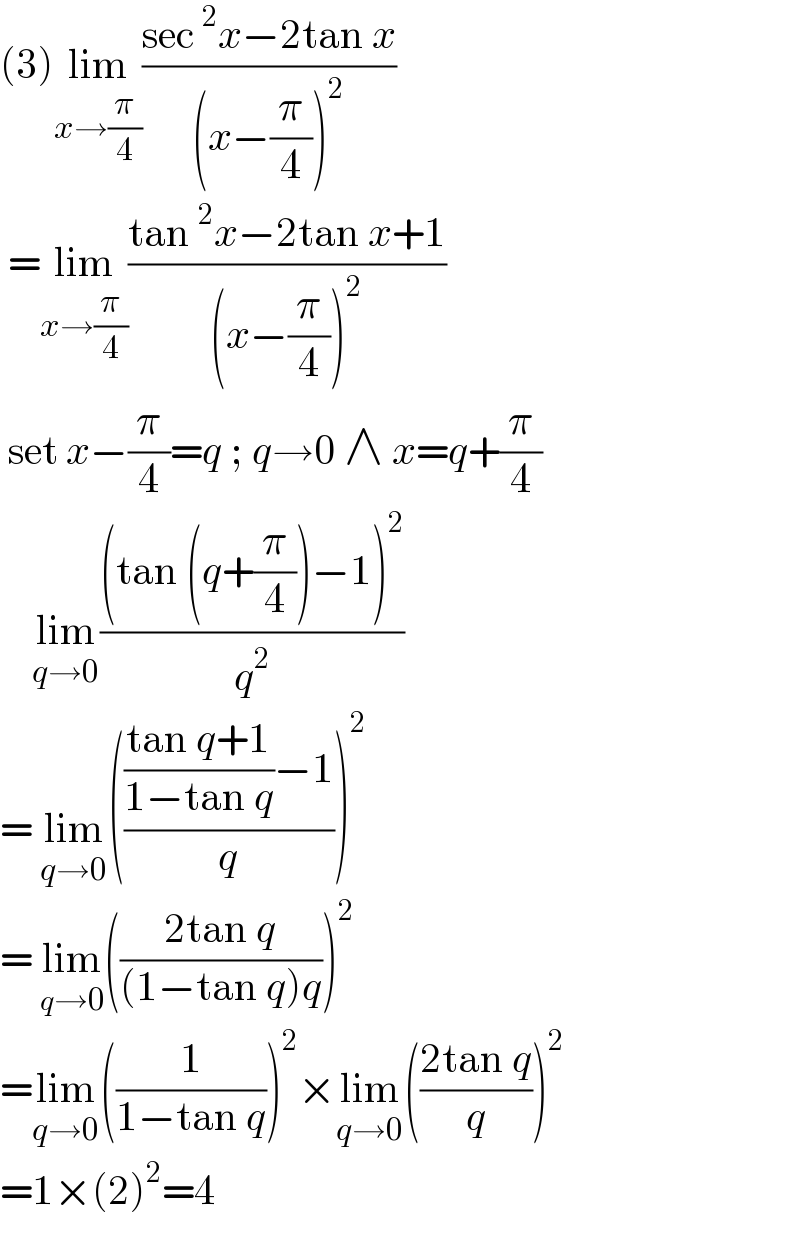1-lim-x-pi-2-cos-4x-cos-2x-2-2x-pi-2-2-lim-x-0-sin-3x-sin-6x-sin-9x-x-3-3-lim-x-pi-4-sec-2-x-2tan-x-x-pi-4-2-4-lim-x-0-12-6x-2-12cos-x-x-4-5-lim-x-

Question Number 147093 by liberty last updated on 18/Jul/21

(1) \lim_{x \to π / 2} \frac{\cos 4 x - \cos 2 x - 2}{{(2 x - π)}^{2}} = ?

(2) \lim_{x \to 0} \frac{\sin 3 x + \sin 6 x - \sin 9 x}{x^{3}} = ?

(3) \lim_{x \to π / 4} \frac{\sec^{2} x - 2 \tan x}{{(x - \frac{π}{4})}^{2}} = ?

(4) \lim_{x \to 0} \frac{12 - 6 x^{2} - 12 \cos x}{x^{4}} = ?

(5) \lim_{x \to 0} \frac{\sin^{2} x - \sin^{2} 2 x + 3 x^{2}}{x^{4}} = ?

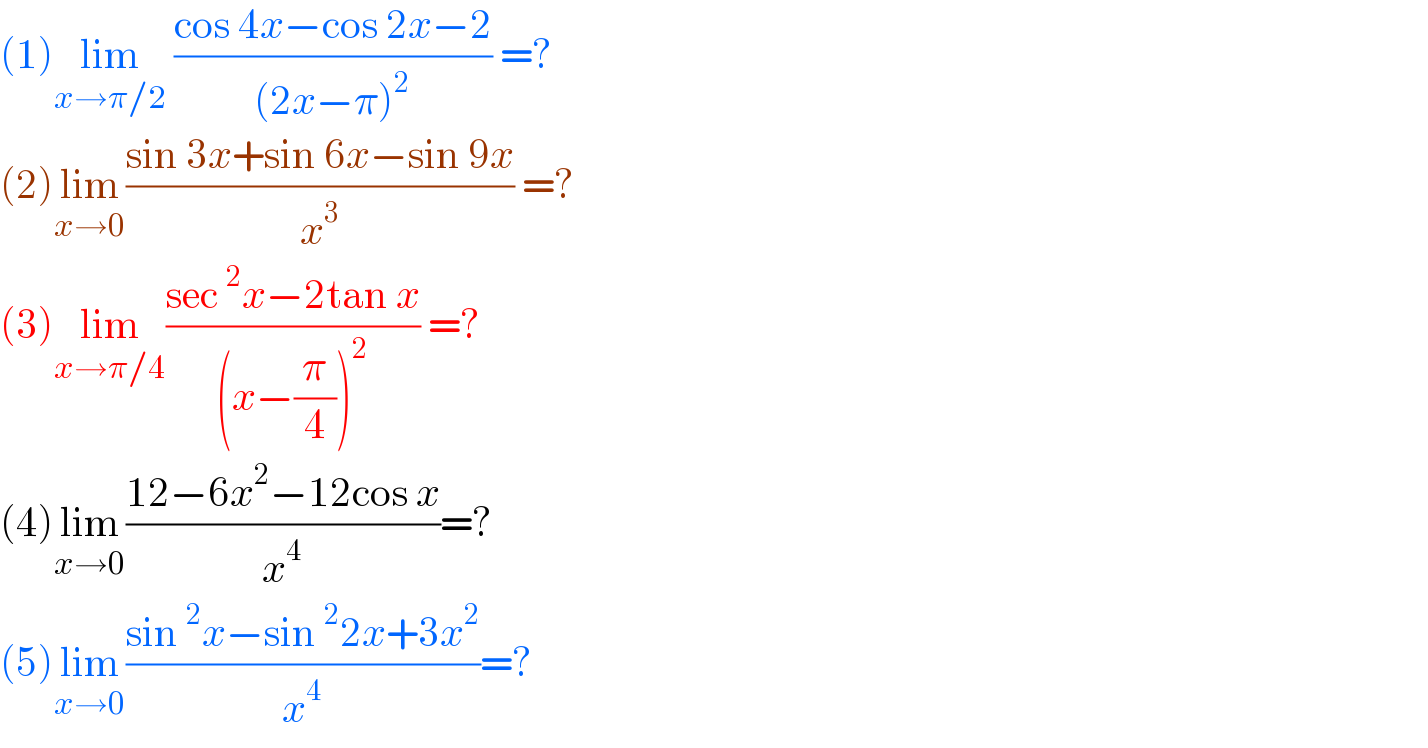
Commented by mathmax by abdo last updated on 18/Jul/21

f (x) = \frac{\cos (4 x) - \cos (2 x) - 2}{{(2 x - π)}^{2}} \Rightarrow f (x) = \frac{\cos (4 x) - \cos (2 x) - 2}{4 {(x - \frac{π}{2})}^{2}}

=_{x - \frac{π}{2} = t} \frac{\cos (4 (\frac{π}{2} + t)) - \cos (2 (\frac{π}{2} + t)) - 2}{{4 t}^{2}} = g (t) (t \to 0)

\Rightarrow g (t) = \frac{\cos (4 t) + \cos (2 t) - 2}{{4 t}^{2}} \Rightarrow

g (t) \sim \frac{1 - \frac{{(4 t)}^{2}}{2} + 1 - \frac{{(2 t)}^{2}}{2} - 2}{{4 t}^{2}} = \frac{- {8 t}^{2} - {2 t}^{2}}{{4 t}^{2}} = \frac{- 10}{4} = - \frac{5}{2} \Rightarrow

\lim_{t \to 0} g (t) = - \frac{5}{2} = \lim_{x \to \frac{π}{2}} f (x)

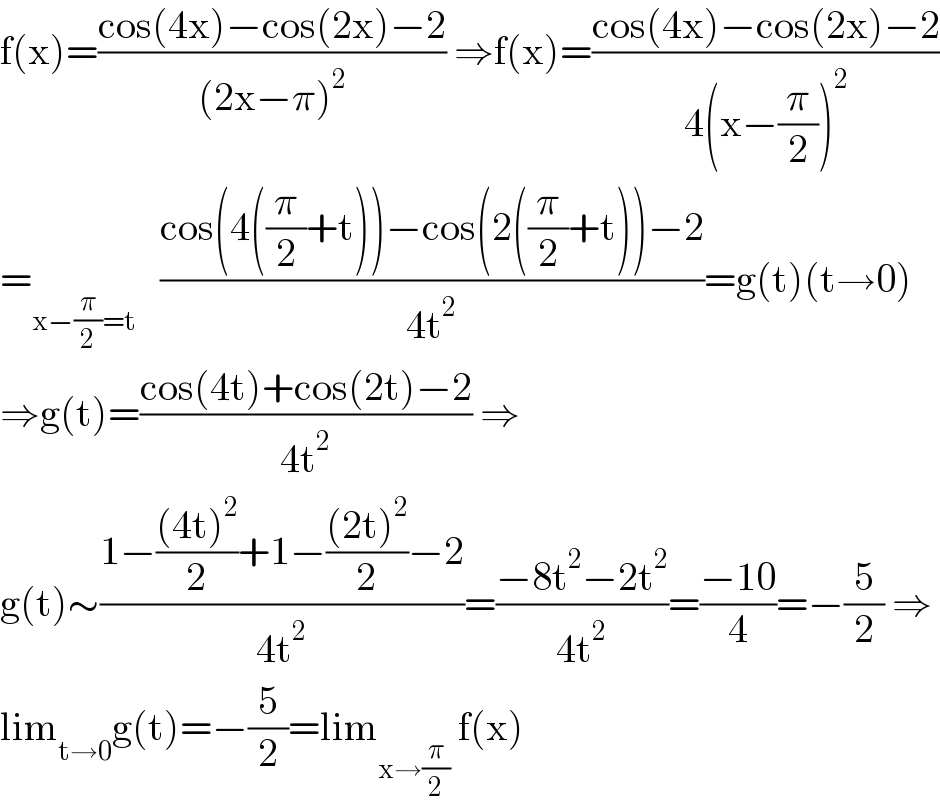
Commented by liberty last updated on 18/Jul/21

(4) \lim_{x \to 0} \frac{12 - 6 x^{2} - 12 (1 - \frac{x^{2}}{2} + \frac{x^{4}}{24})}{x^{4}} =

\lim_{x \to 0} \frac{12 - 6 x^{2} - 12 + 6 x^{2} - \frac{1}{2} x^{4}}{x^{4}} =

\lim_{x \to 0} \frac{- \frac{1}{2} x^{4}}{x^{4}} = - \frac{1}{2}

B y L^{'} H o p i t a l

\lim_{x \to 0} \frac{12 - 6 x^{2} - 12 \cos x}{x^{4}} =

\lim_{x \to 0} \frac{- 12 x + 12 \sin x}{4 x^{3}} =

= - 3 \times \lim_{x \to 0} \frac{x - \sin x}{x^{3}}

= - 3 \times \lim_{x \to 0} \frac{1 - \cos x}{3 x^{2}}

= - \times \lim_{x \to 0} \frac{2 \sin^{2} \frac{1}{2} x}{x^{2}} = - 2 \times \frac{1}{4}

= - \frac{1}{2} .

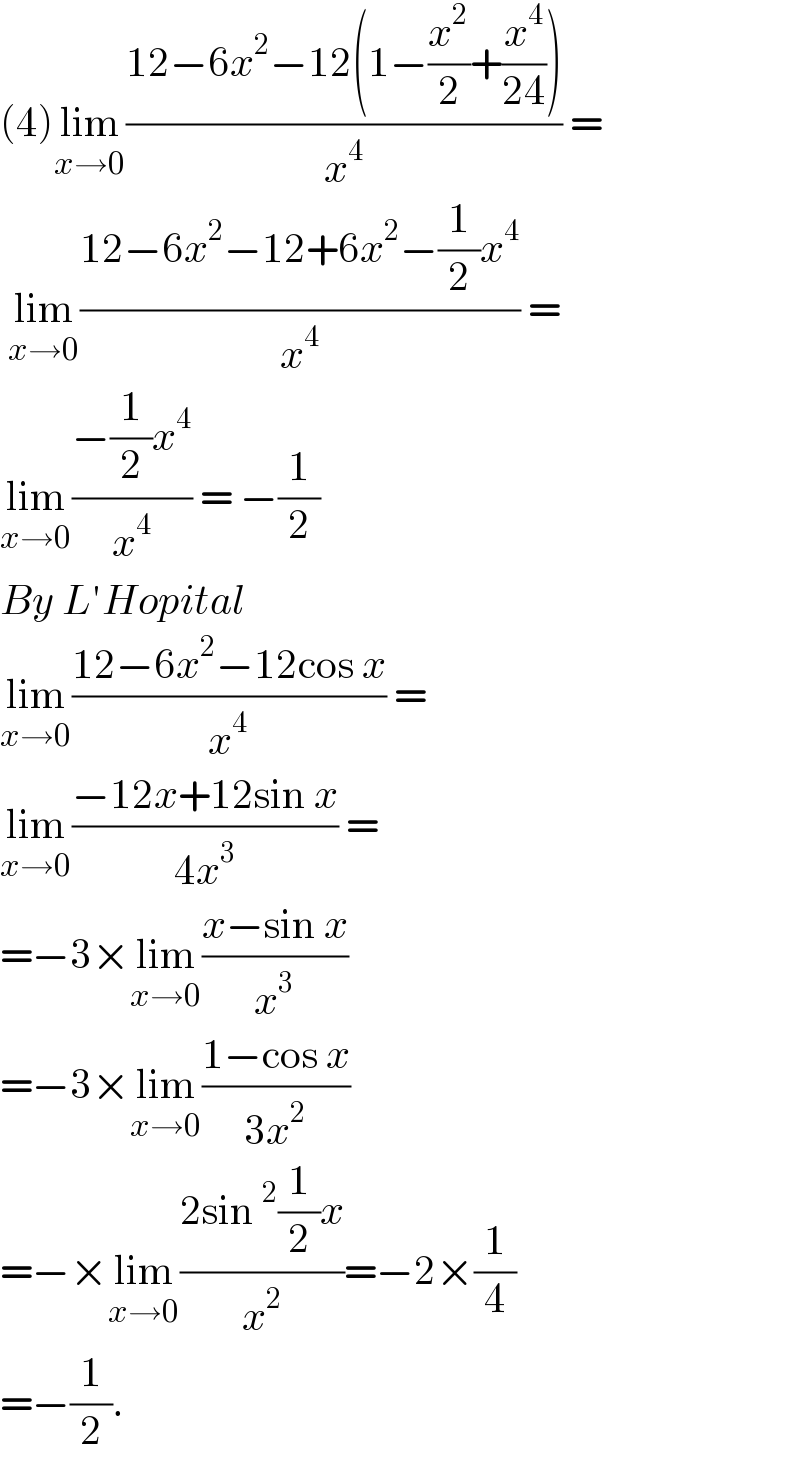
Answered by EDWIN88 last updated on 18/Jul/21

(1) \lim_{x \to \frac{π}{2}} \frac{\cos 4 x - \cos 2 x - 2}{{(2 x - π)}^{2}} = ?

sol : let x - \frac{π}{2} = t; x = \frac{π}{2} + t

\lim_{t \to 0} \frac{\cos (2 π + 4 t) - \cos (π + 2 t) - 2}{{4 t}^{2}}

= \lim_{t \to 0} \frac{\cos 4 t + \cos 2 t - 2}{{4 t}^{2}}

= \lim_{t \to 0} \frac{(\cos 4 t - 1) + (\cos 2 t - 1)}{{4 t}^{2}}

= \lim_{t \to 0} \frac{- 2 \sin^{2} 2 t - 2 \sin^{2} t}{{4 t}^{2}}

= - \frac{1}{2} [\lim_{t \to 0} {(\frac{\sin 2 t}{t})}^{2} + \lim_{t \to 0} {(\frac{\sin t}{t})}^{2}]

= - \frac{1}{2} (2^{2} + 1^{2}) = - \frac{5}{2}

Answered by EDWIN88 last updated on 18/Jul/21

(2) \lim_{x \to 0} \frac{\sin 3 x + \sin 6 x - \sin 9 x}{x^{3}}

= \lim_{x \to 0} \frac{2 \sin (\frac{9 x}{2}) \cos (\frac{3 x}{2}) - 2 \sin (\frac{9 x}{2}) \cos (\frac{9 x}{2})}{x^{3}}

let \frac{3 x}{2} = u; u \to 0, \frac{9 x}{2} = 3 u \land x = \frac{2 u}{3}

= \frac{27}{8} \lim_{u \to 0} \frac{2 \sin 3 u \cos u - 2 \sin 3 u \cos 3 u}{u^{3}}

= \frac{27}{8} \lim_{u \to 0} \frac{2 \sin 3 u (\cos u - \cos 3 u)}{u^{3}}

= \frac{27}{8} {\lim_{u \to 0} \frac{2 \sin 3 u}{u} \times \lim_{u \to 0} \frac{\cos u - \cos 3 u}{u^{2}}}

= \frac{27}{8} {6 \times \lim_{u \to 0} \frac{2 \sin 2 u \sin u}{u^{2}}}

= \frac{27}{8} {6 \times 4} = \frac{27}{8} \times 24 = 81

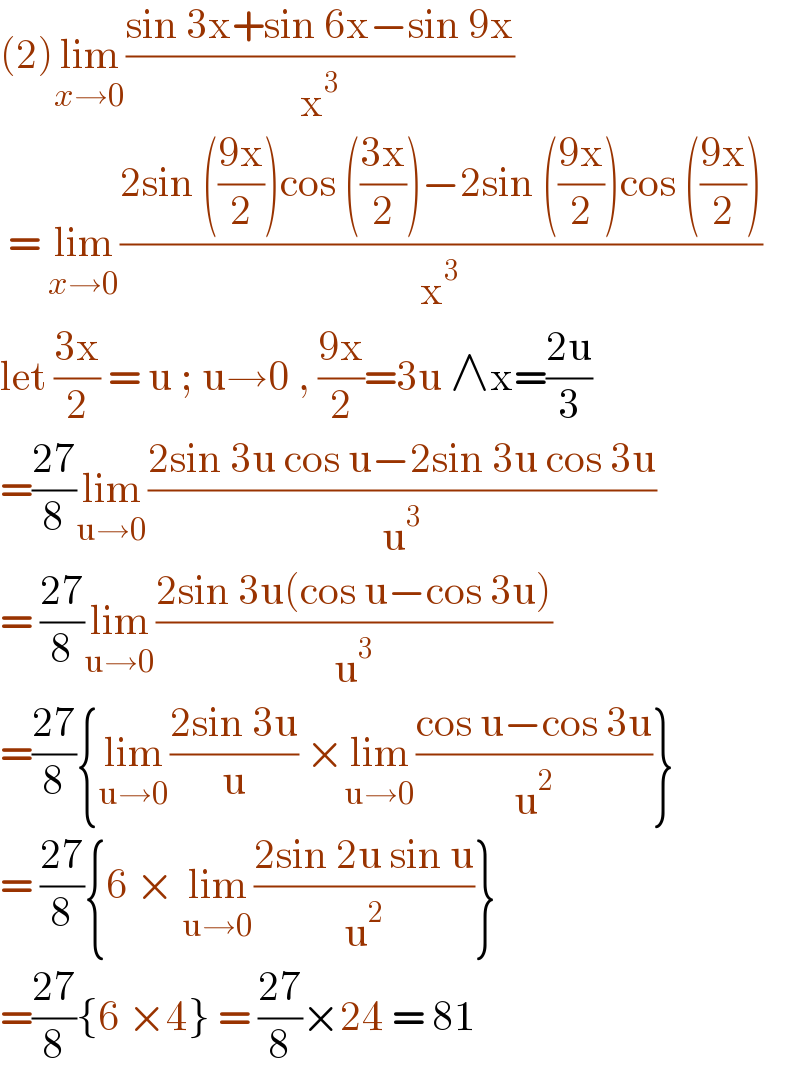
Answered by liberty last updated on 18/Jul/21

(3) \lim_{x \to \frac{π}{4}} \frac{\sec^{2} x - 2 \tan x}{{(x - \frac{π}{4})}^{2}}

= \lim_{x \to \frac{π}{4}} \frac{\tan^{2} x - 2 \tan x + 1}{{(x - \frac{π}{4})}^{2}}

set x - \frac{π}{4} = q; q \to 0 \land x = q + \frac{π}{4}

\lim_{q \to 0} \frac{{(\tan (q + \frac{π}{4}) - 1)}^{2}}{q^{2}}

= \lim_{q \to 0} {(\frac{\frac{\tan q + 1}{1 - \tan q} - 1}{q})}^{2}

= \lim_{q \to 0} {(\frac{2 \tan q}{(1 - \tan q) q})}^{2}

= \lim_{q \to 0} {(\frac{1}{1 - \tan q})}^{2} \times \lim_{q \to 0} {(\frac{2 \tan q}{q})}^{2}

= 1 \times {(2)}^{2} = 4

Facebook Tweet Pin