Question Number 101345 by bobhans last updated on 02/Jul/20
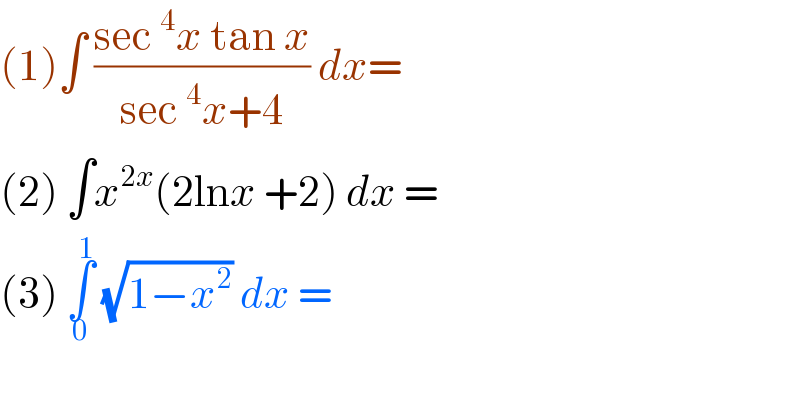
$$\left(\mathrm{1}\right)\int\:\frac{\mathrm{sec}\:^{\mathrm{4}} {x}\:\mathrm{tan}\:{x}}{\mathrm{sec}\:^{\mathrm{4}} {x}+\mathrm{4}}\:{dx}= \\ $$$$\left(\mathrm{2}\right)\:\int{x}^{\mathrm{2}{x}} \left(\mathrm{2ln}{x}\:+\mathrm{2}\right)\:{dx}\:= \\ $$$$\left(\mathrm{3}\right)\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\:{dx}\:=\: \\ $$
Commented by john santu last updated on 02/Jul/20
![(1)∫ ((sec^4 x tan x)/(sec^4 x +4)) dx set : z = sec^4 x + 4 → sec^4 x tan x dx =(1/4) dz I = (1/4)∫ (dz/z) = (1/4) ln ∣z∣ + c = (1/4) ln ∣sec^4 x + 4∣ + c (2) ∫ x^(2x) (2 ln x +2) dx apply a^b = e^(b ln a) J = ∫ e^(2x ln (x) ) (2ln x +2) dx let q = 2x ln x → dq = (2ln x +2)dx J= ∫ e^q dq = e^q + c = x^(2x) + c (3) ∫_0 ^1 (√(1−x^2 )) dx = (π/4) long way : set: sin s = x G = ∫_0 ^(π/2) (√(1−sin^2 s)) cos s ds G= ∫_0 ^(π/2) [ (1/2) + (1/2) cos (2s)] ds = [(1/2)s + (1/4) sin (2s) ]_0 ^(π/2) = (π/4) ♠♥⧫](https://www.tinkutara.com/question/Q101348.png)
$$\left(\mathrm{1}\right)\int\:\frac{\mathrm{sec}\:^{\mathrm{4}} {x}\:\mathrm{tan}\:{x}}{\mathrm{sec}\:^{\mathrm{4}} {x}\:+\mathrm{4}}\:{dx}\: \\ $$$${set}\::\:\mathrm{z}\:=\:\mathrm{sec}\:^{\mathrm{4}} \mathrm{x}\:+\:\mathrm{4}\:\rightarrow\:\mathrm{sec}\:^{\mathrm{4}} \mathrm{x}\:\mathrm{tan}\:\mathrm{x}\:\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{dz} \\ $$$$\mathrm{I}\:=\:\frac{\mathrm{1}}{\mathrm{4}}\int\:\frac{\mathrm{dz}}{\mathrm{z}}\:=\:\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{ln}\:\mid\mathrm{z}\mid\:+\:\mathrm{c}\:\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{ln}\:\mid\mathrm{sec}\:^{\mathrm{4}} \mathrm{x}\:+\:\mathrm{4}\mid\:+\:\mathrm{c}\: \\ $$$$\left(\mathrm{2}\right)\:\int\:{x}^{\mathrm{2}{x}} \:\left(\mathrm{2}\:\mathrm{ln}\:{x}\:+\mathrm{2}\right)\:{dx}\: \\ $$$${apply}\:{a}^{{b}} \:=\:{e}^{{b}\:\mathrm{ln}\:{a}} \\ $$$$\mathrm{J}\:=\:\int\:\mathrm{e}^{\mathrm{2}{x}\:\mathrm{ln}\:\left({x}\right)\:} \left(\mathrm{2ln}\:{x}\:+\mathrm{2}\right)\:{dx}\: \\ $$$${let}\:{q}\:=\:\mathrm{2}{x}\:\mathrm{ln}\:{x}\:\rightarrow\:{dq}\:=\:\left(\mathrm{2ln}\:{x}\:+\mathrm{2}\right){dx} \\ $$$${J}=\:\int\:\mathrm{e}^{{q}} \:{dq}\:=\:{e}^{{q}} \:+\:{c}\:=\:{x}^{\mathrm{2}{x}} \:+\:{c}\: \\ $$$$\left(\mathrm{3}\right)\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\mathrm{dx}\:=\:\frac{\pi}{\mathrm{4}}\: \\ $$$$\mathrm{long}\:\mathrm{way}\::\:\mathrm{set}:\:\mathrm{sin}\:\mathrm{s}\:=\:\:{x}\: \\ $$$$\mathrm{G}\:=\:\underset{\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\sqrt{\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{s}}\:\mathrm{cos}\:\mathrm{s}\:\mathrm{ds}\: \\ $$$$\mathrm{G}=\:\underset{\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\left[\:\frac{\mathrm{1}}{\mathrm{2}}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\:\mathrm{cos}\:\left(\mathrm{2s}\right)\right]\:\mathrm{ds}\: \\ $$$$=\:\left[\frac{\mathrm{1}}{\mathrm{2}}\mathrm{s}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{sin}\:\left(\mathrm{2s}\right)\:\right]_{\mathrm{0}} ^{\pi/\mathrm{2}} =\:\frac{\pi}{\mathrm{4}}\: \\ $$$$\spadesuit\heartsuit\blacklozenge \\ $$
Commented by bramlex last updated on 02/Jul/20

$$\heartsuit\blacklozenge\spadesuit \\ $$
Commented by bobhans last updated on 02/Jul/20

$$\mathrm{thank}\:\mathrm{you} \\ $$
