1-Show-that-0-pi-xdx-a-2-sin-2-x-b-2-cos-2-x-2-pi-2-a-2-b-2-4a-3-b-3-2-The-density-at-the-point-x-y-of-a-lamina-bounded-by-the-circle-x-2-y-2-2ax-0-i

Tinku Tara June 4, 2023 None 0 Comments

Facebook Tweet Pin

Question Number 94992 by Mr.D.N. last updated on 22/May/20

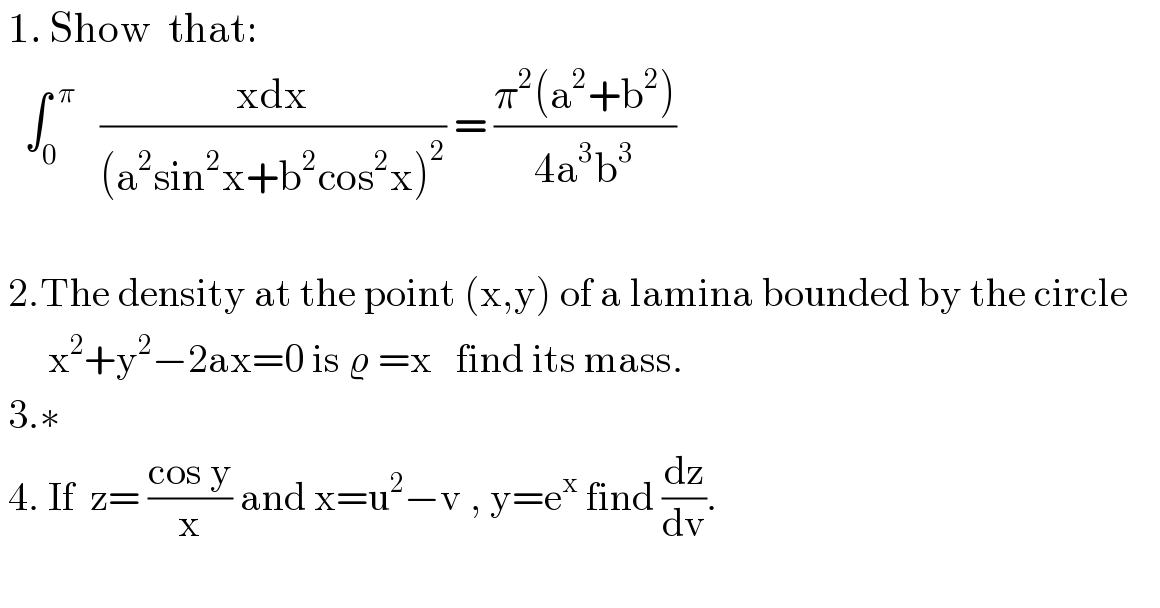
1. Show that: ∫_0 ^( π) ((xdx)/((a^2 sin^2 x+b^2 cos^2 x)^2 )) = ((π^2 (a^2 +b^2 ))/(4a^3 b^3 )) 2.The density at the point (x,y) of a lamina bounded by the circle x^2 +y^2 −2ax=0 is ϱ =x find its mass. 3.∗ 4. If z= ((cos y)/x) and x=u^2 −v , y=e^x find (dz/dv).

1 . Show that :

\int_{0}^{π} \frac{xdx}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} = \frac{π^{2} (a^{2} + b^{2})}{{4 a}^{3} b^{3}}

2 . The density at the point (x, y) of a lamina bounded by the circle

x^{2} + y^{2} - 2 ax = 0 is ϱ = x find its mass .

3 . *

4 . If z = \frac{\cos y}{x} and x = u^{2} - v, y = e^{x} find \frac{dz}{dv} .

Answered by bobhans last updated on 22/May/20

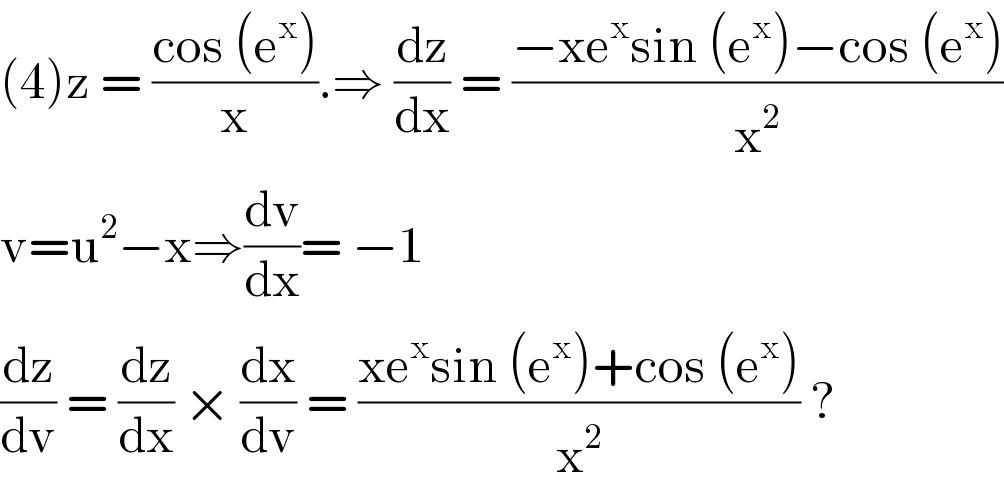
(4)z = ((cos (e^x ))/x).⇒ (dz/dx) = ((−xe^x sin (e^x )−cos (e^x ))/x^2 ) v=u^2 −x⇒(dv/dx)= −1 (dz/dv) = (dz/dx) × (dx/dv) = ((xe^x sin (e^x )+cos (e^x ))/x^2 ) ?

(4) z = \frac{\cos (e^{x})}{x} . \Rightarrow \frac{dz}{dx} = \frac{- {xe}^{x} \sin (e^{x}) - \cos (e^{x})}{x^{2}}

v = u^{2} - x \Rightarrow \frac{dv}{dx} = - 1

\frac{dz}{dv} = \frac{dz}{dx} \times \frac{dx}{dv} = \frac{{xe}^{x} \sin (e^{x}) + \cos (e^{x})}{x^{2}} ?

Commented by Mr.D.N. last updated on 22/May/20

thank you nice solution��

Answered by Ar Brandon last updated on 22/May/20

1\I=∫_0 ^π ((xdx)/((a^2 sin^2 x+b^2 cos^2 x)^2 )) x=π−u⇒dx=−du⇒I=∫_0 ^π (π/((a^2 sin^2 u+b^2 cos^2 u)^2 ))du−I ⇒2I=∫_0 ^π (π/((a^2 sin^2 x+b^2 cos^2 x)^2 ))dx=π∫_0 ^π ((sec^4 x)/((a^2 tan^2 x+b^2 )^2 ))dx ⇒2I=_(t=tanx) π∫_(+0) ^(−0) ((t^2 +1)/((a^2 t^2 +b^2 )^2 ))dt=π∫_(+0) ^(−0) [((1/a^2 )/(a^2 t^2 +b^2 ))+(((a^2 −b^2 )/a^2 )/((a^2 t^2 +b^2 )^2 ))]dt 2I=(π/(a^2 b^2 ))∫_(+0) ^(−0) (1/(((a/b)t)^2 +1))dt+((π(a^2 −b^2 ))/(a^2 b^4 ))∫_(+0) ^(−0) (1/([((a/b)t)^2 +1]^2 ))dt 2I=(π/(a^3 b))[tan^(−1) ((a/b)t)]_(+0) ^(−0) +((π(a^2 −b^2 ))/(a^2 b^4 ))∫_0 ^π (((b/a)sec^2 θ)/(sec^4 θ))dθ =(π^2 /(a^3 b))+((π(a^2 −b^2 ))/(2a^3 b^3 ))∫_0 ^π (1+cos 2θ)dθ=(π^2 /(a^3 b))+((π(a^2 −b^2 ))/(2a^3 b^3 ))[θ+((sin2θ)/2)]_0 ^π =(π^2 /(a^3 b))+((π^2 (a^2 −b^2 ))/(2a^3 b^3 ))=((2π^2 b^2 +a^2 π^2 −π^2 b^2 )/(2a^3 b^3 ))=((π^2 (a^2 +b^2 ))/(2a^3 b^3 )) ⇒∫_0 ^π ((xdx)/((a^2 sin^2 x+b^2 cos^2 x)^2 ))=((π^2 (a^2 +b^2 ))/(4a^3 b^3 ))

1 ∖ I = \int_{0}^{π} \frac{xdx}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}}

x = π - u \Rightarrow dx = - du \Rightarrow I = \int_{0}^{π} \frac{π}{{(a^{2} \sin^{2} u + b^{2} \cos^{2} u)}^{2}} du - I

\Rightarrow 2 I = \int_{0}^{π} \frac{π}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} dx = π \int_{0}^{π} \frac{\sec^{4} x}{{(a^{2} \tan^{2} x + b^{2})}^{2}} dx

\Rightarrow 2 I \underset{t = tanx}{=} π \int_{+ 0}^{- 0} \frac{t^{2} + 1}{{(a^{2} t^{2} + b^{2})}^{2}} dt = π \int_{+ 0}^{- 0} [\frac{1 / a^{2}}{a^{2} t^{2} + b^{2}} + \frac{(a^{2} - b^{2}) / a^{2}}{{(a^{2} t^{2} + b^{2})}^{2}}] dt

2 I = \frac{π}{a^{2} b^{2}} \int_{+ 0}^{- 0} \frac{1}{{(\frac{a}{b} t)}^{2} + 1} dt + \frac{π (a^{2} - b^{2})}{a^{2} b^{4}} \int_{+ 0}^{- 0} \frac{1}{{[{(\frac{a}{b} t)}^{2} + 1]}^{2}} dt

2 I = \frac{π}{a^{3} b} {[\tan^{- 1} (\frac{a}{b} t)]}_{+ 0}^{- 0} + \frac{π (a^{2} - b^{2})}{a^{2} b^{4}} \int_{0}^{π} \frac{(b / a) \sec^{2} θ}{\sec^{4} θ} d θ

= \frac{π^{2}}{a^{3} b} + \frac{π (a^{2} - b^{2})}{{2 a}^{3} b^{3}} \int_{0}^{π} (1 + \cos 2 θ) d θ = \frac{π^{2}}{a^{3} b} + \frac{π (a^{2} - b^{2})}{{2 a}^{3} b^{3}} {[θ + \frac{\sin 2 θ}{2}]}_{0}^{π}

= \frac{π^{2}}{a^{3} b} + \frac{π^{2} (a^{2} - b^{2})}{{2 a}^{3} b^{3}} = \frac{2 π^{2} b^{2} + a^{2} π^{2} - π^{2} b^{2}}{{2 a}^{3} b^{3}} = \frac{π^{2} (a^{2} + b^{2})}{{2 a}^{3} b^{3}}

\Rightarrow \int_{0}^{π} \frac{xdx}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} = \frac{π^{2} (a^{2} + b^{2})}{{4 a}^{3} b^{3}}

Commented by Mr.D.N. last updated on 22/May/20

lim is all[right you might be mistek some way]

l i m i s a l l [r i g h t y o u m i g h t b e m i s t e k s o m e w a y]

Commented by Ar Brandon last updated on 22/May/20

Okay let's check my solution and see if I might have made some mistakes.

Commented by niroj last updated on 22/May/20

let me solve then you find your correction just taken few time then you find easily where is a error....��

Commented by Ar Brandon last updated on 22/May/20

Okay, I'm grateful. Thanks

Commented by Ar Brandon last updated on 22/May/20

Hey hold on. I wanna give it a last try.��

Commented by Ar Brandon last updated on 22/May/20

Thanks for your patience Mr. Niroj ��

Commented by Ar Brandon last updated on 22/May/20

I found my mistake.�� It was necessary for me to respect the signs. That is, distinguish between –0 and +0��

Answered by niroj last updated on 22/May/20

1. I= ∫_0 ^( π) (( xdx)/((a^2 sin^2 x+b^2 cos^2 x)^2 )) .....(i) = ∫_0 ^( π) (((π−x)dx)/({a^2 sin^2 (π−a)+b^2 cos^2 (π−a)}^2 )) I = ∫_0 ^( π) (((π−x)dx)/((a^2 sin^2 x+b^2 cos^2 x)^2 ))....(ii) Added (i)+(ii) 2I= ∫_0 ^π (π/((a^2 sin^2 x+b^2 cos^2 x)^2 ))dx I= (π/2)×2∫_0 ^( (π/2)) (( 1)/((a^2 sin^2 x+b^2 cos^2 x)^2 ))dx = π ∫_0 ^( (π/2)) (( 1)/((a^2 sin^2 x+b^2 cos^2 x)^2 ))dx Divide numerator& denominator by (1/(cos^4 x)) = π∫_0 ^( (π/2)) (( sec^4 xdx)/({(1/(cos^2 x))(a^2 sin^2 x+b^2 cos^2 x)}^2 )) = π ∫_0 ^( (π/2)) (((tan^2 x+1) sec^2 x dx)/((a^2 tan^2 x+b^2 )^2 )) Put tan x = t sec^2 xdx=dt If x=(π/2) ⇒ t=∞ If x=0 ⇒ t=0 Now, π∫_0 ^( ∞) (((t^2 +1)dt)/((a^2 t^2 +b^2 )^2 )) = (π/a^2 )∫_0 ^( ∞) ((a^2 t^2 +a^2 )/((a^2 t^2 +b^2 )^2 ))dt = (π/a^2 ) ∫_(0 ) ^( ∞) (( (a^2 −b^2 )+(a^2 t^2 +b^2 ))/((a^2 t^2 +b^2 )^2 ))dt = (π/a^2 )∫_0 ^( ∞) (((a^2 −b^2 ))/((a^2 t^2 +b^2 )^2 ))dt+ (π/a^2 )∫_0 ^∞ (1/(a^2 t^2 +b^2 ))dt = ((π(a^2 −b^2 ))/a^2 )∫_0 ^( ∞) (1/((a^2 t^2 +b^2 )^2 ))dt+(π/(a^2 .a^2 ))∫_0 ^∞ (1/((t)^2 +((b/a))^2 ))dt here, I= I_1 +I_2 I_1 = ((π(a^2 −b^2 ))/a^2 )∫_0 ^( ∞) (1/((a^2 t^2 +b^2 )^2 ))dt Put, at= b tanθ adt= b sec^2 θdθ dt= (b/a) sec^2 θdθ If t=∞ ⇒ θ= (π/2) If t=0 ⇒ θ=0 ((π(a^2 −b^2 ))/a^2 )(b/a)∫_0 ^( (π/2)) ((sec^2 θdθ)/((b^2 tan^2 θ+b^2 )^2 )) = ((π(a^2 −b^2 )b)/a^3 )∫_0 ^(π/2) ((sec^2 θdθ)/(b^4 .sec^4 θ)) = ((π(a^2 −b^2 ))/(a^3 b^3 ))∫_0 ^(π/2) cos^2 θdθ cos2θ= 1−2cos^2 θ⇒ cos^2 θ =((1−cos2θ)/2) [ (1/2)∫(1−cos2θ)dθ]_0 ^(π/2) = [ (1/2)(θ − ((sin2θ)/2))]_0 ^(π/2) ⇒ [(1/2)((π/2)−((sin2×(π/2))/2))−0] = (π/4) I_1 = ((π(a^2 −b^2 ))/(a^3 b^3 ))×(π/4) Again for I_2 = (π/a^4 )[ (1/(b/a)) tan^(−1) (t/(b/a))]_0 ^∞ = (π/a^4 )[ (a/b)tan^(−1) ((at)/b)]_0 ^∞ = (π/a^4 )[ (a/b)×(π/2)−0] = (π^2 /(2a^3 b)) Now complete solution I= I_1 +I_2 = ((π^2 (a^2 −b^2 ))/(4a^3 b^3 ))+ (π^2 /(2a^3 b)) = (π^2 /(2a^3 b))(((a^2 −b^2 )/(2b^2 ))+1) = (π^2 /(2a^3 b))(((a^2 −b^2 +2b^2 )/(2b^2 ))) = ((π^2 (a^2 +b^2 ))/(4a^3 b^3 )) //. Which is required to proof.

1 . I = \int_{0}^{π} \frac{xdx}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} \dots . . (i)

= \int_{0}^{π} \frac{(π - x) dx}{{a^{2} \sin^{2} (π - a) + b^{2} \cos^{2} (π - a)}^{2}}

I = \int_{0}^{π} \frac{(π - x) dx}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} \dots . (ii)

Added (i) + (ii)

2 I = \int_{0}^{π} \frac{π}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} dx

I = \frac{π}{2} \times 2 \int_{0}^{\frac{π}{2}} \frac{1}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} dx

= π \int_{0}^{\frac{π}{2}} \frac{1}{{(a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}} dx

Divide numerator & denominator by \frac{1}{\cos^{4} x}

= π \int_{0}^{\frac{π}{2}} \frac{\sec^{4} xdx}{{\frac{1}{\cos^{2} x} (a^{2} \sin^{2} x + b^{2} \cos^{2} x)}^{2}}

= π \int_{0}^{\frac{π}{2}} \frac{(\tan^{2} x + 1) \sec^{2} x dx}{{(a^{2} \tan^{2} x + b^{2})}^{2}}

Put \tan x = t

\sec^{2} xdx = dt

If x = \frac{π}{2} \Rightarrow t = \infty

If x = 0 \Rightarrow t = 0

N o w,

π \int_{0}^{\infty} \frac{(t^{2} + 1) dt}{{(a^{2} t^{2} + b^{2})}^{2}}

= \frac{π}{a^{2}} \int_{0}^{\infty} \frac{a^{2} t^{2} + a^{2}}{{(a^{2} t^{2} + b^{2})}^{2}} dt

= \frac{π}{a^{2}} \int_{0}^{\infty} \frac{(a^{2} - b^{2}) + (a^{2} t^{2} + b^{2})}{{(a^{2} t^{2} + b^{2})}^{2}} dt

= \frac{π}{a^{2}} \int_{0}^{\infty} \frac{(a^{2} - b^{2})}{{(a^{2} t^{2} + b^{2})}^{2}} dt + \frac{π}{a^{2}} \int_{0}^{\infty} \frac{1}{a^{2} t^{2} + b^{2}} dt

= \frac{π (a^{2} - b^{2})}{a^{2}} \int_{0}^{\infty} \frac{1}{{(a^{2} t^{2} + b^{2})}^{2}} dt + \frac{π}{a^{2} . a^{2}} \int_{0}^{\infty} \frac{1}{{(t)}^{2} + {(\frac{b}{a})}^{2}} dt

here, I = I_{1} + I_{2}

I_{1} = \frac{π (a^{2} - b^{2})}{a^{2}} \int_{0}^{\infty} \frac{1}{{(a^{2} t^{2} + b^{2})}^{2}} dt

Put, at = b \tan θ

adt = b \sec^{2} θ d θ

dt = \frac{b}{a} \sec^{2} θ d θ

If t = \infty \Rightarrow θ = \frac{π}{2}

If t = 0 \Rightarrow θ = 0

\frac{π (a^{2} - b^{2})}{a^{2}} \frac{b}{a} \int_{0}^{\frac{π}{2}} \frac{\sec^{2} θ d θ}{{(b^{2} \tan^{2} θ + b^{2})}^{2}}

= \frac{π (a^{2} - b^{2}) b}{a^{3}} \int_{0}^{\frac{π}{2}} \frac{\sec^{2} θ d θ}{b^{4} . \sec^{4} θ}

= \frac{π (a^{2} - b^{2})}{a^{3} b^{3}} \int_{0}^{\frac{π}{2}} \cos^{2} θ d θ

\cos 2 θ = 1 - {2 \cos}^{2} θ \Rightarrow \cos^{2} θ = \frac{1 - \cos 2 θ}{2}

{[\frac{1}{2} \int (1 - \cos 2 θ) d θ]}_{0}^{\frac{π}{2}}

= {[\frac{1}{2} (θ - \frac{\sin 2 θ}{2})]}_{0}^{\frac{π}{2}} \Rightarrow [\frac{1}{2} (\frac{π}{2} - \frac{\sin 2 \times \frac{π}{2}}{2}) - 0]

= \frac{π}{4}

I_{1} = \frac{π (a^{2} - b^{2})}{a^{3} b^{3}} \times \frac{π}{4}

Again for

I_{2} = \frac{π}{a^{4}} {[\frac{1}{\frac{b}{a}} \tan^{- 1} \frac{t}{\frac{b}{a}}]}_{0}^{\infty}

= \frac{π}{a^{4}} {[\frac{a}{b} \tan^{- 1} \frac{at}{b}]}_{0}^{\infty}

= \frac{π}{a^{4}} [\frac{a}{b} \times \frac{π}{2} - 0]

= \frac{π^{2}}{{2 a}^{3} b}

N o w complete solution

I = I_{1} + I_{2}

= \frac{π^{2} (a^{2} - b^{2})}{{4 a}^{3} b^{3}} + \frac{π^{2}}{{2 a}^{3} b}

= \frac{π^{2}}{{2 a}^{3} b} (\frac{a^{2} - b^{2}}{{2 b}^{2}} + 1)

= \frac{π^{2}}{{2 a}^{3} b} (\frac{a^{2} - b^{2} + {2 b}^{2}}{{2 b}^{2}})

= \frac{π^{2} (a^{2} + b^{2})}{{4 a}^{3} b^{3}} / / .

Which is required to proof .

Commented by Mr.D.N. last updated on 22/May/20

Thanks for all of you to presents your ability it's really I appreciate.. ��

Answered by niroj last updated on 22/May/20

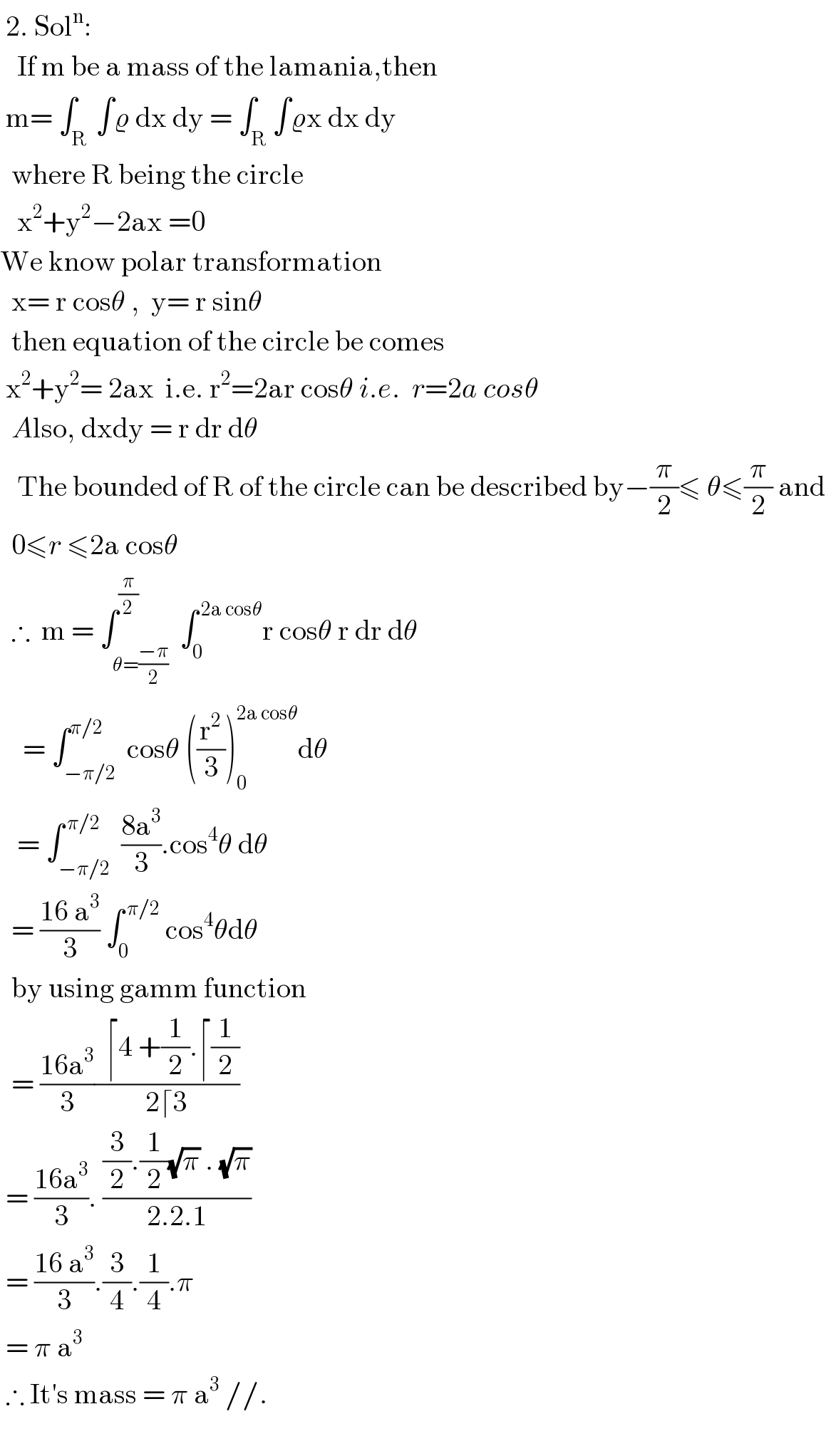
2. Sol^n : If m be a mass of the lamania,then m= ∫_(R ) ∫ϱ dx dy = ∫_R ∫ϱx dx dy where R being the circle x^2 +y^2 −2ax =0 We know polar transformation x= r cosθ , y= r sinθ then equation of the circle be comes x^2 +y^2 = 2ax i.e. r^2 =2ar cosθ i.e. r=2a cosθ Also, dxdy = r dr dθ The bounded of R of the circle can be described by−(π/2)≤ θ≤(π/2) and 0≤r ≤2a cosθ ∴ m = ∫_(θ=((−π)/2)) ^(π/2) ∫_0 ^( 2a cosθ) r cosθ r dr dθ = ∫_(−π/2) ^(π/2) cosθ ((r^2 /3))_0 ^(2a cosθ) dθ = ∫_(−π/2) ^( π/2) ((8a^3 )/3).cos^4 θ dθ = ((16 a^3 )/3) ∫_0 ^( π/2) cos^4 θdθ by using gamm function = ((16a^3 )/3)(( ⌈4 +(1/2).⌈(1/2))/(2⌈3)) = ((16a^3 )/3). (((3/2).(1/2)(√π) . (√π))/(2.2.1)) = ((16 a^3 )/3).(3/4).(1/4).π = π a^3 ∴ It′s mass = π a^3 //.

2 . {Sol}^{n} :

If m be a mass of the lamania, then

m = \int_{R} \int ϱ dx dy = \int_{R} \int ϱ x dx dy

where R being the circle

x^{2} + y^{2} - 2 ax = 0

We know polar transformation

x = r \cos θ, y = r \sin θ

then equation of the circle be comes

x^{2} + y^{2} = 2 ax i . e . r^{2} = 2 ar \cos θ i . e . r = 2 a c o s θ

A lso, dxdy = r dr d θ

The bounded of R of the circle can be described by - \frac{π}{2} ⩽ θ ⩽ \frac{π}{2} and

0 ⩽ r ⩽ 2 a \cos θ

∴ m = \int_{θ = \frac{- π}{2}}^{\frac{π}{2}} \int_{0}^{2 a \cos θ} r \cos θ r dr d θ

= \int_{- π / 2}^{π / 2} \cos θ {(\frac{r^{2}}{3})}_{0}^{2 a \cos θ} d θ

= \int_{- π / 2}^{π / 2} \frac{{8 a}^{3}}{3} . \cos^{4} θ d θ

= \frac{16 a^{3}}{3} \int_{0}^{π / 2} \cos^{4} θ d θ

by using gamm function

= \frac{{16 a}^{3}}{3} \frac{⌈ 4 + \frac{1}{2} . ⌈ \frac{1}{2}}{2 ⌈ 3}

= \frac{{16 a}^{3}}{3} . \frac{\frac{3}{2} . \frac{1}{2} \sqrt{π} . \sqrt{π}}{2.2.1}

= \frac{16 a^{3}}{3} . \frac{3}{4} . \frac{1}{4} . π

= π a^{3}

∴ {It}^{'} s mass = π a^{3} / / .

Commented by Mr.D.N. last updated on 22/May/20

what nice outstanding��

Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Facebook Tweet Pin

Leave a Reply Cancel reply