1-x-2-2-x-4-8x-2-4-dx-2-Shortest-distance-between-the-parabolas-y-2-4x-and-y-2-2x-6-is-

Question Number 58478 by rahul 19 last updated on 23/Apr/19

{1} \int \frac{x^{2} - 2}{x^{4} + 8 x^{2} + 4} d x = ?

{2} S h o r t e s t d i s t a n c e b e t w e e n t h e

p a r a b o l a s y^{2} = 4 x a n d y^{2} = 2 x - 6 i s ?

Commented by maxmathsup by imad last updated on 24/Apr/19

l e t A = \int \frac{x^{2} - 2}{x^{4} + 8 x^{2} + 4} d x r o o t s o f x^{4} + 8 x^{2} + 4 = 0 \Rightarrow t^{2} + 8 t + 4 = 0 w i t h t = x^{2}

Δ^{^{'}} = 4^{2} - 4 = 12 \Rightarrow t_{1} = - 4 + 2 \sqrt{3} a n d t_{2} = - 4 - 2 \sqrt{3} \Rightarrow

\frac{x^{2} - 2}{x^{4} + 8 x^{2} + 4} = \frac{t - 2}{(t - t_{1}) (t - t_{2})} = F (t) = \frac{a}{t - t_{1}} + \frac{b}{t - t_{2}}

a = {l i m}_{t \to t_{1}} (t - t_{1}) F (t) = \frac{t_{1} - 2}{t_{1} - t_{2}} = \frac{- 6 + 2 \sqrt{3}}{4 \sqrt{3}} = \frac{- 3 + \sqrt{3}}{2 \sqrt{3}} = \frac{- \sqrt{3} + 1}{2}

b = {l i m}_{t \to t_{2}} (t - t_{2}) F (t) = \frac{t_{2} - 2}{t_{2} - t_{1}} = \frac{- 6 - 2 \sqrt{3}}{- 4 \sqrt{3}} = \frac{3 + \sqrt{3}}{2 \sqrt{3}} = \frac{\sqrt{3} + 1}{2} \Rightarrow

A = \frac{1 - \sqrt{3}}{2} \int \frac{d x}{x^{2} - t_{1}} + \frac{1 + \sqrt{3}}{2} \int \frac{d x}{x^{2} - t_{2}} b u t

\int \frac{d x}{x^{2} - t_{1}} = \int \frac{d x}{x^{2} + 4 - 2 \sqrt{3}} d x = \int \frac{d x}{x^{2} + {(1 - \sqrt{3})}^{2}} =_{x = (1 - \sqrt{3}) u} \int \frac{(1 - \sqrt{3}) d u}{{(1 - \sqrt{3})}^{2} (1 + u^{2})}

= \frac{1}{1 - \sqrt{3}} a r c t a n (u) + c_{1} = \frac{1}{1 - \sqrt{3}} a r c t a n (\frac{x}{(1 - \sqrt{3})}) a l s o

\int \frac{d x}{x^{2} - t_{2}} = \int \frac{d x}{x^{2} + 4 + 2 \sqrt{3}} = \int \frac{d x}{x^{2} + {(1 + \sqrt{3})}^{2}} = \frac{1}{1 + \sqrt{3}} a r c t a n (\frac{x}{1 + \sqrt{3}}) + c_{2} \Rightarrow

A = \frac{1}{2} a r c t a n (\frac{x}{1 - \sqrt{3}}) + \frac{1}{2} a r c t a n (\frac{x}{1 + \sqrt{3}}) + C .

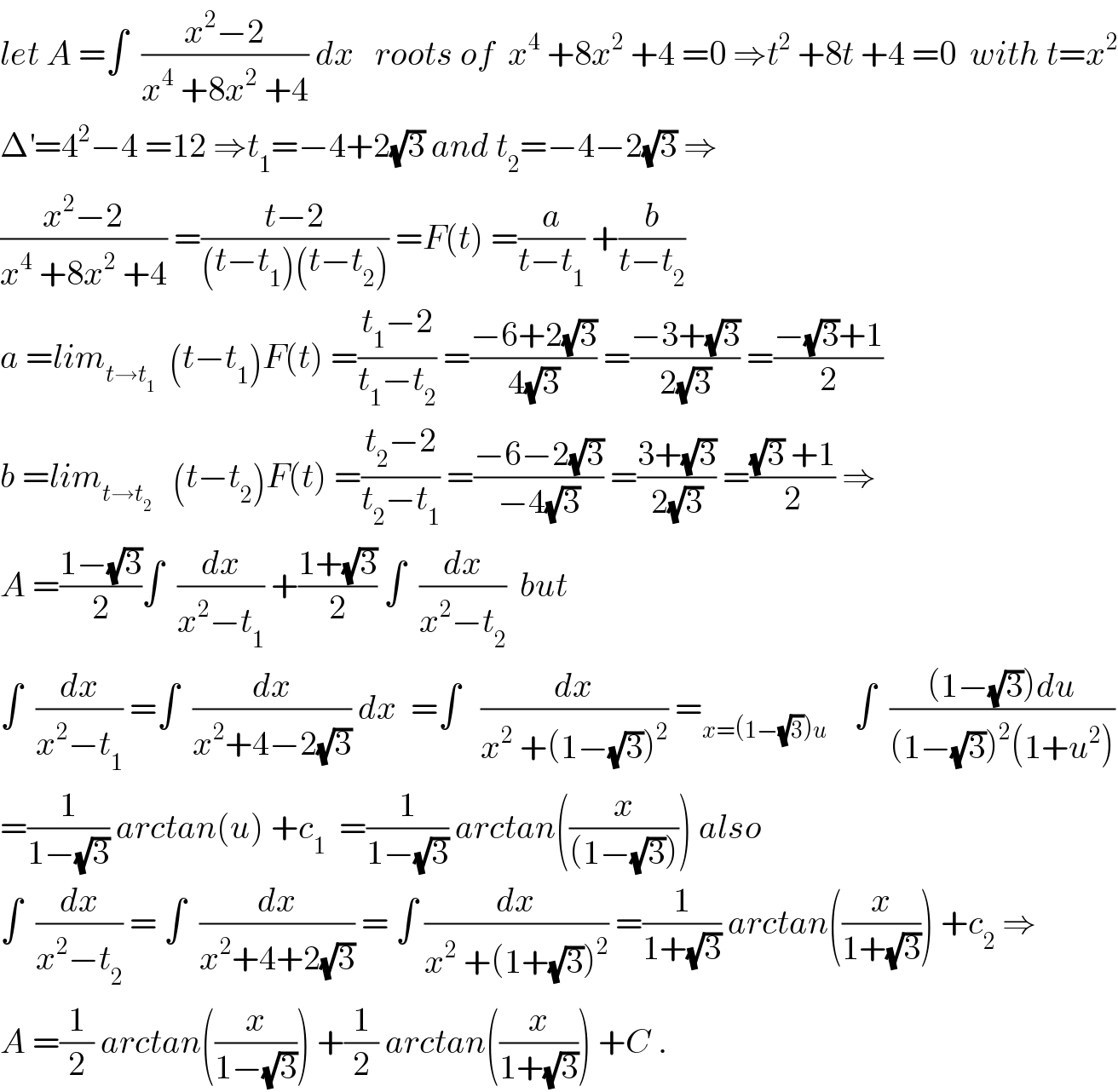
Answered by Prithwish sen last updated on 23/Apr/19

\int \frac{x^{2} - 2}{x^{4} + {8 x}^{2} + 4} dx

= \int \frac{(1 - \frac{2}{x^{2}})}{x^{2} + \frac{4}{x^{2}} + 8} dx

= \int \frac{d (x + \frac{2}{x})}{{(x + \frac{2}{x})}^{2} + 4}

= \frac{1}{2} \tan^{- 1} ∣ \frac{x^{2} + 2}{2 x} ∣ + C

Commented by rahul 19 last updated on 24/Apr/19

t h a n k u s i r .

Answered by mr W last updated on 23/Apr/19

(2)

f r o m p o i n t (\frac{h^{2}}{4}, h) t o p o i n t (\frac{k^{2} + 6}{2}, k) :

D = d^{2} = {(\frac{h^{2}}{4} - \frac{k^{2} + 6}{2})}^{2} + {(h - k)}^{2}

\frac{\partial D}{\partial h} = 2 (\frac{h^{2}}{4} - \frac{k^{2} + 6}{2}) \frac{2 h}{4} + 2 (h - k) = 0

\Rightarrow (\frac{h^{2}}{4} - \frac{k^{2} + 6}{2}) \frac{h}{2} + (h - k) = 0 \dots (i)

\frac{\partial D}{\partial k} = - 2 (\frac{h^{2}}{4} - \frac{k^{2} + 6}{2}) \frac{2 k}{2} - 2 (h - k) = 0

\Rightarrow (\frac{h^{2}}{4} - \frac{k^{2} + 6}{2}) k + (h - k) = 0 \dots (i i)

f r o m (i) a n d (i i) :

\Rightarrow k = \frac{h}{2}

\Rightarrow \frac{h^{2}}{8} = 2

\Rightarrow h = \pm 4 \Rightarrow p o i n t (4, \pm 4)

\Rightarrow k = \pm 2 \Rightarrow p o i n t (5, \pm 2)

m i n . d = \sqrt{D_{m i n}} = \sqrt{{(5 - 4)}^{2} + {(2 - 4)}^{2}} = \sqrt{5}

Commented by rahul 19 last updated on 24/Apr/19

t h a n k U s i r .