Question Number 168341 by Tibo last updated on 08/Apr/22

$$\int\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} {dx}=? \\ $$
Answered by Florian last updated on 08/Apr/22
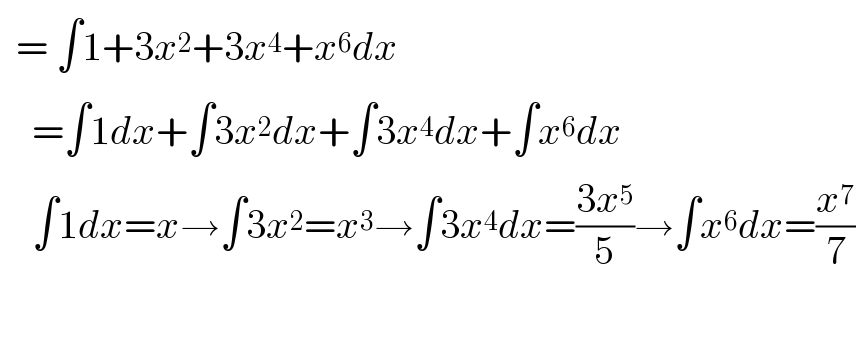
$$\:\:=\:\int\mathrm{1}+\mathrm{3}{x}^{\mathrm{2}} +\mathrm{3}{x}^{\mathrm{4}} +{x}^{\mathrm{6}} {dx} \\ $$$$\:\:\:\:=\int\mathrm{1}{dx}+\int\mathrm{3}{x}^{\mathrm{2}} {dx}+\int\mathrm{3}{x}^{\mathrm{4}} {dx}+\int{x}^{\mathrm{6}} {dx} \\ $$$$\:\:\:\:\int\mathrm{1}{dx}={x}\rightarrow\int\mathrm{3}{x}^{\mathrm{2}} ={x}^{\mathrm{3}} \rightarrow\int\mathrm{3}{x}^{\mathrm{4}} {dx}=\frac{\mathrm{3}{x}^{\mathrm{5}} }{\mathrm{5}}\rightarrow\int{x}^{\mathrm{6}} {dx}=\frac{{x}^{\mathrm{7}} }{\mathrm{7}} \\ $$$$\:\:\:\:\: \\ $$
Commented by Florian last updated on 08/Apr/22
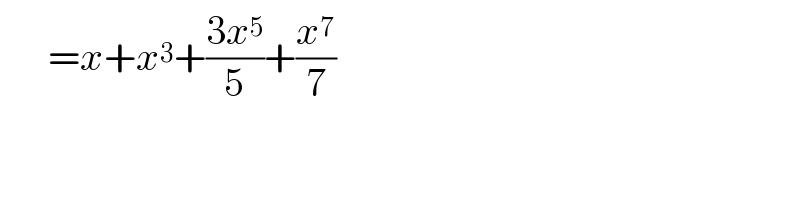
$$\:\:\:\:\:\:={x}+{x}^{\mathrm{3}} +\frac{\mathrm{3}{x}^{\mathrm{5}} }{\mathrm{5}}+\frac{{x}^{\mathrm{7}} }{\mathrm{7}} \\ $$
Commented by Tibo last updated on 08/Apr/22

$$\:\:{thank}\:{you}! \\ $$
