Question Number 106010 by bemath last updated on 02/Aug/20
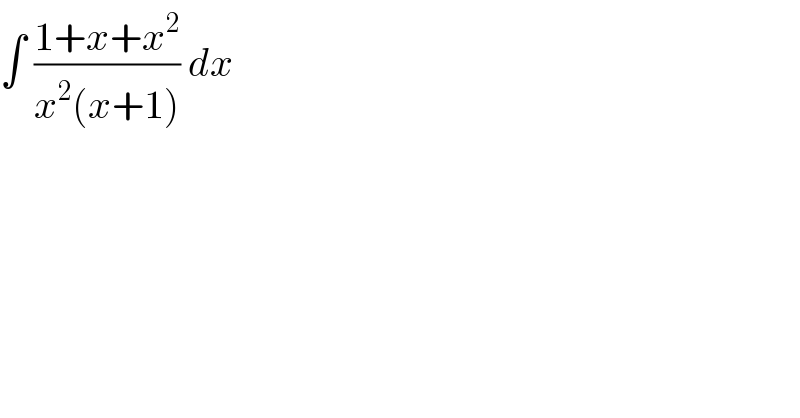
$$\int\:\frac{\mathrm{1}+{x}+{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} \left({x}+\mathrm{1}\right)}\:{dx}\: \\ $$
Answered by Dwaipayan Shikari last updated on 02/Aug/20
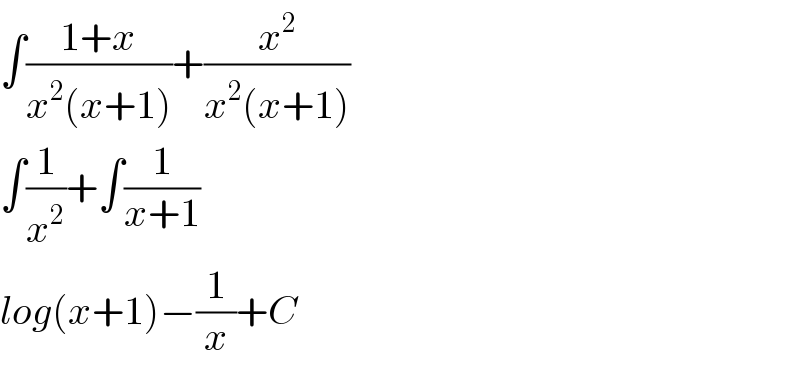
$$\int\frac{\mathrm{1}+{x}}{{x}^{\mathrm{2}} \left({x}+\mathrm{1}\right)}+\frac{{x}^{\mathrm{2}} }{{x}^{\mathrm{2}} \left({x}+\mathrm{1}\right)} \\ $$$$\int\frac{\mathrm{1}}{{x}^{\mathrm{2}} }+\int\frac{\mathrm{1}}{{x}+\mathrm{1}} \\ $$$${log}\left({x}+\mathrm{1}\right)−\frac{\mathrm{1}}{{x}}+{C} \\ $$
