1-x-y-2-x-1-y-3-find-x-2-y-2-

Question Number 91843 by jagoll last updated on 03/May/20

{\begin{cases} \frac{1}{x} + y = 2 \\ x + \frac{1}{y} = 3 \end{cases}

f i n d x^{2} + y^{2}

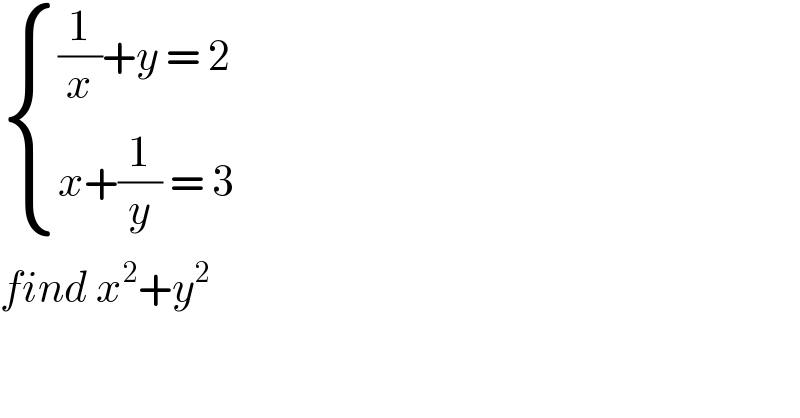
Commented by jagoll last updated on 03/May/20

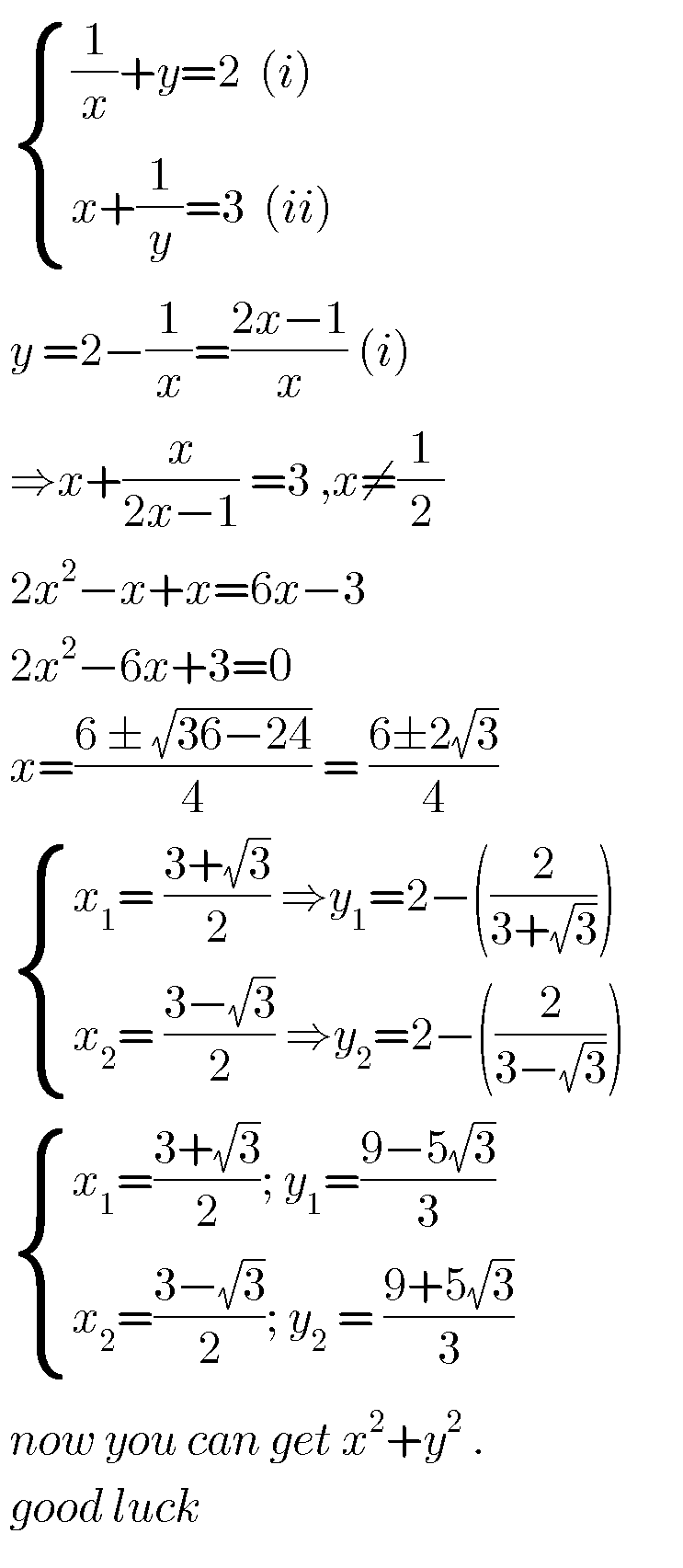
Commented by jagoll last updated on 03/May/20

��

Commented by john santu last updated on 03/May/20

��

Commented by Prithwish Sen 1 last updated on 03/May/20

dividing eqn (i) by (ii)

\frac{y}{x} = \frac{2}{3} \Rightarrow \frac{x}{3} = \frac{y}{2} = k say then x = 3 k, y = 2 k

putting these in any one of the given equation

6 k^{2} - 6 k + 1 = 0 \Rightarrow k = \frac{3 \pm \sqrt{3}}{6} \Rightarrow k^{2} = \frac{2 \pm \sqrt{3}}{6}

∴ x^{2} + y^{2} = (9 + 4) . \frac{2 \pm \sqrt{3}}{6} = 13 \frac{(2 \pm \sqrt{3})}{6}

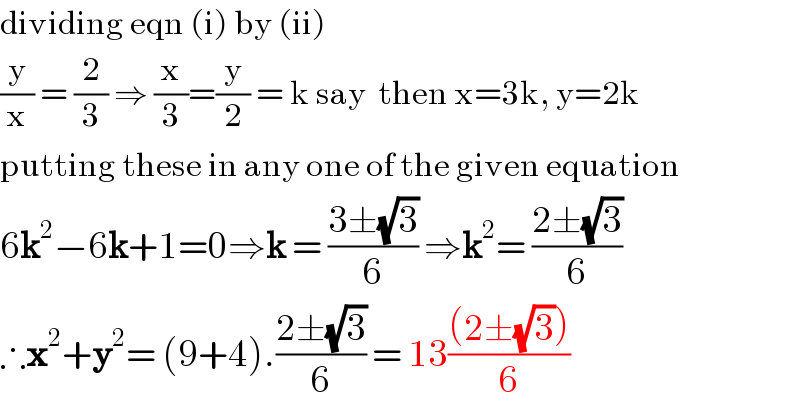
Commented by jagoll last updated on 03/May/20

��

Answered by mr W last updated on 03/May/20

l e t x + y = u, x y = v

(i) \times (i i) :

1 + x y + \frac{1}{x y} + 1 = 6

x y + \frac{1}{x y} = 4

v + \frac{1}{v} = 4

v^{2} - 4 v + 1 = 0

\Rightarrow v = 2 \pm \sqrt{3}

(i) + (i i) :

x + \frac{1}{x} + y + \frac{1}{y} = 5

(x + y) (1 + \frac{1}{x y}) = 5

u (1 + \frac{1}{v}) = 5

\Rightarrow u = \frac{5}{1 + \frac{1}{v}} = \frac{5 v}{v + 1}

x^{2} + y^{2} = {(x + y)}^{2} - 2 x y = u^{2} - 2 v

= \frac{25 v^{2}}{{(v + 1)}^{2}} - 2 v = \frac{13 (2 \pm \sqrt{3})}{6}

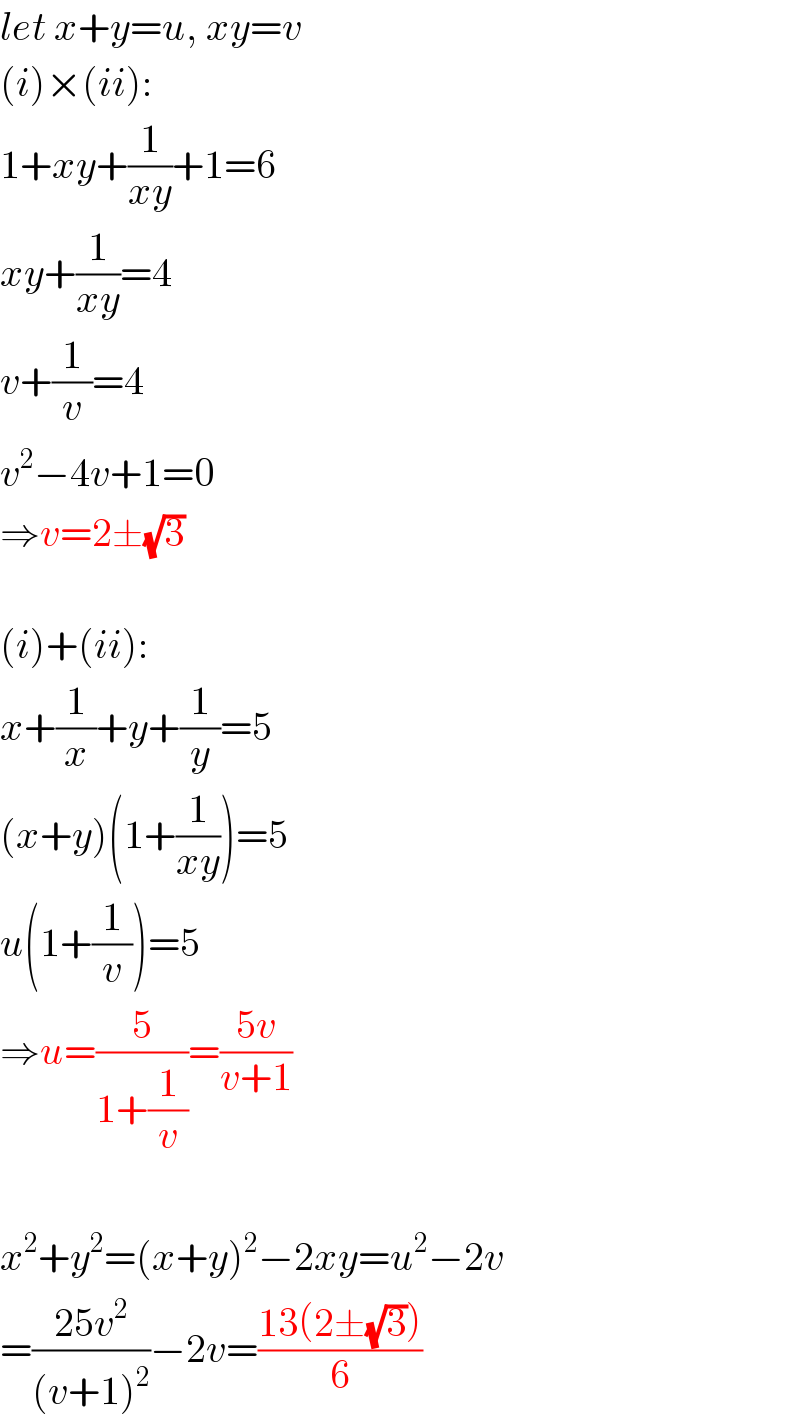
Commented by jagoll last updated on 03/May/20

��

Answered by MJS last updated on 03/May/20

without thinking, without tricks :

{\begin{cases} 1 + x y = 2 x \\ 1 + x y = 3 y \end{cases} \Rightarrow y = \frac{2}{3} x

\Rightarrow 2 x^{2} - 6 x + 3 = 0 \Rightarrow x = \frac{3 \pm \sqrt{3}}{2} \Rightarrow y = \frac{3 \pm \sqrt{3}}{3}

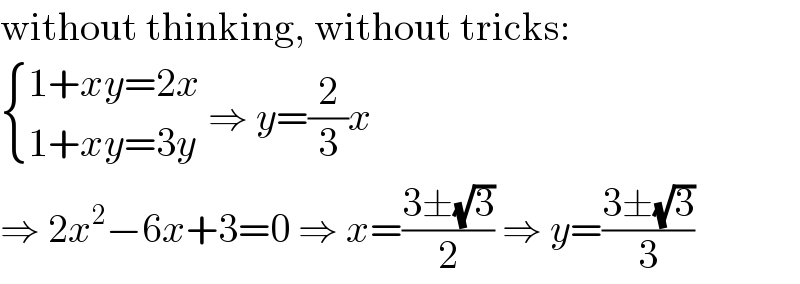
Commented by jagoll last updated on 03/May/20

��

Commented by Ar Brandon last updated on 03/May/20

�� Brilliant !