Question Number 149042 by mathdanisur last updated on 02/Aug/21
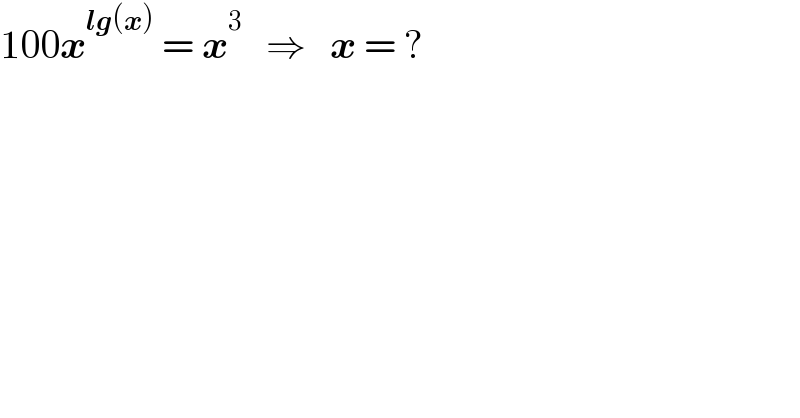
$$\mathrm{100}\boldsymbol{{x}}^{\boldsymbol{{lg}}\left(\boldsymbol{{x}}\right)} \:=\:\boldsymbol{{x}}^{\mathrm{3}} \:\:\:\Rightarrow\:\:\:\boldsymbol{{x}}\:=\:? \\ $$
Answered by bramlexs22 last updated on 02/Aug/21
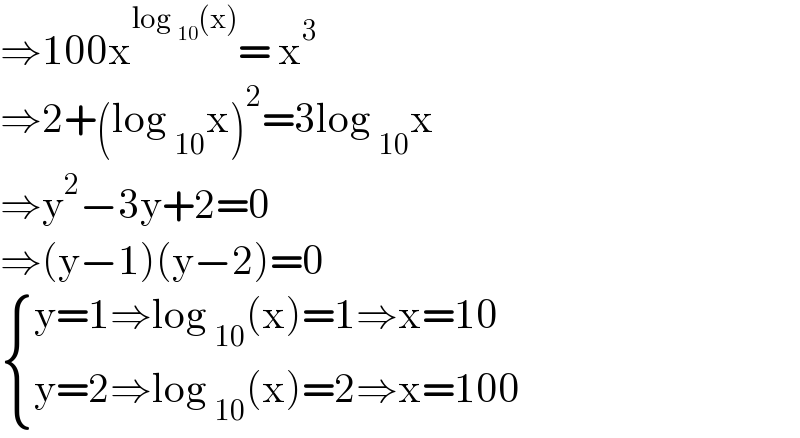
$$\Rightarrow\mathrm{100x}^{\mathrm{log}\:_{\mathrm{10}} \left(\mathrm{x}\right)} =\:\mathrm{x}^{\mathrm{3}} \\ $$$$\Rightarrow\mathrm{2}+\left(\mathrm{log}\:_{\mathrm{10}} \mathrm{x}\right)^{\mathrm{2}} =\mathrm{3log}\:_{\mathrm{10}} \mathrm{x} \\ $$$$\Rightarrow\mathrm{y}^{\mathrm{2}} −\mathrm{3y}+\mathrm{2}=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{y}−\mathrm{1}\right)\left(\mathrm{y}−\mathrm{2}\right)=\mathrm{0} \\ $$$$\begin{cases}{\mathrm{y}=\mathrm{1}\Rightarrow\mathrm{log}\:_{\mathrm{10}} \left(\mathrm{x}\right)=\mathrm{1}\Rightarrow\mathrm{x}=\mathrm{10}}\\{\mathrm{y}=\mathrm{2}\Rightarrow\mathrm{log}\:_{\mathrm{10}} \left(\mathrm{x}\right)=\mathrm{2}\Rightarrow\mathrm{x}=\mathrm{100}}\end{cases} \\ $$
Commented by mathdanisur last updated on 02/Aug/21

$${Thank}\:{You}\:{Ser} \\ $$
