Question Number 147611 by mathdanisur last updated on 22/Jul/21
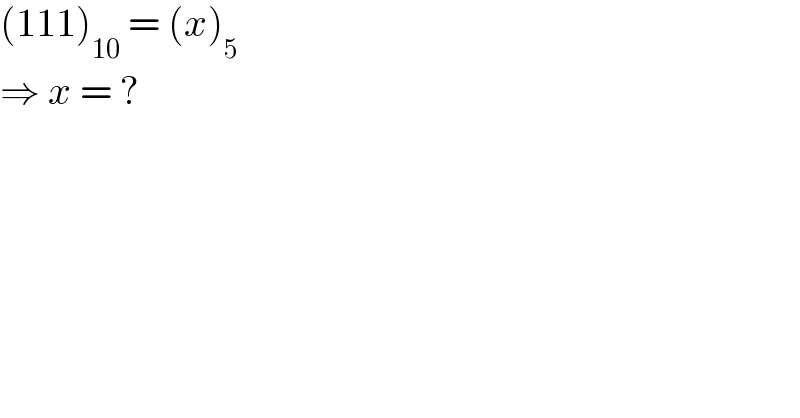
$$\left(\mathrm{111}\right)_{\mathrm{10}} \:=\:\left({x}\right)_{\mathrm{5}} \\ $$$$\Rightarrow\:{x}\:=\:? \\ $$
Answered by bobhans last updated on 22/Jul/21
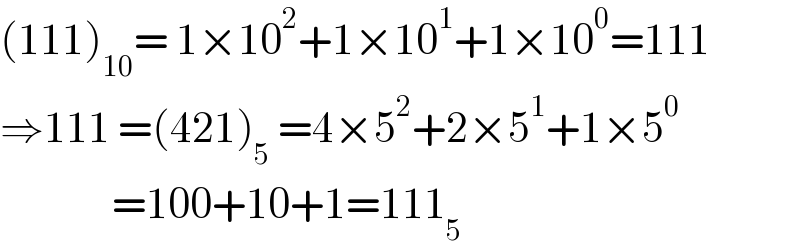
$$\left(\mathrm{111}\right)_{\mathrm{10}} =\:\mathrm{1}×\mathrm{10}^{\mathrm{2}} +\mathrm{1}×\mathrm{10}^{\mathrm{1}} +\mathrm{1}×\mathrm{10}^{\mathrm{0}} =\mathrm{111} \\ $$$$\Rightarrow\mathrm{111}\:=\left(\mathrm{421}\right)_{\mathrm{5}} \:=\mathrm{4}×\mathrm{5}^{\mathrm{2}} +\mathrm{2}×\mathrm{5}^{\mathrm{1}} +\mathrm{1}×\mathrm{5}^{\mathrm{0}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{100}+\mathrm{10}+\mathrm{1}=\mathrm{111}_{\mathrm{5}} \\ $$
Commented by mathdanisur last updated on 22/Jul/21
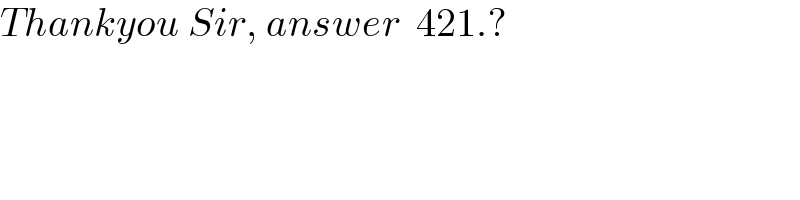
$${Thankyou}\:{Sir},\:{answer}\:\:\mathrm{421}.? \\ $$
Commented by bobhans last updated on 22/Jul/21

$$\mathrm{yes} \\ $$
Commented by mathdanisur last updated on 22/Jul/21

$${thanks}\:{Sir} \\ $$
Answered by gsk2684 last updated on 22/Jul/21

$$\mathrm{5}\mid\mathrm{111} \\ $$$$\mathrm{5}\mid\mathrm{22}\rightarrow\mathrm{1} \\ $$$$\:\:\:\:\mathrm{4}\rightarrow\mathrm{2} \\ $$$$\left(\mathrm{111}\right)_{\mathrm{10}} =\left(\mathrm{421}\right)_{\mathrm{5}} \\ $$
Commented by mathdanisur last updated on 22/Jul/21

$${thankyou}\:{Sir} \\ $$
Answered by Rasheed.Sindhi last updated on 22/Jul/21
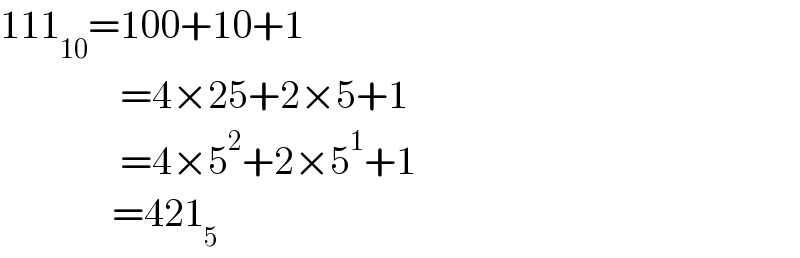
$$\mathrm{111}_{\mathrm{10}} =\mathrm{100}+\mathrm{10}+\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{4}×\mathrm{25}+\mathrm{2}×\mathrm{5}+\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{4}×\mathrm{5}^{\mathrm{2}} +\mathrm{2}×\mathrm{5}^{\mathrm{1}} +\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{421}_{\mathrm{5}} \\ $$
Commented by mathdanisur last updated on 22/Jul/21

$${Thank}\:{you}\:{Sir} \\ $$
