16-64-16-64-16-64-16-1-3-2-1-2-1-2-1-2-1-3-

Question Number 100492 by bobhans last updated on 27/Jun/20

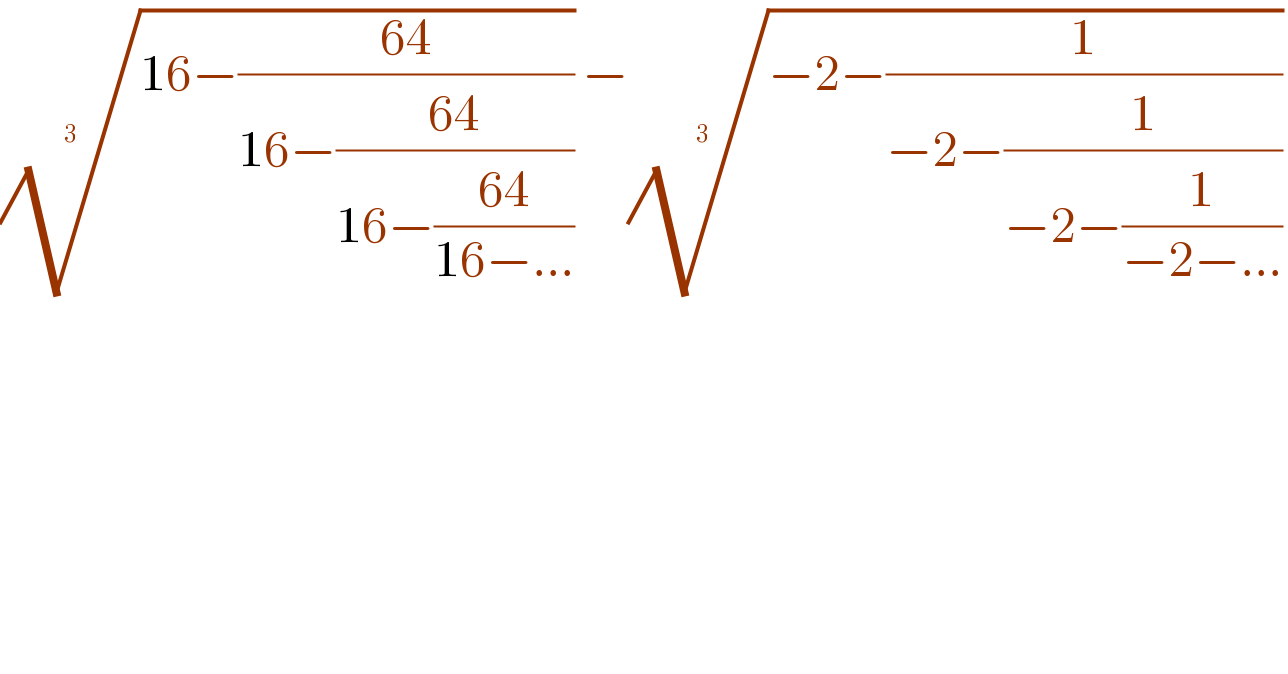
$$\sqrt[{\mathrm{3}\:\:\:}]{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−…}}}}−\sqrt[{\mathrm{3}\:\:}]{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−…}}}} \\ $$

Commented by Dwaipayan Shikari last updated on 27/Jun/20

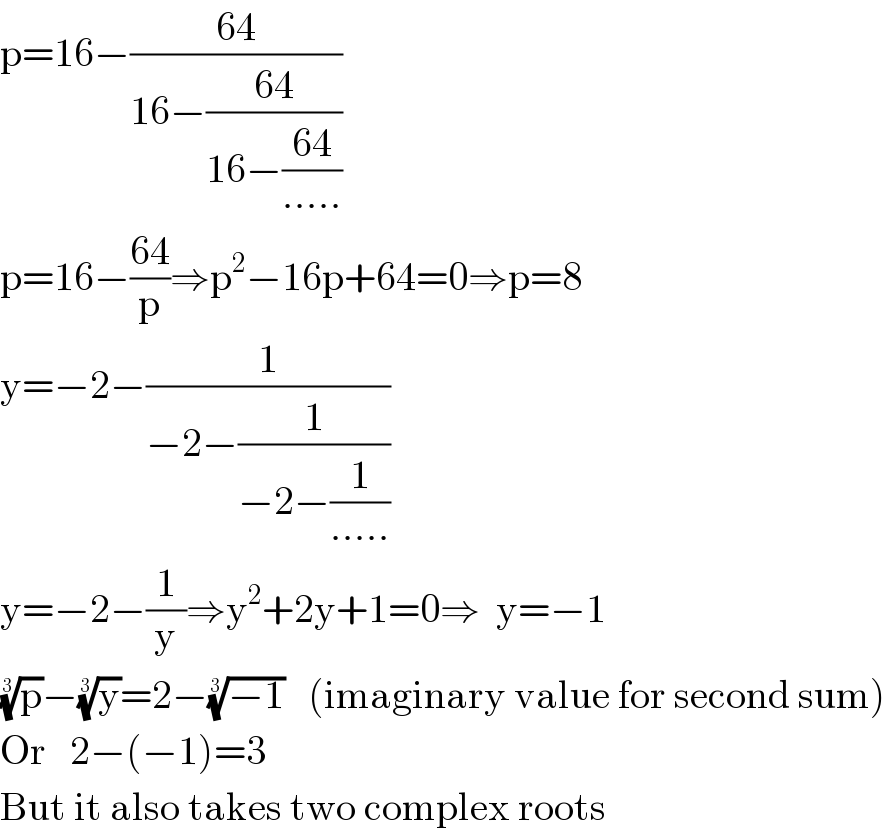
p=16−((64)/(16−((64)/(16−((64)/(.....)))))) p=16−((64)/p)⇒p^2 −16p+64=0⇒p=8 y=−2−(1/(−2−(1/(−2−(1/(.....)))))) y=−2−(1/y)⇒y^2 +2y+1=0⇒ y=−1 (p)^(1/3) −(y)^(1/3) =2−((−1))^(1/3) (imaginary value for second sum) Or 2−(−1)=3 But it also takes two complex roots

$$\mathrm{p}=\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{…..}}} \\ $$$$\mathrm{p}=\mathrm{16}−\frac{\mathrm{64}}{\mathrm{p}}\Rightarrow\mathrm{p}^{\mathrm{2}} −\mathrm{16p}+\mathrm{64}=\mathrm{0}\Rightarrow\mathrm{p}=\mathrm{8} \\ $$$$\mathrm{y}=−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{…..}}} \\ $$$$\mathrm{y}=−\mathrm{2}−\frac{\mathrm{1}}{\mathrm{y}}\Rightarrow\mathrm{y}^{\mathrm{2}} +\mathrm{2y}+\mathrm{1}=\mathrm{0}\Rightarrow\:\:\mathrm{y}=−\mathrm{1} \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{p}}−\sqrt[{\mathrm{3}}]{\mathrm{y}}=\mathrm{2}−\sqrt[{\mathrm{3}}]{−\mathrm{1}}\:\:\:\left(\mathrm{imaginary}\:\mathrm{value}\:\mathrm{for}\:\mathrm{second}\:\mathrm{sum}\right) \\ $$$$\mathrm{Or}\:\:\:\mathrm{2}−\left(−\mathrm{1}\right)=\mathrm{3} \\ $$$$\mathrm{But}\:\mathrm{it}\:\mathrm{also}\:\mathrm{takes}\:\mathrm{two}\:\mathrm{complex}\:\mathrm{roots} \\ $$

Commented by floor(10²Eta[1]) last updated on 27/Jun/20

bro the imaginary value is (√(−1)) the^3 (√(−1)) it′s just −1 [(−1)^3 =−1] (and the other 2 complex roots)

$${bro}\:{the}\:{imaginary}\:{value}\:{is}\:\sqrt{−\mathrm{1}} \\ $$$${the}\:^{\mathrm{3}} \sqrt{−\mathrm{1}}\:{it}'{s}\:{just}\:−\mathrm{1}\:\left[\left(−\mathrm{1}\right)^{\mathrm{3}} =−\mathrm{1}\right] \\ $$$$\left({and}\:{the}\:{other}\:\mathrm{2}\:{complex}\:{roots}\right) \\ $$

Commented by Coronavirus last updated on 27/Jun/20

��

Answered by bramlex last updated on 27/Jun/20

let q = ((16−((64)/(16−((64)/(16−...))))))^(1/3) q^3 = 16−((64)/q) ⇒ q^4 −16q+64 = 0 let r = ((−2−(1/(−2−(1/(−2−...))))))^(1/(3 )) r^3 = −2−(1/r) ⇒ r^4 +2r+1=0 ; r=−1

$${let}\:{q}\:=\:\sqrt[{\mathrm{3}}]{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−…}}} \\ $$$${q}^{\mathrm{3}} \:=\:\mathrm{16}−\frac{\mathrm{64}}{{q}}\:\Rightarrow\:{q}^{\mathrm{4}} −\mathrm{16}{q}+\mathrm{64}\:=\:\mathrm{0} \\ $$$${let}\:{r}\:=\:\sqrt[{\mathrm{3}\:}]{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−…}}} \\ $$$${r}^{\mathrm{3}} \:=\:−\mathrm{2}−\frac{\mathrm{1}}{{r}}\:\Rightarrow\:{r}^{\mathrm{4}} +\mathrm{2}{r}+\mathrm{1}=\mathrm{0}\:;\:{r}=−\mathrm{1} \\ $$$$ \\ $$

Answered by floor(10²Eta[1]) last updated on 27/Jun/20

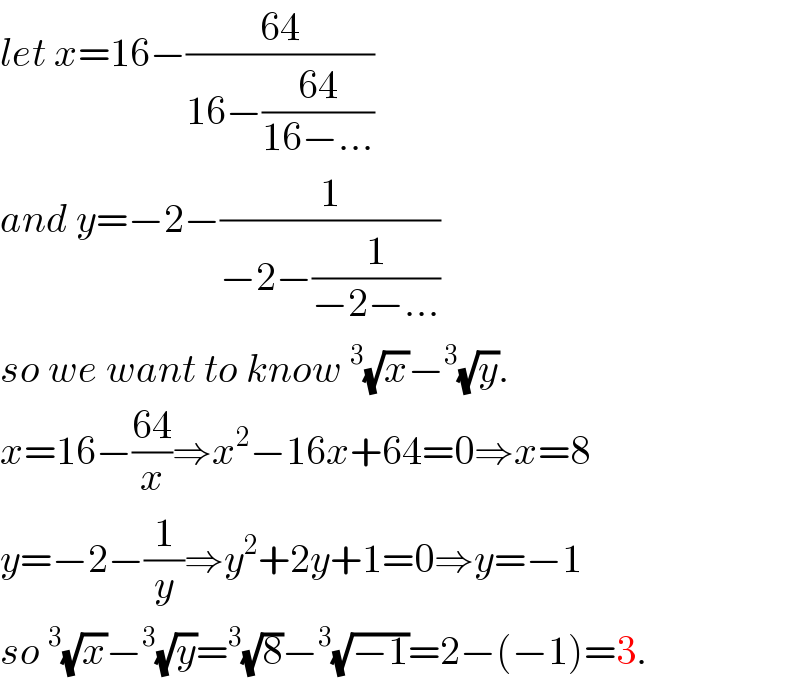
let x=16−((64)/(16−((64)/(16−...)))) and y=−2−(1/(−2−(1/(−2−...)))) so we want to know^3 (√x)−^3 (√y). x=16−((64)/x)⇒x^2 −16x+64=0⇒x=8 y=−2−(1/y)⇒y^2 +2y+1=0⇒y=−1 so^3 (√x)−^3 (√y)=^3 (√8)−^3 (√(−1))=2−(−1)=3.

$${let}\:{x}=\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−\frac{\mathrm{64}}{\mathrm{16}−…}}\:\: \\ $$$${and}\:{y}=−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−\frac{\mathrm{1}}{−\mathrm{2}−…}} \\ $$$${so}\:{we}\:{want}\:{to}\:{know}\:^{\mathrm{3}} \sqrt{{x}}−^{\mathrm{3}} \sqrt{{y}}. \\ $$$${x}=\mathrm{16}−\frac{\mathrm{64}}{{x}}\Rightarrow{x}^{\mathrm{2}} −\mathrm{16}{x}+\mathrm{64}=\mathrm{0}\Rightarrow{x}=\mathrm{8}\: \\ $$$${y}=−\mathrm{2}−\frac{\mathrm{1}}{{y}}\Rightarrow{y}^{\mathrm{2}} +\mathrm{2}{y}+\mathrm{1}=\mathrm{0}\Rightarrow{y}=−\mathrm{1} \\ $$$${so}\:^{\mathrm{3}} \sqrt{{x}}−^{\mathrm{3}} \sqrt{{y}}=^{\mathrm{3}} \sqrt{\mathrm{8}}−^{\mathrm{3}} \sqrt{−\mathrm{1}}=\mathrm{2}−\left(−\mathrm{1}\right)=\mathrm{3}. \\ $$

Facebook Tweet Pin

16-64-16-64-16-64-16-1-3-2-1-2-1-2-1-2-1-3-

Leave a Reply Cancel reply