Question Number 164599 by mathlove last updated on 19/Jan/22
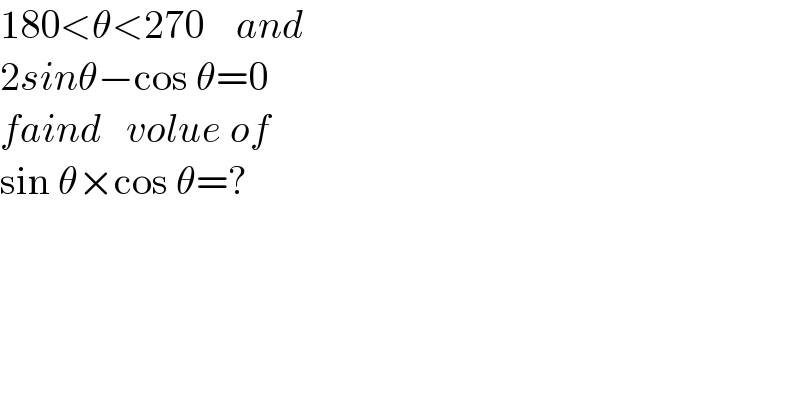
$$\mathrm{180}<\theta<\mathrm{270}\:\:\:\:{and} \\ $$$$\mathrm{2}{sin}\theta−\mathrm{cos}\:\theta=\mathrm{0} \\ $$$${faind}\:\:\:{volue}\:{of} \\ $$$$\mathrm{sin}\:\theta×\mathrm{cos}\:\theta=? \\ $$
Commented by cortano1 last updated on 19/Jan/22

$$\:\mathrm{tan}\:\theta\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:\rightarrow\begin{cases}{\mathrm{sin}\:\theta=−\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}}\\{\mathrm{cos}\:\theta=−\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}}\end{cases} \\ $$$$\:\mathrm{cos}\:\theta\:×\mathrm{sin}\:\theta\:=\:\frac{\mathrm{2}}{\mathrm{5}} \\ $$
