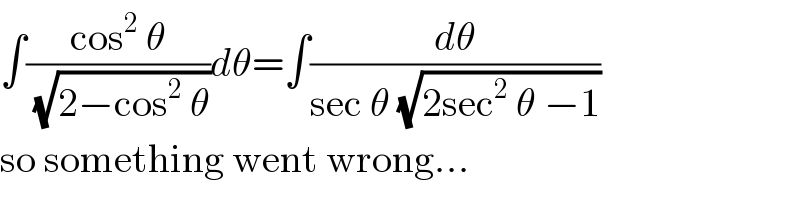2-1-x-2-1-x-2-dx-

Question Number 36892 by anik last updated on 06/Jun/18

2 . \int [\sqrt{(1 - x^{2}) / (1 + x^{2})}] d x = ?

Commented by math khazana by abdo last updated on 11/Jun/18

l e t I = \int \sqrt{\frac{1 - x^{2}}{1 + x^{2}}} d x c h a n g e m e n t x = s i n t g i v e

I = \int \frac{c o s t}{\sqrt{2 {c o s}^{2} t - 1}} c o s t d t

= \int \frac{{c o s}^{2} t}{\sqrt{2 {c o s}^{2} t - 1}} d t = \int \frac{1}{(1 + {t a n}^{2} t) \sqrt{2 \frac{1}{1 + {t a n}^{2} t} - 1}} d t

= \int \frac{d t}{(1 + {t a n}^{2} t) \sqrt{2 - 1 - {t a n}^{2} t}} \sqrt{1 + {t a n}^{2} t} d t

= \int \frac{d t}{\sqrt{1 + {t a n}^{2} t} \sqrt{1 - {t a n}^{2} t}} c h a n g e m e n t

t a n t = u g i v e

I = \int \frac{1}{\sqrt{1 + u^{2}} \sqrt{1 - u^{2}}} \frac{d u}{1 + u^{2}}

= \int \frac{d u}{(1 + u^{2}) \sqrt{1 - u^{4}}} \dots . b e c o n t i n u e d \dots .

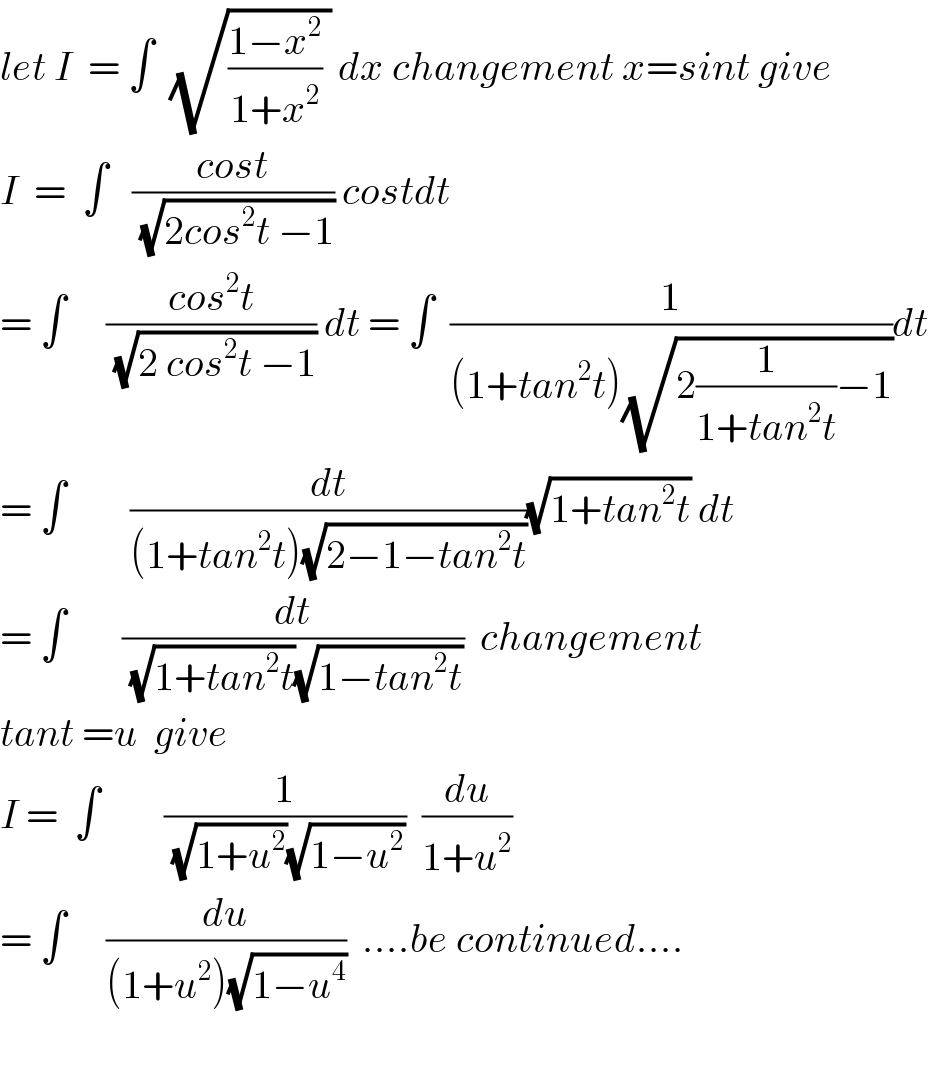
Answered by anik last updated on 06/Jun/18

Commented by anik last updated on 06/Jun/18

Indrajit Karmakar have solved this .

Commented by MJS last updated on 06/Jun/18

\int \frac{\cos^{2} θ}{\sqrt{2 - \cos^{2} θ}} d θ = \int \frac{d θ}{\sec θ \sqrt{{2 \sec}^{2} θ - 1}}

so something went wrong \dots

Facebook Tweet Pin