2-2-2-2-2-2-2-2-

Question Number 124203 by n0y0n last updated on 01/Dec/20

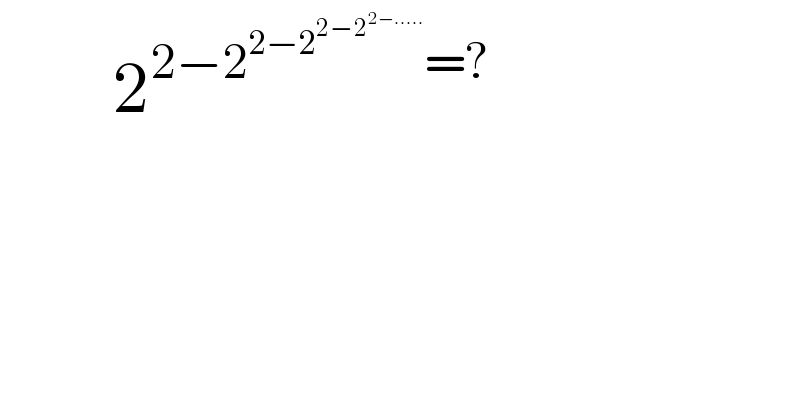
2^{2 - 2^{2 - 2^{2 - 2^{2 - \dots . .}}} = ?}

Commented by n0y0n last updated on 01/Dec/20

pls details

Commented by MJS_new last updated on 01/Dec/20

{it}^{'} s not an answer, this user is not able to

post his own questions \dots

Commented by Ar Brandon last updated on 01/Dec/20

��

Answered by MJS_new last updated on 01/Dec/20

x = 2^{2 - 2^{2 - 2^{\dots}}}

\ln x = (2 - x) \ln 2

let t = \frac{\ln x}{\ln 2}

2^{t} + t - 2 = 0

approximating I get t \approx . 543000440865

\Rightarrow x \approx 1.45699955913

Answered by mr W last updated on 01/Dec/20

x = 2^{2 - x}

x 2^{x} = 2^{2}

{x e}^{x \ln 2} = 4

x \ln 2 e^{x \ln 2} = 4 \ln 2

x \ln 2 = W (4 \ln 2)

\Rightarrow x = \frac{W (4 \ln 2)}{\ln 2} \approx 1.457

Facebook Tweet Pin