Question Number 180990 by Acem last updated on 20/Nov/22
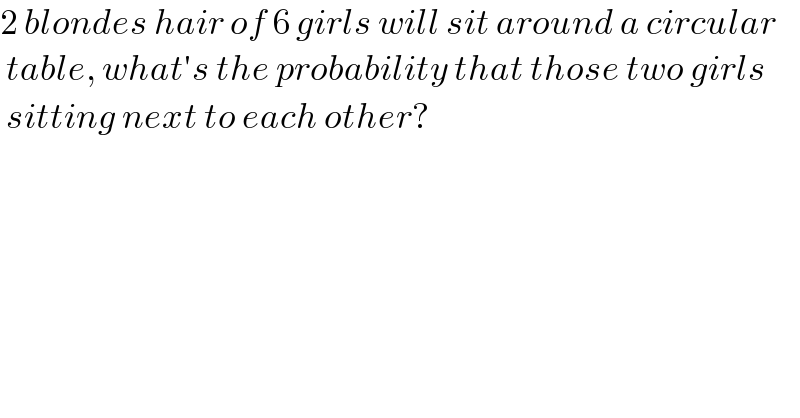
$$\mathrm{2}\:{blondes}\:{hair}\:{of}\:\mathrm{6}\:{girls}\:{will}\:{sit}\:{around}\:{a}\:{circular} \\ $$$$\:{table},\:{what}'{s}\:{the}\:{probability}\:{that}\:{those}\:{two}\:{girls} \\ $$$$\:{sitting}\:{next}\:{to}\:{each}\:{other}? \\ $$
Answered by mr W last updated on 20/Nov/22
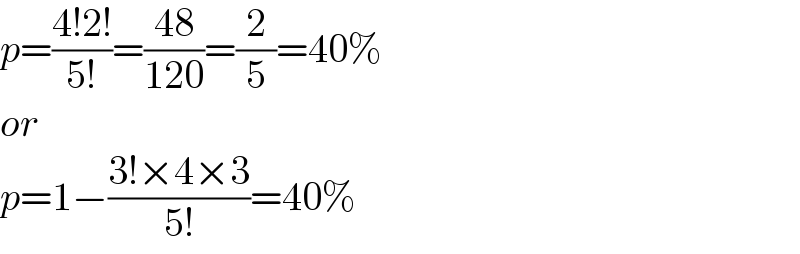
$${p}=\frac{\mathrm{4}!\mathrm{2}!}{\mathrm{5}!}=\frac{\mathrm{48}}{\mathrm{120}}=\frac{\mathrm{2}}{\mathrm{5}}=\mathrm{40\%} \\ $$$${or} \\ $$$${p}=\mathrm{1}−\frac{\mathrm{3}!×\mathrm{4}×\mathrm{3}}{\mathrm{5}!}=\mathrm{40\%} \\ $$
Commented by Acem last updated on 20/Nov/22

$$\checkmark\: \\ $$$${Very}\:{well}\:{Sir}! \\ $$
