Question Number 31249 by Joel578 last updated on 04/Mar/18
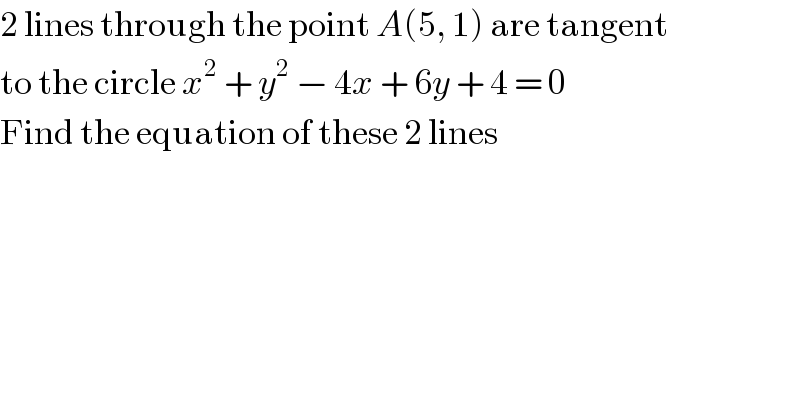
Commented by Joel578 last updated on 04/Mar/18

Commented by Joel578 last updated on 04/Mar/18
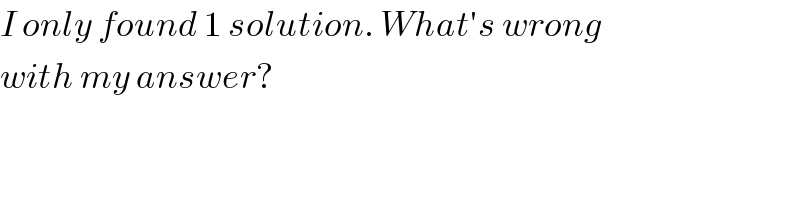
Commented by ajfour last updated on 04/Mar/18

Commented by Joel578 last updated on 05/Mar/18
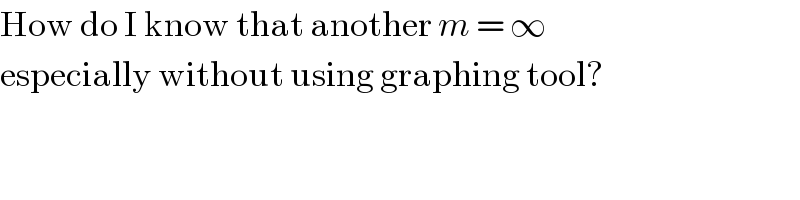
Commented by ajfour last updated on 05/Mar/18
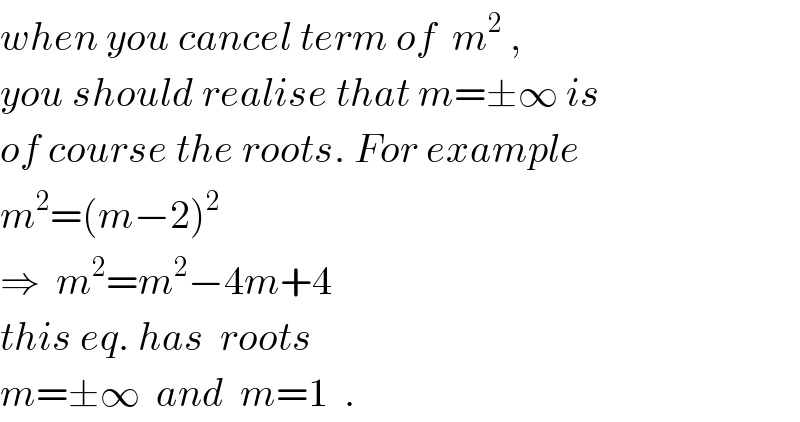
Commented by Joel578 last updated on 05/Mar/18
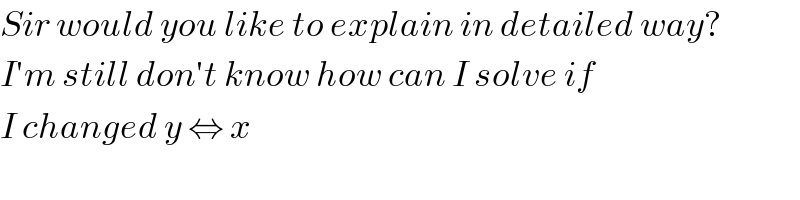
Commented by Joel578 last updated on 05/Mar/18

Commented by MJS last updated on 05/Mar/18
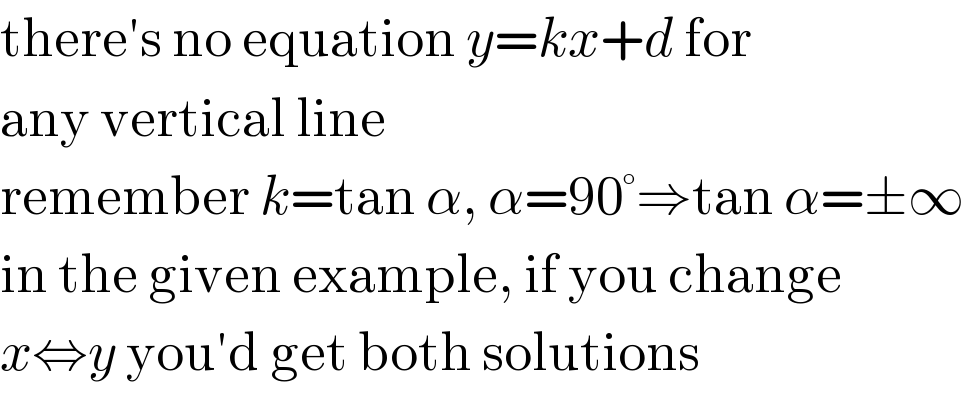
Commented by MJS last updated on 05/Mar/18
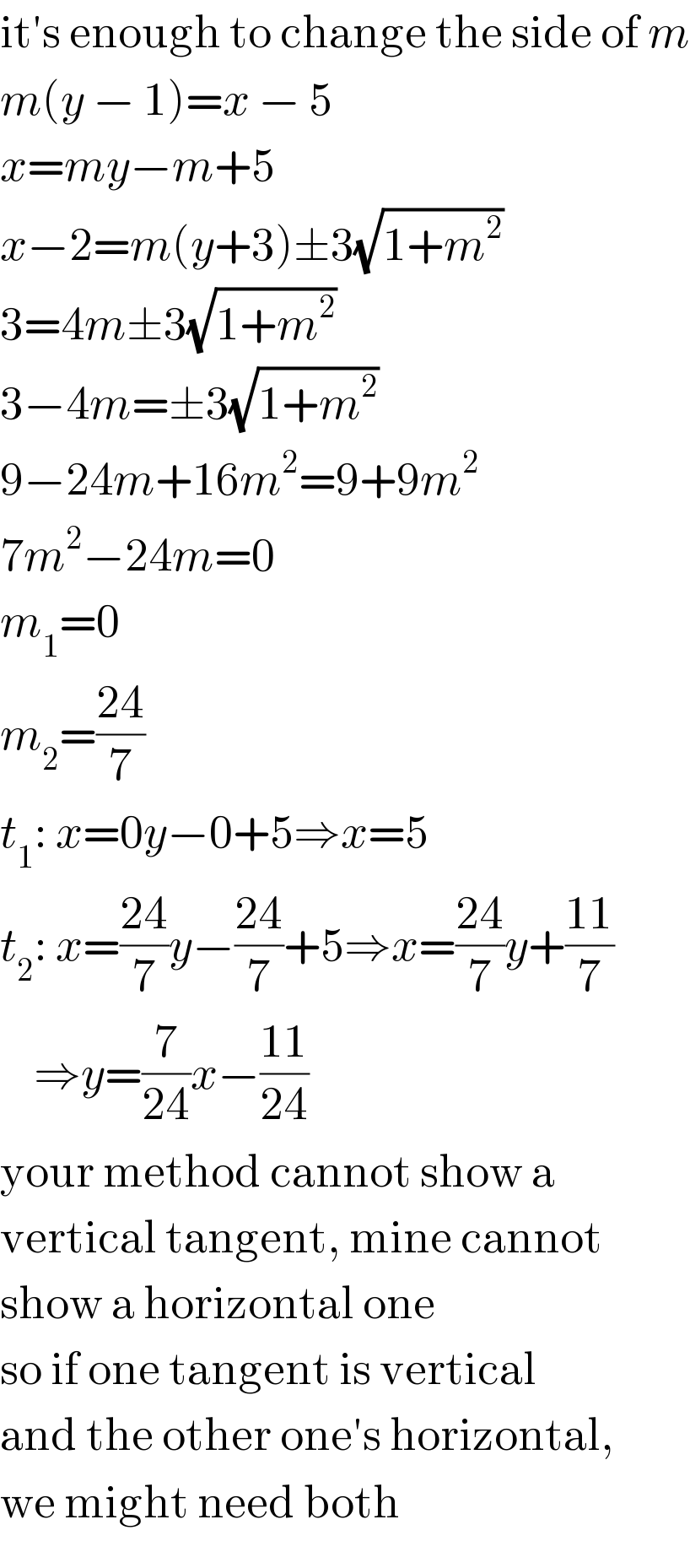
Commented by Joel578 last updated on 06/Mar/18