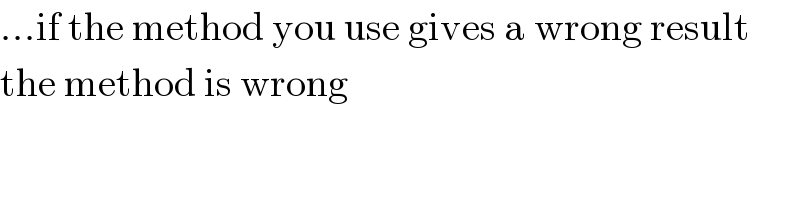Question Number 92196 by I want to learn more last updated on 05/May/20

Commented by john santu last updated on 05/May/20
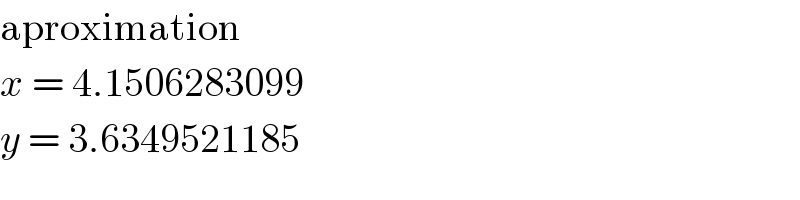
Commented by I want to learn more last updated on 05/May/20
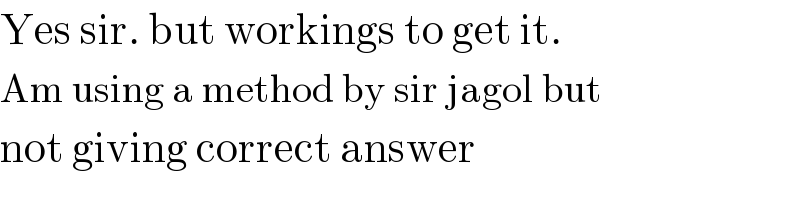
Commented by MJS last updated on 05/May/20
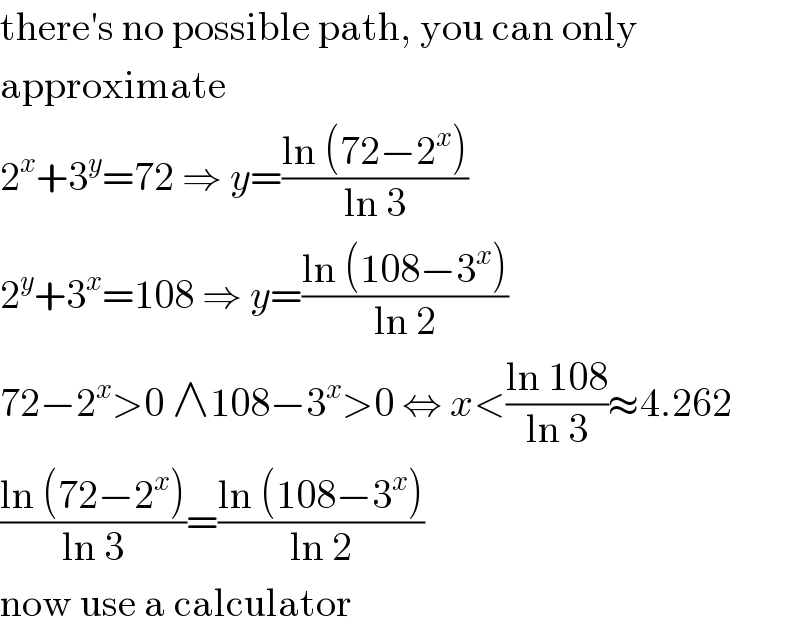
Commented by MJS last updated on 05/May/20