Question Number 40583 by Cheyboy last updated on 24/Jul/18

Answered by $@ty@m last updated on 24/Jul/18

Commented by Cheyboy last updated on 24/Jul/18
Answered by MrW3 last updated on 24/Jul/18
![let t=(√x)>0 2^t =t^2 2^(t/2) =t e^((ln 2 t)/2) =t −((ln 2)/2)=−((ln 2 t)/2) e^(−((ln 2 t)/2)) ⇒−((ln 2 t)/2)=W(−((ln 2)/2)) ⇒t=−(2/(ln 2)) W(−((ln 2)/2)) ⇒x=t^2 =[−(2/(ln 2)) W(−((ln 2)/2))]^2 = { (((−((−2×1.3863)/(ln 2)))^2 =16)),(((−((−2×0.6931)/(ln 2)))^2 =4)) :}](https://www.tinkutara.com/question/Q40586.png)
Commented by Cheyboy last updated on 24/Jul/18

Commented by MrW3 last updated on 24/Jul/18

Commented by Cheyboy last updated on 25/Jul/18
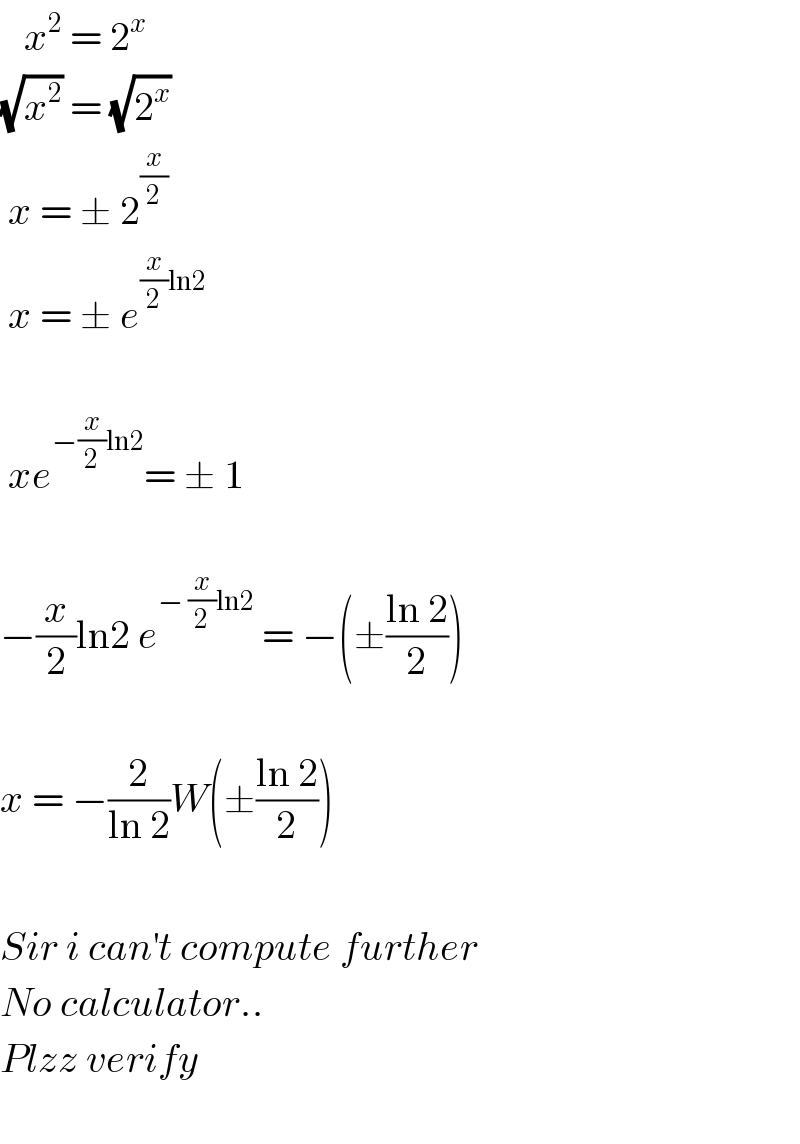
Commented by MrW3 last updated on 25/Jul/18

Commented by Cheyboy last updated on 26/Jul/18

