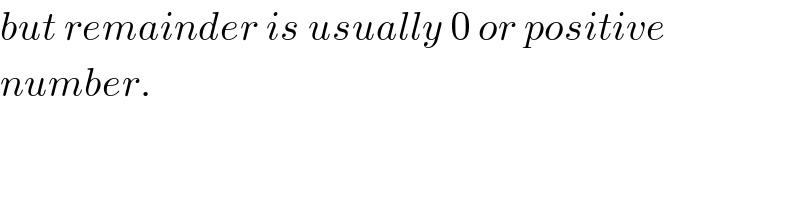Question Number 90200 by Ar Brandon last updated on 21/Apr/20
![26≡R_1 [37] 1 ≡R_2 [3] 2≡R_3 [5] Find R_1 , R_2 and R_3](https://www.tinkutara.com/question/Q90200.png)
Commented by Rio Michael last updated on 22/Apr/20
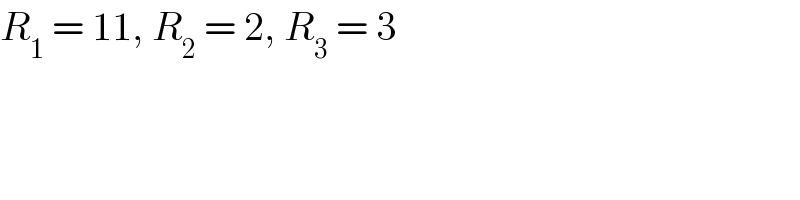
Commented by Ar Brandon last updated on 22/Apr/20

Commented by Rio Michael last updated on 22/Apr/20
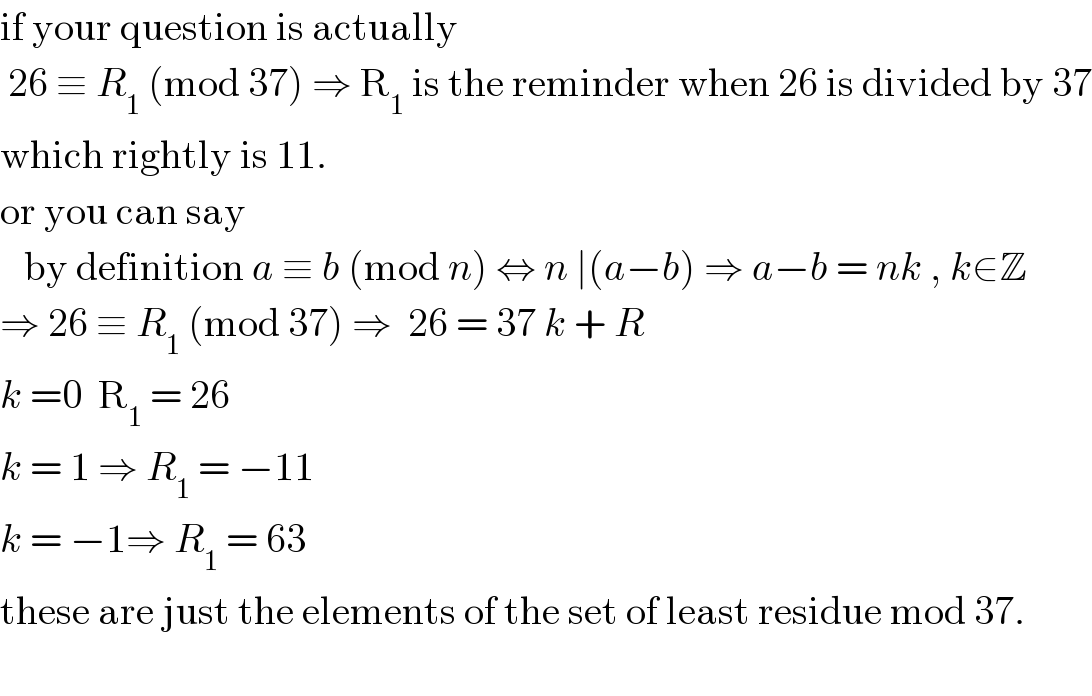
Commented by Ar Brandon last updated on 22/Apr/20

Commented by mr W last updated on 22/Apr/20

Commented by Ar Brandon last updated on 22/Apr/20
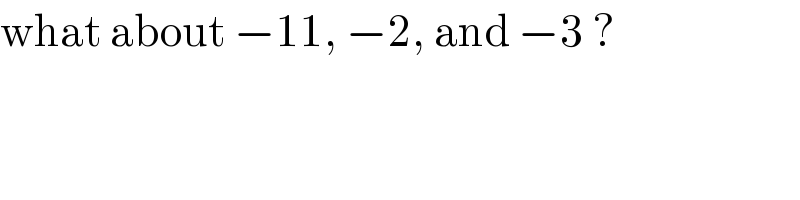
Commented by mr W last updated on 22/Apr/20