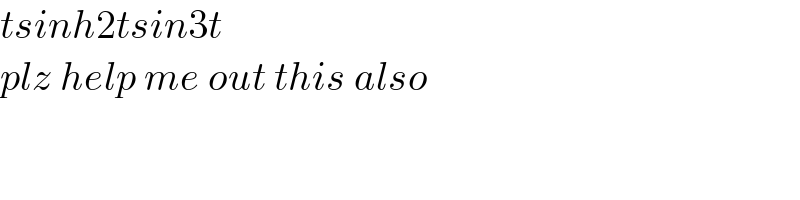Question Number 128460 by BHOOPENDRA last updated on 07/Jan/21
$$\mathrm{2}{e}^{\mathrm{3}{t}} {sin}\mathrm{4}{t}\: \\ $$$${find}\:{laplace}\:{transformation}? \\ $$
Answered by Dwaipayan Shikari last updated on 07/Jan/21
$$\mathscr{L}\left(\mathrm{2}{e}^{\mathrm{3}{t}} {sin}\mathrm{4}{t}\right) \\ $$$$=\frac{\mathrm{1}}{{i}}\int_{\mathrm{0}} ^{\infty} \left({e}^{\mathrm{3}{t}} {e}^{\mathrm{4}{it}} −{e}^{−\mathrm{4}{it}} {e}^{\mathrm{3}{t}} \right){e}^{−{st}} {dt} \\ $$$$=\frac{\mathrm{1}}{{i}}\int_{\mathrm{0}} ^{\infty} {e}^{−\left({s}−\mathrm{3}−\mathrm{4}{i}\right){t}} −{e}^{−\left({s}+\mathrm{4}{i}−\mathrm{3}\right){t}} {dt} \\ $$$$=\frac{\mathrm{1}}{{i}}\left(\frac{\mathrm{1}}{\left({s}−\mathrm{3}−\mathrm{4}{i}\right)}−\frac{\mathrm{1}}{\left({s}−\mathrm{3}+\mathrm{4}{i}\right)}\right)\Gamma\left(\mathrm{1}\right) \\ $$$$=\frac{\mathrm{1}}{{i}}\left(\frac{\mathrm{8}{i}}{\left({s}^{\mathrm{2}} −\mathrm{6}{s}+\mathrm{9}+\mathrm{16}\right)}\right)=\frac{\mathrm{8}}{{s}^{\mathrm{2}} −\mathrm{6}{s}+\mathrm{25}} \\ $$
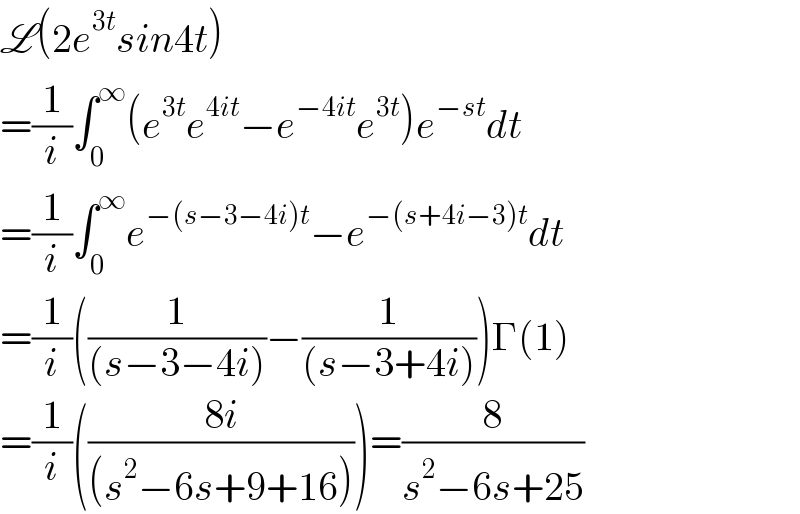
Commented by BHOOPENDRA last updated on 07/Jan/21
$${tsinh}\mathrm{2}{tsin}\mathrm{3}{t} \\ $$$${plz}\:{help}\:{me}\:{out}\:{this}\: \\ $$$${n}\:{thanks}\:{for}\:{this}\:{solution} \\ $$
Answered by mnjuly1970 last updated on 07/Jan/21
![solution: L [f(t)]=F (s) L [e^(at) f(t)]=F(s−a).... L [2e^(3t) sin(4t)]=2∗(4/((s−3)^2 +16)) =(8/(s^2 −6s+25))](https://www.tinkutara.com/question/Q128470.png)
$${solution}: \\ $$$$\mathscr{L}\:\left[{f}\left({t}\right)\right]={F}\:\left({s}\right) \\ $$$$\:\:\mathscr{L}\:\left[{e}^{{at}} {f}\left({t}\right)\right]={F}\left({s}−{a}\right)…. \\ $$$$\mathscr{L}\:\left[\mathrm{2}{e}^{\mathrm{3}{t}} {sin}\left(\mathrm{4}{t}\right)\right]=\mathrm{2}\ast\frac{\mathrm{4}}{\left({s}−\mathrm{3}\right)^{\mathrm{2}} +\mathrm{16}} \\ $$$$\:\:\:=\frac{\mathrm{8}}{{s}^{\mathrm{2}} −\mathrm{6}{s}+\mathrm{25}} \\ $$
Commented by BHOOPENDRA last updated on 07/Jan/21
$${tsinh}\mathrm{2}{tsin}\mathrm{3}{t}\: \\ $$$${plz}\:{help}\:{me}\:{out}\:{this}\:{also} \\ $$