Menu Close

3-cos-6x-2-sin-3x-cos-4x-4tan-2-4x-

Tinku Tara 0 Comments
Pin




Question Number 129958 by bemath last updated on 21/Jan/21
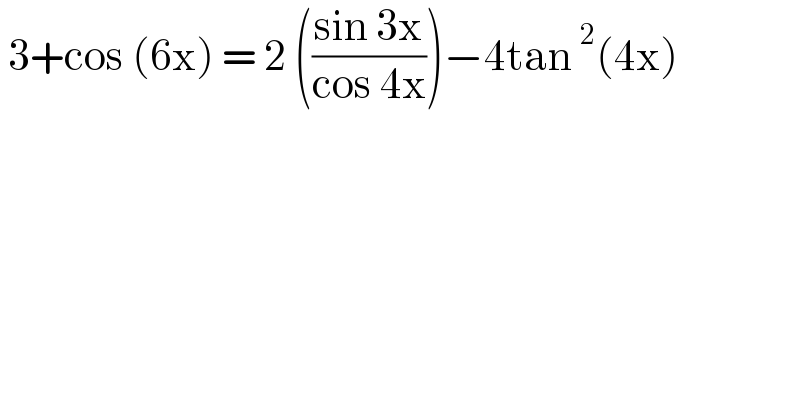
3+cos (6x) = 2 (((sin 3x)/(cos 4x)))−4tan^2 (4x)
$$\:\mathrm{3}+\mathrm{cos}\:\left(\mathrm{6x}\right)\:=\:\mathrm{2}\:\left(\frac{\mathrm{sin}\:\mathrm{3x}}{\mathrm{cos}\:\mathrm{4x}}\right)−\mathrm{4tan}\:^{\mathrm{2}} \left(\mathrm{4x}\right) \\ $$
Answered by MJS_new last updated on 21/Jan/21
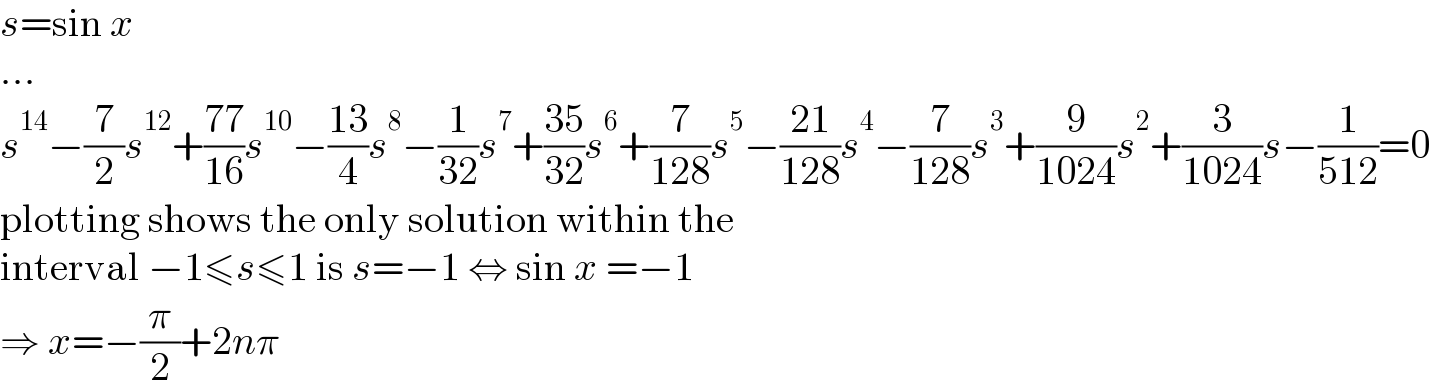
s=sin x ... s^(14) −(7/2)s^(12) +((77)/(16))s^(10) −((13)/4)s^8 −(1/(32))s^7 +((35)/(32))s^6 +(7/(128))s^5 −((21)/(128))s^4 −(7/(128))s^3 +(9/(1024))s^2 +(3/(1024))s−(1/(512))=0 plotting shows the only solution within the interval −1≤s≤1 is s=−1 ⇔ sin x =−1 ⇒ x=−(π/2)+2nπ
$${s}=\mathrm{sin}\:{x} \\ $$$$… \\ $$$${s}^{\mathrm{14}} −\frac{\mathrm{7}}{\mathrm{2}}{s}^{\mathrm{12}} +\frac{\mathrm{77}}{\mathrm{16}}{s}^{\mathrm{10}} −\frac{\mathrm{13}}{\mathrm{4}}{s}^{\mathrm{8}} −\frac{\mathrm{1}}{\mathrm{32}}{s}^{\mathrm{7}} +\frac{\mathrm{35}}{\mathrm{32}}{s}^{\mathrm{6}} +\frac{\mathrm{7}}{\mathrm{128}}{s}^{\mathrm{5}} −\frac{\mathrm{21}}{\mathrm{128}}{s}^{\mathrm{4}} −\frac{\mathrm{7}}{\mathrm{128}}{s}^{\mathrm{3}} +\frac{\mathrm{9}}{\mathrm{1024}}{s}^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{1024}}{s}−\frac{\mathrm{1}}{\mathrm{512}}=\mathrm{0} \\ $$$$\mathrm{plotting}\:\mathrm{shows}\:\mathrm{the}\:\mathrm{only}\:\mathrm{solution}\:\mathrm{within}\:\mathrm{the} \\ $$$$\mathrm{interval}\:−\mathrm{1}\leqslant{s}\leqslant\mathrm{1}\:\mathrm{is}\:{s}=−\mathrm{1}\:\Leftrightarrow\:\mathrm{sin}\:{x}\:=−\mathrm{1} \\ $$$$\Rightarrow\:{x}=−\frac{\pi}{\mathrm{2}}+\mathrm{2}{n}\pi \\ $$
Commented by EDWIN88 last updated on 22/Jan/21
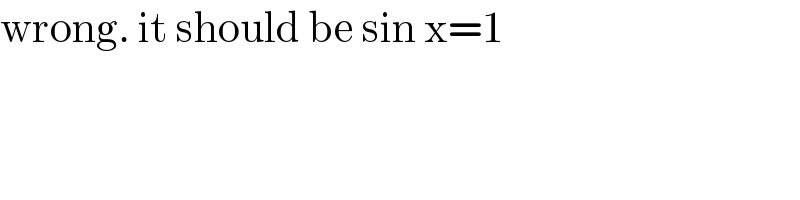
wrong. it should be sin x=1
$$\mathrm{wrong}.\:\mathrm{it}\:\mathrm{should}\:\mathrm{be}\:\mathrm{sin}\:\mathrm{x}=\mathrm{1} \\ $$
Commented by MJS_new last updated on 22/Jan/21
no. you forgot to test your solution with x=(π/2) in the given equation you get 2=−2 within 0≤x<2π the only solution is ((3π)/2)
$$\mathrm{no}.\:\mathrm{you}\:\mathrm{forgot}\:\mathrm{to}\:\mathrm{test}\:\mathrm{your}\:\mathrm{solution} \\ $$$$\mathrm{with}\:{x}=\frac{\pi}{\mathrm{2}}\:\mathrm{in}\:\mathrm{the}\:\mathrm{given}\:\mathrm{equation}\:\mathrm{you}\:\mathrm{get}\:\mathrm{2}=−\mathrm{2} \\ $$$$\mathrm{within}\:\mathrm{0}\leqslant{x}<\mathrm{2}\pi\:\mathrm{the}\:\mathrm{only}\:\mathrm{solution}\:\mathrm{is}\:\frac{\mathrm{3}\pi}{\mathrm{2}} \\ $$
Answered by EDWIN88 last updated on 22/Jan/21
3 +cos 6x = ((2sin 3x)/(cos 4x)) −((4sin^2 4x)/(cos^2 4x)) 3cos^2 4x+cos^2 4x cos 6x=2sin 3xcos 4x−4sin^2 4x 3cos^2 4x+cos^2 4x cos 6x=2sin 3x cos 4x−4+4cos^2 4x cos^2 4x cos 6x = 2sin 3x cos 4x−4+cos^2 4x cos^2 4x (1−2sin^2 3x)=2sin 3x cos 4x+cos^2 4x−4 cos^2 4x−2sin^2 3x cos^2 4x = 2sin 3x cos 4x +cos^2 4x−4 2sin^2 3x cos^2 4x +2sin 3x cos 3x −4=0 (sin 3x cos 4x)^2 +sin 3x cos 4x−2=0 let sin 3x cos 4x = y y^2 +y−2=0 →(y−1)(y+2)=0 for y = 1 ⇒sin 3x cos 4x=1 for y=−2⇒sin 3x cos 4x = −2 { ((sin 3x=3sin x−4sin^3 x)),((cos 4x=1−2sin^2 2x =1−2(4sin^2 x cos^2 x))) :} cos 4x = 1−8sin^2 x(1−sin^2 x) = 1−8sin^2 x+8sin^4 x (∗)(3sin x−4sin^3 x)(8sin^4 x−8sin^2 x+1)−1=0 24sin^5 x−24sin^3 x+3sin x−32sin^7 x+32sin^5 x−4sin^3 x−1=0 −32sin^7 x+56sin^5 x−28sin^3 x+3sin x−1=0 (sin x+1)(−32sin^6 x+32sin^5 x+24sin^4 x−24sin^3 x−4sin^2 x+4sin x−1)=0 ⇔ sin x = −1=sin (−(π/2)) x = 2nπ−(π/2) ; n∈Z
$$\mathrm{3}\:+\mathrm{cos}\:\mathrm{6x}\:=\:\frac{\mathrm{2sin}\:\mathrm{3x}}{\mathrm{cos}\:\mathrm{4x}}\:−\frac{\mathrm{4sin}\:^{\mathrm{2}} \mathrm{4x}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}} \\ $$$$\mathrm{3cos}\:^{\mathrm{2}} \mathrm{4x}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:\mathrm{cos}\:\mathrm{6x}=\mathrm{2sin}\:\mathrm{3xcos}\:\mathrm{4x}−\mathrm{4sin}\:^{\mathrm{2}} \mathrm{4x} \\ $$$$\mathrm{3cos}\:^{\mathrm{2}} \mathrm{4x}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:\mathrm{cos}\:\mathrm{6x}=\mathrm{2sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}−\mathrm{4}+\mathrm{4cos}\:^{\mathrm{2}} \mathrm{4x} \\ $$$$\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:\mathrm{cos}\:\mathrm{6x}\:=\:\mathrm{2sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}−\mathrm{4}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x} \\ $$$$\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:\left(\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{3x}\right)=\mathrm{2sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}−\mathrm{4} \\ $$$$\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{3x}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:=\:\mathrm{2sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}\:+\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}−\mathrm{4} \\ $$$$\:\mathrm{2sin}\:^{\mathrm{2}} \mathrm{3x}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{4x}\:+\mathrm{2sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{3x}\:−\mathrm{4}=\mathrm{0} \\ $$$$\:\left(\mathrm{sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}\right)^{\mathrm{2}} +\mathrm{sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}−\mathrm{2}=\mathrm{0} \\ $$$$\:\mathrm{let}\:\mathrm{sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}\:=\:\mathrm{y} \\ $$$$\:\mathrm{y}^{\mathrm{2}} +\mathrm{y}−\mathrm{2}=\mathrm{0}\:\rightarrow\left(\mathrm{y}−\mathrm{1}\right)\left(\mathrm{y}+\mathrm{2}\right)=\mathrm{0} \\ $$$$\:\mathrm{for}\:\mathrm{y}\:=\:\mathrm{1}\:\Rightarrow\mathrm{sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}=\mathrm{1} \\ $$$$\:\mathrm{for}\:\mathrm{y}=−\mathrm{2}\Rightarrow\mathrm{sin}\:\mathrm{3x}\:\mathrm{cos}\:\mathrm{4x}\:=\:−\mathrm{2} \\ $$$$\begin{cases}{\mathrm{sin}\:\mathrm{3x}=\mathrm{3sin}\:\mathrm{x}−\mathrm{4sin}\:^{\mathrm{3}} \mathrm{x}}\\{\mathrm{cos}\:\mathrm{4x}=\mathrm{1}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{2x}\:=\mathrm{1}−\mathrm{2}\left(\mathrm{4sin}\:^{\mathrm{2}} \mathrm{x}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}\right)}\end{cases} \\ $$$$\:\:\mathrm{cos}\:\mathrm{4x}\:=\:\mathrm{1}−\mathrm{8sin}\:^{\mathrm{2}} \mathrm{x}\left(\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{1}−\mathrm{8sin}\:^{\mathrm{2}} \mathrm{x}+\mathrm{8sin}\:^{\mathrm{4}} \mathrm{x}\: \\ $$$$\:\left(\ast\right)\left(\mathrm{3sin}\:\mathrm{x}−\mathrm{4sin}\:^{\mathrm{3}} \mathrm{x}\right)\left(\mathrm{8sin}\:^{\mathrm{4}} \mathrm{x}−\mathrm{8sin}\:^{\mathrm{2}} \mathrm{x}+\mathrm{1}\right)−\mathrm{1}=\mathrm{0} \\ $$$$\:\mathrm{24sin}\:^{\mathrm{5}} \mathrm{x}−\mathrm{24sin}\:^{\mathrm{3}} \mathrm{x}+\mathrm{3sin}\:\mathrm{x}−\mathrm{32sin}\:^{\mathrm{7}} \mathrm{x}+\mathrm{32sin}\:^{\mathrm{5}} \mathrm{x}−\mathrm{4sin}\:^{\mathrm{3}} \mathrm{x}−\mathrm{1}=\mathrm{0} \\ $$$$−\mathrm{32sin}\:^{\mathrm{7}} \mathrm{x}+\mathrm{56sin}\:^{\mathrm{5}} \mathrm{x}−\mathrm{28sin}\:^{\mathrm{3}} \mathrm{x}+\mathrm{3sin}\:\mathrm{x}−\mathrm{1}=\mathrm{0} \\ $$$$\left(\mathrm{sin}\:\mathrm{x}+\mathrm{1}\right)\left(−\mathrm{32sin}\:^{\mathrm{6}} \mathrm{x}+\mathrm{32sin}\:^{\mathrm{5}} \mathrm{x}+\mathrm{24sin}\:^{\mathrm{4}} \mathrm{x}−\mathrm{24sin}\:^{\mathrm{3}} \mathrm{x}−\mathrm{4sin}\:^{\mathrm{2}} \mathrm{x}+\mathrm{4sin}\:\mathrm{x}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\Leftrightarrow\:\mathrm{sin}\:\mathrm{x}\:=\:−\mathrm{1}=\mathrm{sin}\:\left(−\frac{\pi}{\mathrm{2}}\right) \\ $$$$\:\:\:\:\:\:\mathrm{x}\:=\:\mathrm{2n}\pi−\frac{\pi}{\mathrm{2}}\:;\:\mathrm{n}\in\mathbb{Z} \\ $$
Commented by MJS_new last updated on 22/Jan/21
thank you for checking it again
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{for}\:\mathrm{checking}\:\mathrm{it}\:\mathrm{again} \\ $$
Commented by EDWIN88 last updated on 22/Jan/21
thanks too
$$\mathrm{thanks}\:\mathrm{too} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *