Question Number 79306 by jagoll last updated on 24/Jan/20

Commented by kaivan.ahmadi last updated on 24/Jan/20
![3−x≥0⇒x≤3 x+1≥0⇒x≥−1 ⇒−1≤x≤3 3−x+x−1−2(√((3−x)(x+1)))>(1/4)⇒ −2(√((3−x)(x+1)))>−(7/4)⇒(√((3−x)(x+1)))<(7/8)⇒ (3−x)(x+1)<((49)/(64))⇒3−x^2 +2x+3<((49)/(64))⇒ x^2 −2x−((143)/(64))>0 Δ=4+((143)/(16))=((207)/(64)) x_(1,2) =((2±((√(207))/8))/2)=1±((√(207))/(16))=1±((3(√(23)))/(16)) x∈[−1,1−((3(√(23)))/(16))]∪[1+((3(√(23)))/(16)),3]](https://www.tinkutara.com/question/Q79324.png)
Commented by key of knowledge last updated on 24/Jan/20

Commented by kaivan.ahmadi last updated on 24/Jan/20

Commented by kaivan.ahmadi last updated on 24/Jan/20

Commented by john santu last updated on 24/Jan/20
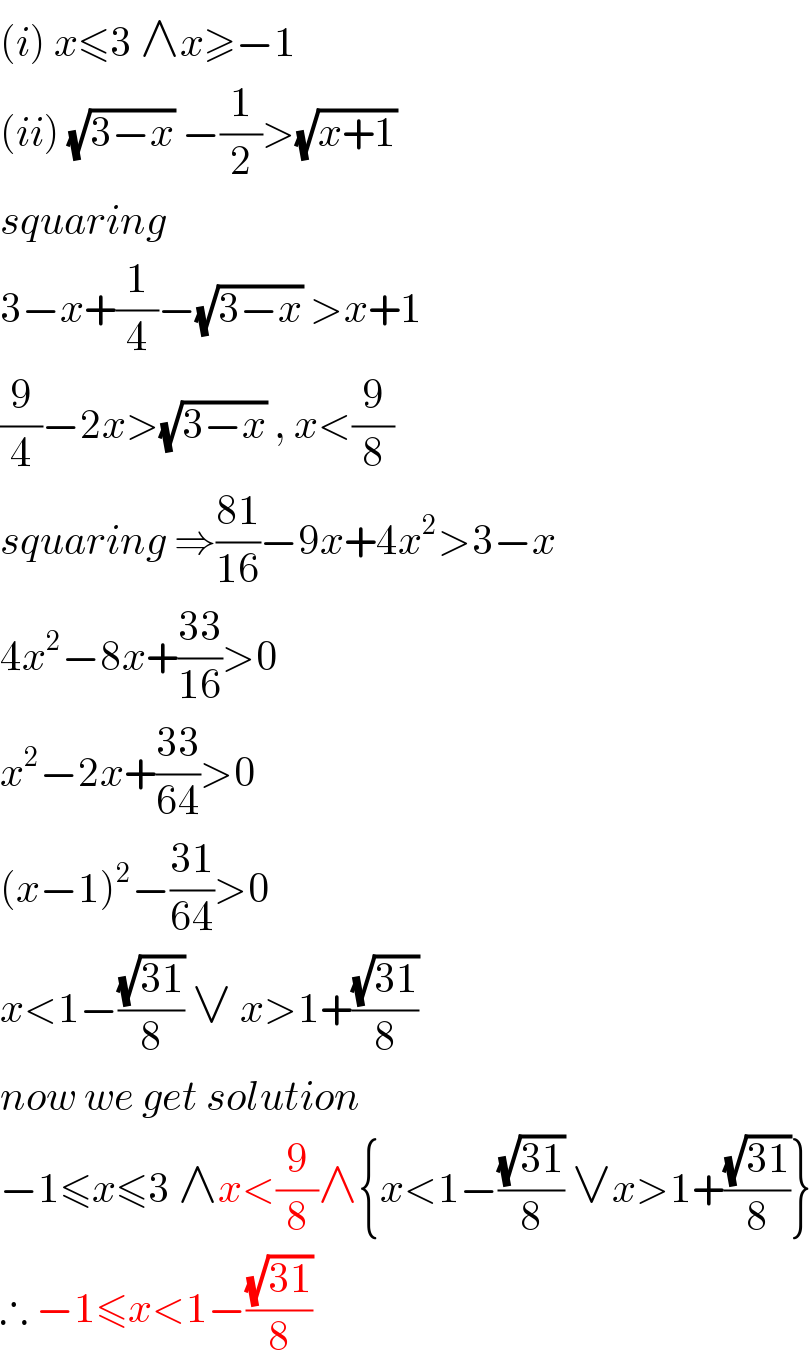
Commented by john santu last updated on 24/Jan/20

Commented by jagoll last updated on 24/Jan/20

Answered by key of knowledge last updated on 24/Jan/20
![3−x≥0∧x+1≥0⇒−1≤x≤3 (i) (√(3−x))>(√(x+1))+(1/2)⇒3−x>(1/4)+x+1+(√(x+1))⇒ (7/4)−2x>(√(x+1)) ((√(x+1))≥0⇒(7/4)−2x≥0⇒x≤(7/8) (ii)) 4x^2 −8x+((33)/(16))>0⇒((8+(√(31)))/8)<x∨x<((8−(√(31)))/8) (iii) i∩ii∩iii=[−1,((8−(√(31)))/8)]](https://www.tinkutara.com/question/Q79339.png)
