Question Number 61873 by lalitchand last updated on 10/Jun/19
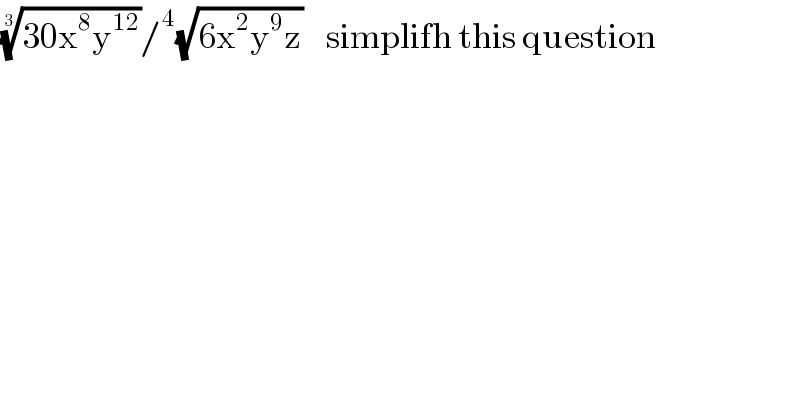
$$\sqrt[{\mathrm{3}}]{\mathrm{30x}^{\mathrm{8}} \mathrm{y}^{\mathrm{12}} }/^{\mathrm{4}} \sqrt{\mathrm{6x}^{\mathrm{2}} \mathrm{y}^{\mathrm{9}} \mathrm{z}}\:\:\:\:\mathrm{simplifh}\:\mathrm{this}\:\mathrm{question} \\ $$
Commented by MJS last updated on 10/Jun/19
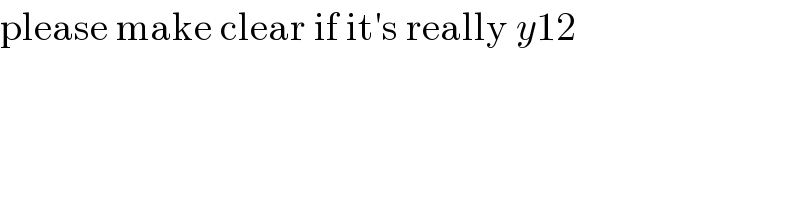
$$\mathrm{please}\:\mathrm{make}\:\mathrm{clear}\:\mathrm{if}\:\mathrm{it}'\mathrm{s}\:\mathrm{really}\:{y}\mathrm{12} \\ $$
Commented by lalitchand last updated on 10/Jun/19

$$\mathrm{yes}\:\mathrm{its}\:\mathrm{power} \\ $$
Answered by MJS last updated on 10/Jun/19
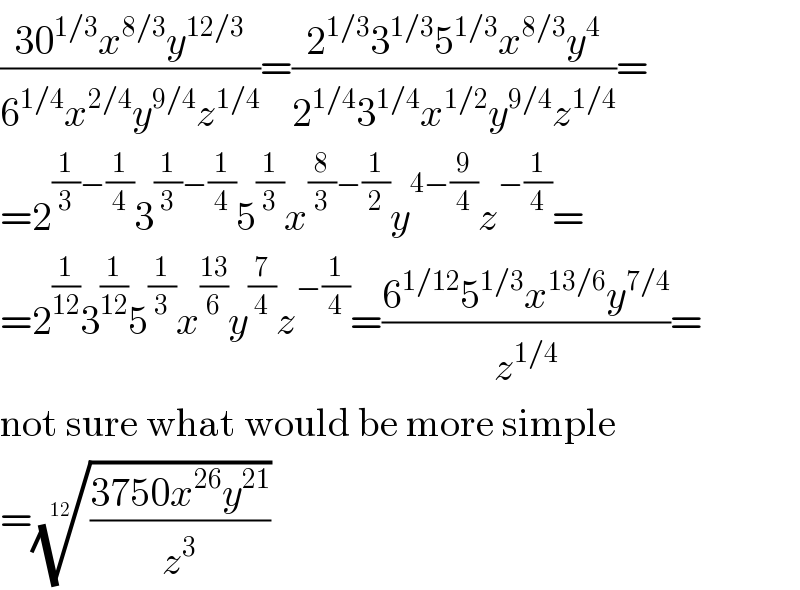
$$\frac{\mathrm{30}^{\mathrm{1}/\mathrm{3}} {x}^{\mathrm{8}/\mathrm{3}} {y}^{\mathrm{12}/\mathrm{3}} }{\mathrm{6}^{\mathrm{1}/\mathrm{4}} {x}^{\mathrm{2}/\mathrm{4}} {y}^{\mathrm{9}/\mathrm{4}} {z}^{\mathrm{1}/\mathrm{4}} }=\frac{\mathrm{2}^{\mathrm{1}/\mathrm{3}} \mathrm{3}^{\mathrm{1}/\mathrm{3}} \mathrm{5}^{\mathrm{1}/\mathrm{3}} {x}^{\mathrm{8}/\mathrm{3}} {y}^{\mathrm{4}} }{\mathrm{2}^{\mathrm{1}/\mathrm{4}} \mathrm{3}^{\mathrm{1}/\mathrm{4}} {x}^{\mathrm{1}/\mathrm{2}} {y}^{\mathrm{9}/\mathrm{4}} {z}^{\mathrm{1}/\mathrm{4}} }= \\ $$$$=\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{4}}} \mathrm{3}^{\frac{\mathrm{1}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{4}}} \mathrm{5}^{\frac{\mathrm{1}}{\mathrm{3}}} {x}^{\frac{\mathrm{8}}{\mathrm{3}}−\frac{\mathrm{1}}{\mathrm{2}}} {y}^{\mathrm{4}−\frac{\mathrm{9}}{\mathrm{4}}} {z}^{−\frac{\mathrm{1}}{\mathrm{4}}} = \\ $$$$=\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{12}}} \mathrm{3}^{\frac{\mathrm{1}}{\mathrm{12}}} \mathrm{5}^{\frac{\mathrm{1}}{\mathrm{3}}} {x}^{\frac{\mathrm{13}}{\mathrm{6}}} {y}^{\frac{\mathrm{7}}{\mathrm{4}}} {z}^{−\frac{\mathrm{1}}{\mathrm{4}}} =\frac{\mathrm{6}^{\mathrm{1}/\mathrm{12}} \mathrm{5}^{\mathrm{1}/\mathrm{3}} {x}^{\mathrm{13}/\mathrm{6}} {y}^{\mathrm{7}/\mathrm{4}} }{{z}^{\mathrm{1}/\mathrm{4}} }= \\ $$$$\mathrm{not}\:\mathrm{sure}\:\mathrm{what}\:\mathrm{would}\:\mathrm{be}\:\mathrm{more}\:\mathrm{simple} \\ $$$$=\sqrt[{\mathrm{12}}]{\frac{\mathrm{3750}{x}^{\mathrm{26}} {y}^{\mathrm{21}} }{{z}^{\mathrm{3}} }} \\ $$
Commented by lalitchand last updated on 10/Jun/19

$$\mathrm{this}\:\mathrm{is}\:\mathrm{what}\:\mathrm{i}\:\mathrm{got}\:\mathrm{but}\:\mathrm{kn}\:\mathrm{answer}\:\mathrm{sheet}\:\mathrm{it}\:\mathrm{was}\:\mathrm{different}\: \\ $$
Commented by MJS last updated on 10/Jun/19

$$\mathrm{then}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{sheet}\:\mathrm{is}\:\mathrm{wrong} \\ $$
