Menu Close

3cos-2-x-1-sin-5-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 148137 by Willson last updated on 25/Jul/21
∫ ((3cos^2 (x)+1)/(sin^5 (x)))dx = ???
$$\int\:\:\frac{\mathrm{3}{cos}^{\mathrm{2}} \left({x}\right)+\mathrm{1}}{{sin}^{\mathrm{5}} \left({x}\right)}{dx}\:=\:??? \\ $$
Answered by puissant last updated on 25/Jul/21
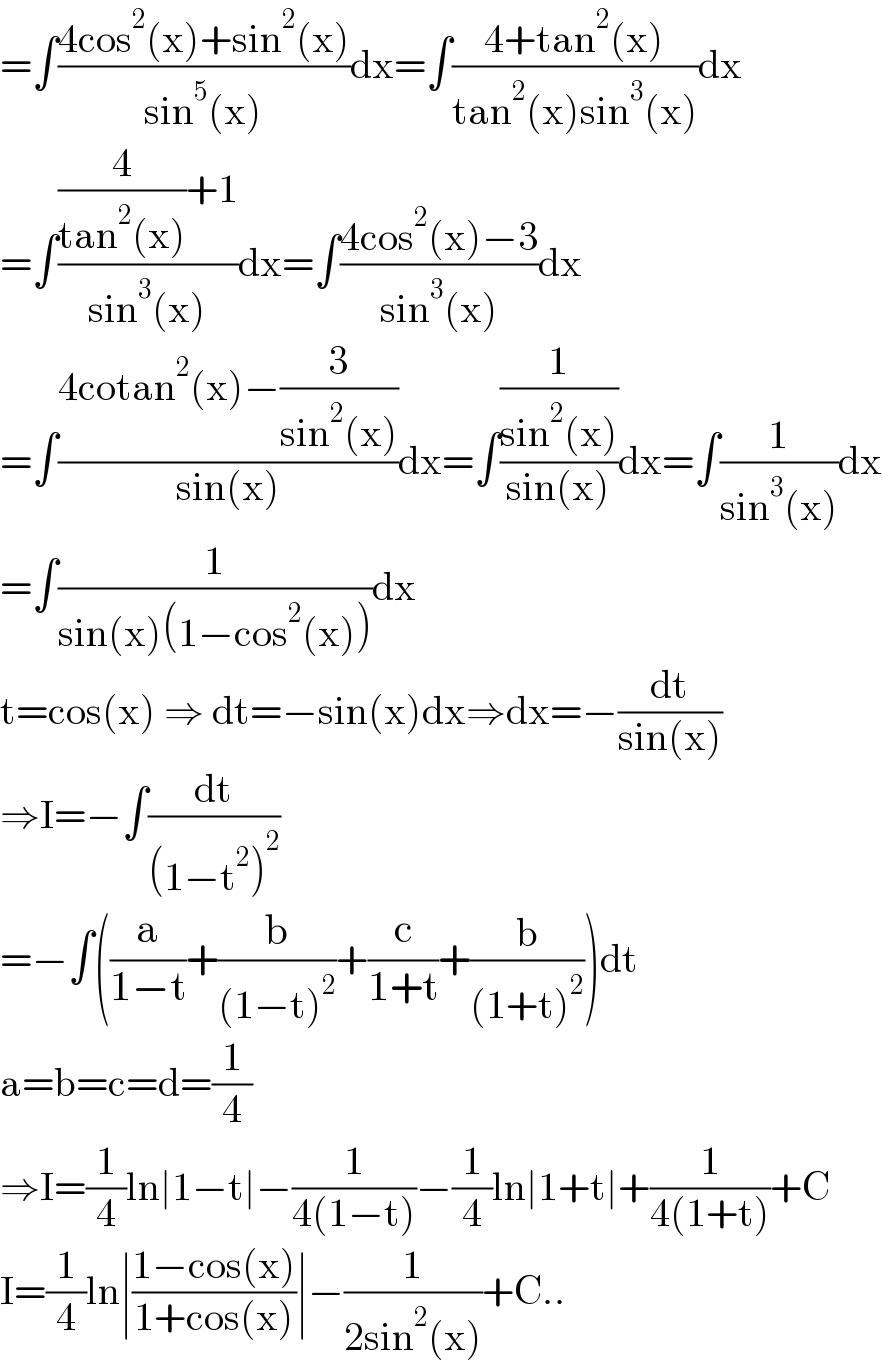
=∫((4cos^2 (x)+sin^2 (x))/(sin^5 (x)))dx=∫((4+tan^2 (x))/(tan^2 (x)sin^3 (x)))dx =∫(((4/(tan^2 (x)))+1)/(sin^3 (x)))dx=∫((4cos^2 (x)−3)/(sin^3 (x)))dx =∫((4cotan^2 (x)−(3/(sin^2 (x))))/(sin(x)))dx=∫((1/(sin^2 (x)))/(sin(x)))dx=∫(1/(sin^3 (x)))dx =∫(1/(sin(x)(1−cos^2 (x))))dx t=cos(x) ⇒ dt=−sin(x)dx⇒dx=−(dt/(sin(x))) ⇒I=−∫(dt/((1−t^2 )^2 )) =−∫((a/(1−t))+(b/((1−t)^2 ))+(c/(1+t))+(b/((1+t)^2 )))dt a=b=c=d=(1/4) ⇒I=(1/4)ln∣1−t∣−(1/(4(1−t)))−(1/4)ln∣1+t∣+(1/(4(1+t)))+C I=(1/4)ln∣((1−cos(x))/(1+cos(x)))∣−(1/(2sin^2 (x)))+C..
$$=\int\frac{\mathrm{4cos}^{\mathrm{2}} \left(\mathrm{x}\right)+\mathrm{sin}^{\mathrm{2}} \left(\mathrm{x}\right)}{\mathrm{sin}^{\mathrm{5}} \left(\mathrm{x}\right)}\mathrm{dx}=\int\frac{\mathrm{4}+\mathrm{tan}^{\mathrm{2}} \left(\mathrm{x}\right)}{\mathrm{tan}^{\mathrm{2}} \left(\mathrm{x}\right)\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx} \\ $$$$=\int\frac{\frac{\mathrm{4}}{\mathrm{tan}^{\mathrm{2}} \left(\mathrm{x}\right)}+\mathrm{1}}{\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx}=\int\frac{\mathrm{4cos}^{\mathrm{2}} \left(\mathrm{x}\right)−\mathrm{3}}{\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx} \\ $$$$=\int\frac{\mathrm{4cotan}^{\mathrm{2}} \left(\mathrm{x}\right)−\frac{\mathrm{3}}{\mathrm{sin}^{\mathrm{2}} \left(\mathrm{x}\right)}}{\mathrm{sin}\left(\mathrm{x}\right)}\mathrm{dx}=\int\frac{\frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{2}} \left(\mathrm{x}\right)}}{\mathrm{sin}\left(\mathrm{x}\right)}\mathrm{dx}=\int\frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{3}} \left(\mathrm{x}\right)}\mathrm{dx} \\ $$$$=\int\frac{\mathrm{1}}{\mathrm{sin}\left(\mathrm{x}\right)\left(\mathrm{1}−\mathrm{cos}^{\mathrm{2}} \left(\mathrm{x}\right)\right)}\mathrm{dx} \\ $$$$\mathrm{t}=\mathrm{cos}\left(\mathrm{x}\right)\:\Rightarrow\:\mathrm{dt}=−\mathrm{sin}\left(\mathrm{x}\right)\mathrm{dx}\Rightarrow\mathrm{dx}=−\frac{\mathrm{dt}}{\mathrm{sin}\left(\mathrm{x}\right)} \\ $$$$\Rightarrow\mathrm{I}=−\int\frac{\mathrm{dt}}{\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$$=−\int\left(\frac{\mathrm{a}}{\mathrm{1}−\mathrm{t}}+\frac{\mathrm{b}}{\left(\mathrm{1}−\mathrm{t}\right)^{\mathrm{2}} }+\frac{\mathrm{c}}{\mathrm{1}+\mathrm{t}}+\frac{\mathrm{b}}{\left(\mathrm{1}+\mathrm{t}\right)^{\mathrm{2}} }\right)\mathrm{dt} \\ $$$$\mathrm{a}=\mathrm{b}=\mathrm{c}=\mathrm{d}=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{I}=\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\mathrm{1}−\mathrm{t}\mid−\frac{\mathrm{1}}{\mathrm{4}\left(\mathrm{1}−\mathrm{t}\right)}−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\mathrm{1}+\mathrm{t}\mid+\frac{\mathrm{1}}{\mathrm{4}\left(\mathrm{1}+\mathrm{t}\right)}+\mathrm{C} \\ $$$$\mathrm{I}=\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{x}\right)}{\mathrm{1}+\mathrm{cos}\left(\mathrm{x}\right)}\mid−\frac{\mathrm{1}}{\mathrm{2sin}^{\mathrm{2}} \left(\mathrm{x}\right)}+\mathrm{C}.. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *