Menu Close

3x-2-11x-6-x-2-1-x-5-dx-

Tinku Tara 0 Comments
Pin




Question Number 101756 by bramlex last updated on 04/Jul/20
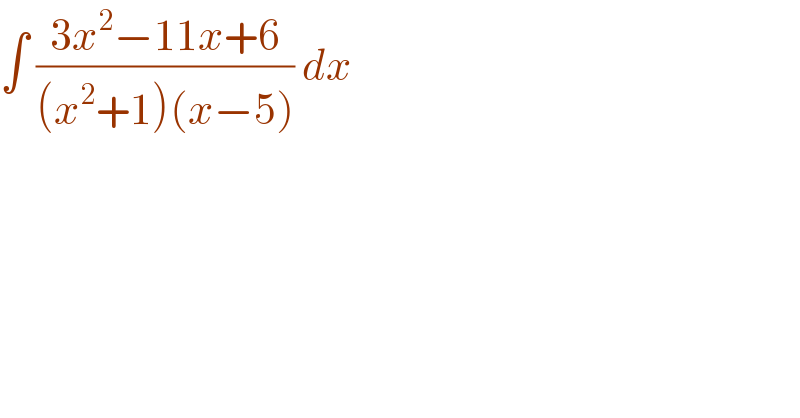
∫ ((3x^2 −11x+6)/((x^2 +1)(x−5))) dx
$$\int\:\frac{\mathrm{3}{x}^{\mathrm{2}} −\mathrm{11}{x}+\mathrm{6}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}−\mathrm{5}\right)}\:{dx}\: \\ $$
Answered by john santu last updated on 04/Jul/20
3x^2 −11x+6 = (x−5)(ax+b)+c(x^2 +1) x=0 ; 6 = −5b+c x=5 ; 26 = 26c ⇒c=1 ∧b = −1 x=−1; 20= (−6)(−a−1)+2 3 = a+1 ⇒a = 2 ∫ ((2x−1)/(x^2 +1)) dx + ∫ (1/(x−5)) dx = ∫ ((d(x^2 +1))/(x^2 +1)) − arctan (x) + ln∣x−5∣ + c = ln(x^2 +1)+ ln(x−5)−arctan (x) +c = ln((x^2 +1)(x−5))−arctan (x)+ c (JS ⊛ )
$$\mathrm{3}{x}^{\mathrm{2}} −\mathrm{11}{x}+\mathrm{6}\:=\:\left({x}−\mathrm{5}\right)\left({ax}+{b}\right)+{c}\left({x}^{\mathrm{2}} +\mathrm{1}\right) \\ $$$${x}=\mathrm{0}\:;\:\mathrm{6}\:=\:−\mathrm{5}{b}+{c} \\ $$$${x}=\mathrm{5}\:;\:\mathrm{26}\:=\:\mathrm{26}{c}\:\Rightarrow{c}=\mathrm{1}\:\wedge{b}\:=\:−\mathrm{1} \\ $$$${x}=−\mathrm{1};\:\mathrm{20}=\:\left(−\mathrm{6}\right)\left(−{a}−\mathrm{1}\right)+\mathrm{2} \\ $$$$\mathrm{3}\:=\:{a}+\mathrm{1}\:\Rightarrow{a}\:=\:\mathrm{2} \\ $$$$\int\:\frac{\mathrm{2}{x}−\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:{dx}\:+\:\int\:\frac{\mathrm{1}}{{x}−\mathrm{5}}\:{dx}\:= \\ $$$$\int\:\frac{{d}\left({x}^{\mathrm{2}} +\mathrm{1}\right)}{{x}^{\mathrm{2}} +\mathrm{1}}\:−\:\mathrm{arctan}\:\left({x}\right)\:+\:\mathrm{ln}\mid{x}−\mathrm{5}\mid\:+\:\mathrm{c} \\ $$$$=\:\mathrm{ln}\left({x}^{\mathrm{2}} +\mathrm{1}\right)+\:\mathrm{ln}\left({x}−\mathrm{5}\right)−\mathrm{arctan}\:\left({x}\right)\:+{c}\: \\ $$$$=\:\mathrm{ln}\left(\left({x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}−\mathrm{5}\right)\right)−\mathrm{arctan}\:\left({x}\right)+\:{c} \\ $$$$\left({J}\mathrm{S}\:\circledast\:\right) \\ $$
Answered by Dwaipayan Shikari last updated on 04/Jul/20
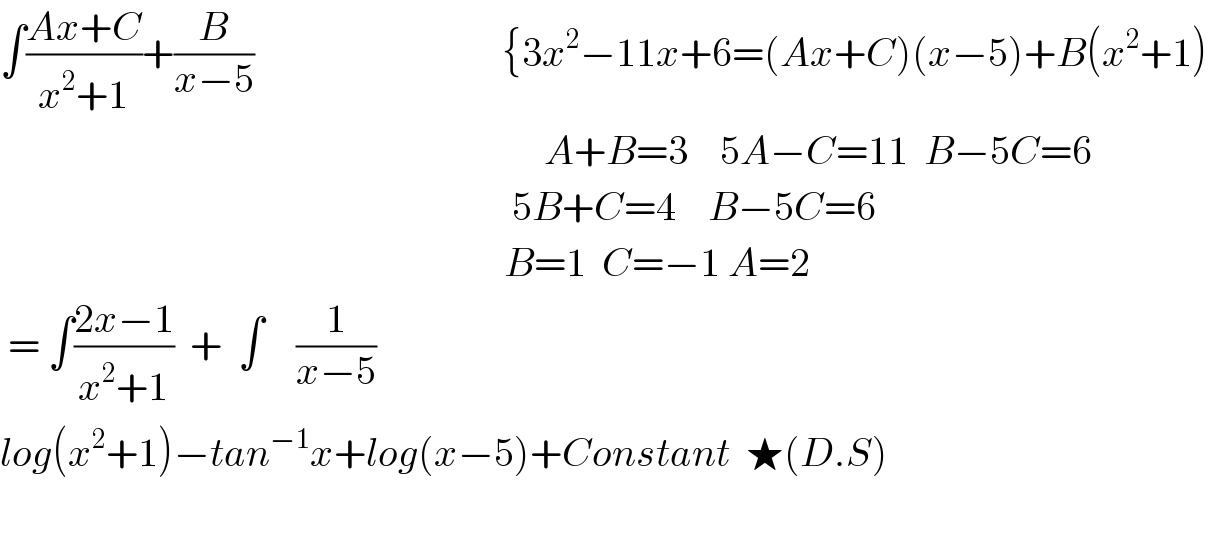
∫((Ax+C)/(x^2 +1))+(B/(x−5)) {3x^2 −11x+6=(Ax+C)(x−5)+B(x^2 +1) A+B=3 5A−C=11 B−5C=6 5B+C=4 B−5C=6 B=1 C=−1 A=2 = ∫((2x−1)/(x^2 +1)) + ∫ (1/(x−5)) log(x^2 +1)−tan^(−1) x+log(x−5)+Constant ★(D.S)
$$\int\frac{{Ax}+{C}}{{x}^{\mathrm{2}} +\mathrm{1}}+\frac{{B}}{{x}−\mathrm{5}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left\{\mathrm{3}{x}^{\mathrm{2}} −\mathrm{11}{x}+\mathrm{6}=\left({Ax}+{C}\right)\left({x}−\mathrm{5}\right)+{B}\left({x}^{\mathrm{2}} +\mathrm{1}\right)\right. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{A}+{B}=\mathrm{3}\:\:\:\:\mathrm{5}{A}−{C}=\mathrm{11}\:\:{B}−\mathrm{5}{C}=\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{5}{B}+{C}=\mathrm{4}\:\:\:\:{B}−\mathrm{5}{C}=\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{B}=\mathrm{1}\:\:{C}=−\mathrm{1}\:{A}=\mathrm{2} \\ $$$$\:=\:\int\frac{\mathrm{2}{x}−\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:\:+\:\:\int\:\:\:\:\frac{\mathrm{1}}{{x}−\mathrm{5}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$${log}\left({x}^{\mathrm{2}} +\mathrm{1}\right)−{tan}^{−\mathrm{1}} {x}+{log}\left({x}−\mathrm{5}\right)+{Constant}\:\:\bigstar\left({D}.{S}\right) \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *