Question Number 152271 by peter frank last updated on 27/Aug/21
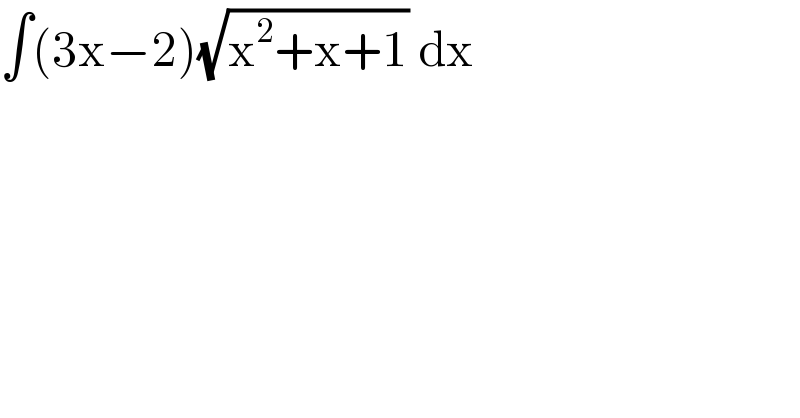
$$\int\left(\mathrm{3x}−\mathrm{2}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\:\mathrm{dx} \\ $$
Answered by qaz last updated on 27/Aug/21
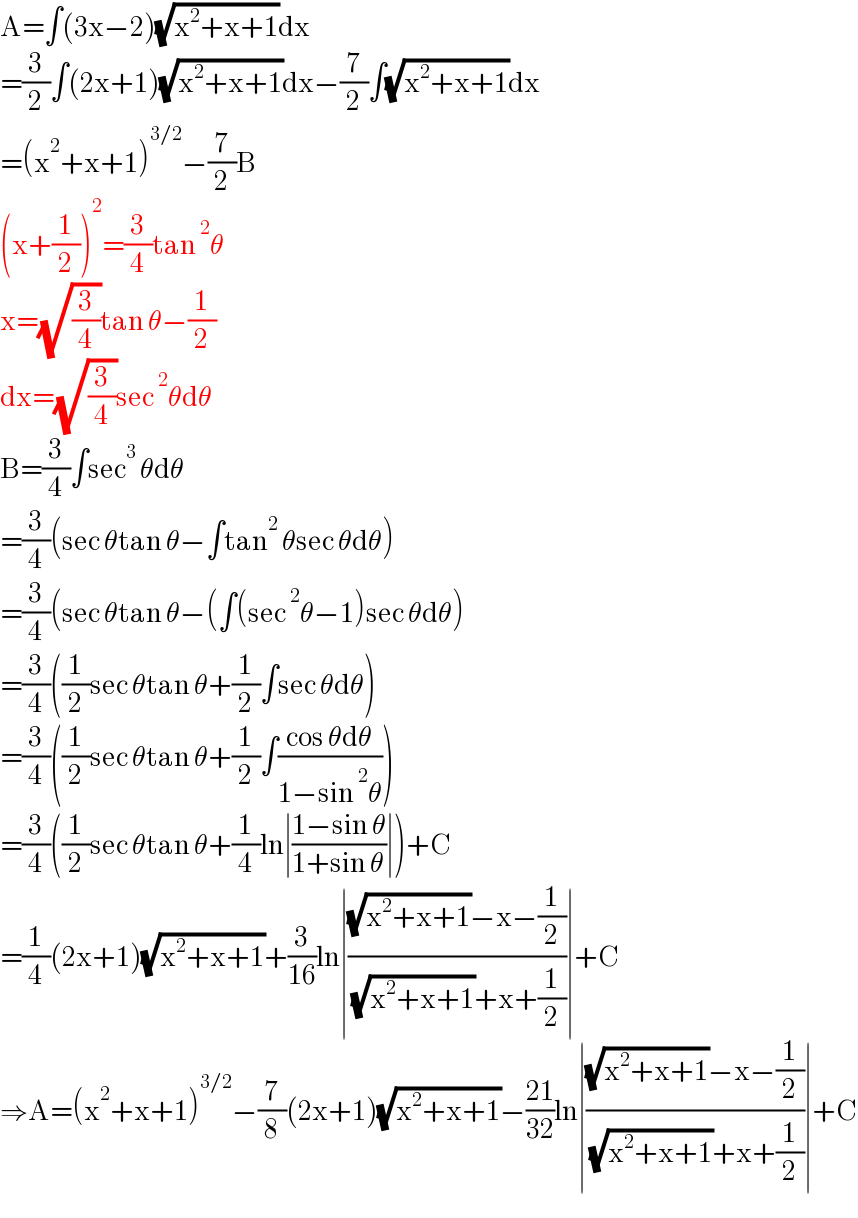
$$\mathrm{A}=\int\left(\mathrm{3x}−\mathrm{2}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}\int\left(\mathrm{2x}+\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\mathrm{dx}−\frac{\mathrm{7}}{\mathrm{2}}\int\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$$$=\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}/\mathrm{2}} −\frac{\mathrm{7}}{\mathrm{2}}\mathrm{B} \\ $$$$\left(\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} =\frac{\mathrm{3}}{\mathrm{4}}\mathrm{tan}\:^{\mathrm{2}} \theta \\ $$$$\mathrm{x}=\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}\mathrm{tan}\:\theta−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{dx}=\sqrt{\frac{\mathrm{3}}{\mathrm{4}}}\mathrm{sec}\:^{\mathrm{2}} \theta\mathrm{d}\theta \\ $$$$\mathrm{B}=\frac{\mathrm{3}}{\mathrm{4}}\int\mathrm{sec}^{\mathrm{3}} \:\theta\mathrm{d}\theta \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\left(\mathrm{sec}\:\theta\mathrm{tan}\:\theta−\int\mathrm{tan}^{\mathrm{2}} \:\theta\mathrm{sec}\:\theta\mathrm{d}\theta\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\left(\mathrm{sec}\:\theta\mathrm{tan}\:\theta−\left(\int\left(\mathrm{sec}\:^{\mathrm{2}} \theta−\mathrm{1}\right)\mathrm{sec}\:\theta\mathrm{d}\theta\right)\right. \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sec}\:\theta\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{sec}\:\theta\mathrm{d}\theta\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sec}\:\theta\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{cos}\:\theta\mathrm{d}\theta}{\mathrm{1}−\mathrm{sin}\:^{\mathrm{2}} \theta}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{4}}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sec}\:\theta\mathrm{tan}\:\theta+\frac{\mathrm{1}}{\mathrm{4}}\mathrm{ln}\mid\frac{\mathrm{1}−\mathrm{sin}\:\theta}{\mathrm{1}+\mathrm{sin}\:\theta}\mid\right)+\mathrm{C} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{2x}+\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}+\frac{\mathrm{3}}{\mathrm{16}}\mathrm{ln}\mid\frac{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}−\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}+\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}}\mid+\mathrm{C} \\ $$$$\Rightarrow\mathrm{A}=\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}/\mathrm{2}} −\frac{\mathrm{7}}{\mathrm{8}}\left(\mathrm{2x}+\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}−\frac{\mathrm{21}}{\mathrm{32}}\mathrm{ln}\mid\frac{\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}−\mathrm{x}−\frac{\mathrm{1}}{\mathrm{2}}}{\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}+\mathrm{x}+\frac{\mathrm{1}}{\mathrm{2}}}\mid+\mathrm{C} \\ $$
