3x-3-4x-3-0-solve-this-problem-

Question Number 108473 by subhankar10 last updated on 17/Aug/20

{3 x}^{3} + 4 x - 3 = 0

solve this problem .

Answered by Sarah85 last updated on 17/Aug/20

x^{3} + \frac{4}{3} x - 1 = 0

p = \frac{4}{3} \land q = - 1 \Rightarrow D = {(\frac{p}{3})}^{3} + {(\frac{q}{2})}^{2} > 0 \Rightarrow Cardano

u = \sqrt[3]{- \frac{q}{2} + \sqrt{\frac{p^{3}}{27} + \frac{q^{2}}{4}}} \land v = - \sqrt[3]{\frac{q}{2} + \sqrt{\frac{p^{3}}{27} + \frac{q^{2}}{4}}}

x_{1} = u + v

x_{2} = ω u + ω^{2} v

x_{3} = ω^{2} u + ω v

where ω = - \frac{1}{2} + \frac{\sqrt{3}}{2} i

now put p = \frac{4}{3} \land q = - 1

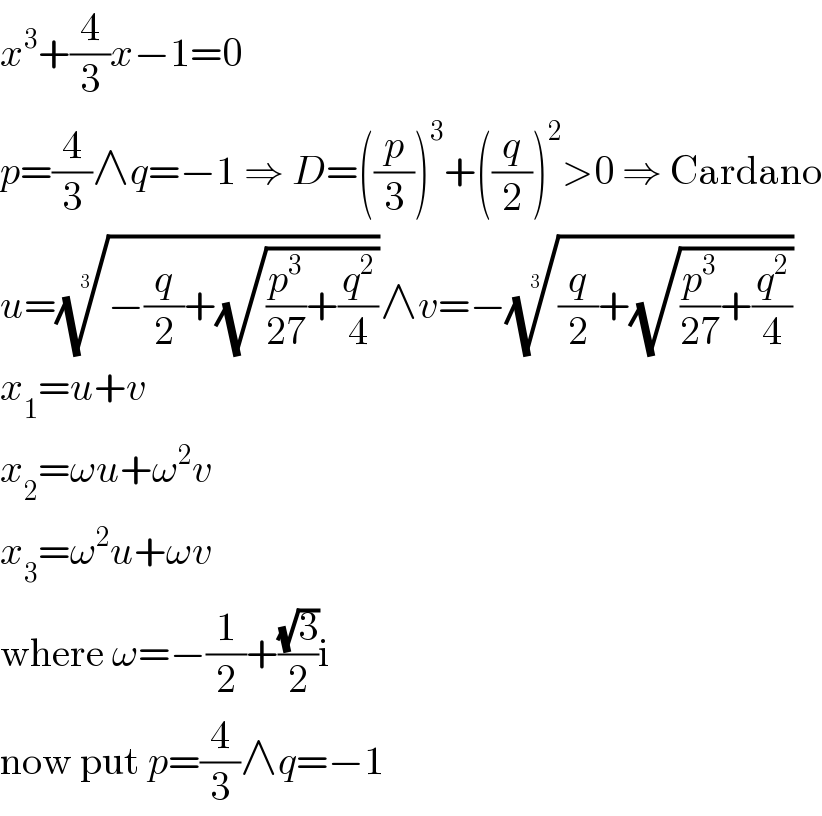
Answered by 1549442205PVT last updated on 17/Aug/20

{3 x}^{3} + 4 x - 3 = 0 (1)

Put x = \frac{2}{3} y then (1) \Leftrightarrow \frac{8}{9} y^{3} + \frac{8}{3} y - 3 = 0

\Leftrightarrow y^{3} + 3 y - \frac{27}{8} = 0 (2) . Put y = z - \frac{1}{z}

(2) \Leftrightarrow z^{3} - \frac{1}{z^{3}} - 3 (z - \frac{1}{z}) + 3 (z - \frac{1}{z}) - \frac{27}{8} = 0

\Leftrightarrow z^{3} - \frac{1}{z^{3}} - \frac{27}{8} = 0 . Put z^{3} = t we get

t - \frac{1}{t} - \frac{27}{8} = 0 \Leftrightarrow {8 t}^{2} - 27 t - 8 = 0

Δ = 27^{2} + 4 . 8^{2} = 729 + 256 = 985

\Rightarrow z^{3} = t = \frac{27 \pm \sqrt{985}}{16} \Rightarrow z =^{3} \sqrt{\frac{27 \pm \sqrt{985}}{16}}

\Rightarrow \frac{1}{z} =^{3} \sqrt{\frac{16}{27 \pm \sqrt{985}}}

i) For z =^{3} \sqrt{\frac{27 + \sqrt{985}}{16}} \Rightarrow \frac{1}{z} =^{3} \sqrt{\frac{16}{27 + \sqrt{985}}}

we get x_{1} = \frac{2}{3} y = \frac{2}{3} (z - \frac{1}{z}) =

= \frac{2}{3} [^{3} \sqrt{\frac{27 + \sqrt{985}}{16}} -^{3} \sqrt{\frac{16}{27 + \sqrt{985}}}]

ii) For z =^{3} \sqrt{\frac{27 - \sqrt{985}}{16}} \Rightarrow \frac{1}{z} =^{3} \sqrt{\frac{16}{27 - \sqrt{985}}}

z - \frac{1}{z} = [3 \sqrt{\frac{27 - \sqrt{985}}{16}} -^{3} \sqrt{\frac{16}{27 - \sqrt{985}}}]

= [^{3} \sqrt{\frac{- 256}{16 (27 + \sqrt{985}}} -^{3} \sqrt{\frac{16 (27 + \sqrt{985})}{- 256}}

=^{3} \sqrt{\frac{- 16}{27 + \sqrt{985}}} -^{3} \sqrt{\frac{27 + \sqrt{985}}{- 16}}

=^{3} \sqrt{\frac{27 + \sqrt{985}}{16}} -^{3} \sqrt{\frac{16}{27 + \sqrt{985}}}

we get x_{2} = \frac{2}{3} y = \frac{2}{3} (z - \frac{1}{z}) = x_{1}

Thus, the given equation has unique

solution x = \frac{2}{3} [3 \sqrt{\frac{27 + \sqrt{985}}{16}} -^{3} \sqrt{\frac{16}{27 + \sqrt{985}}}]

Commented by Sarah85 last updated on 17/Aug/20

{we}^{'} ve got {Cardano}^{'} s solution formula and

for the cases where D < 0 {there}^{'} s a trigonometric

solution formula . no need to solve each single

case like this .

and btw a 3 rd degree polynomial a l w a y s has

three solutions

Commented by 1549442205PVT last updated on 17/Aug/20

The above solution only mention to

real roots . If you want to find two other

complex roots then they are in the cubic

root of z^{3} = \frac{27 \pm \sqrt{985}}{16} because the cubic

root of an arbitrary number has always

three different values in field of

complex number