Question Number 57469 by olalekan2 last updated on 05/Apr/19

$$\frac{\mathrm{4}{tan}\mathrm{75}}{\mathrm{1}−{tan}^{\mathrm{2}} \mathrm{75}}=\frac{\mathrm{1}}{{cos}\mathrm{150}}\: \\ $$$${find}\:{tan}\mathrm{75}\:{in}\:{surd}\:{form} \\ $$
Commented by kaivan.ahmadi last updated on 05/Apr/19
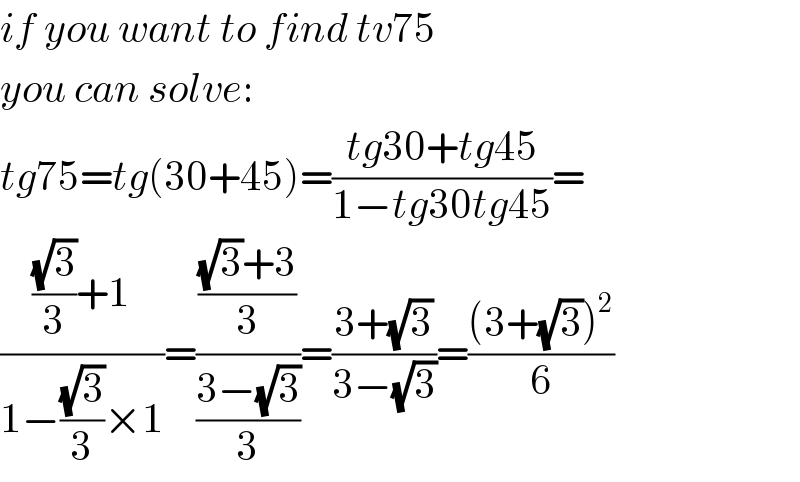
$${if}\:{you}\:{want}\:{to}\:{find}\:{tv}\mathrm{75} \\ $$$${you}\:{can}\:{solve}: \\ $$$${tg}\mathrm{75}={tg}\left(\mathrm{30}+\mathrm{45}\right)=\frac{{tg}\mathrm{30}+{tg}\mathrm{45}}{\mathrm{1}−{tg}\mathrm{30}{tg}\mathrm{45}}= \\ $$$$\frac{\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}+\mathrm{1}}{\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{3}}×\mathrm{1}}=\frac{\frac{\sqrt{\mathrm{3}}+\mathrm{3}}{\mathrm{3}}}{\frac{\mathrm{3}−\sqrt{\mathrm{3}}}{\mathrm{3}}}=\frac{\mathrm{3}+\sqrt{\mathrm{3}}}{\mathrm{3}−\sqrt{\mathrm{3}}}=\frac{\left(\mathrm{3}+\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }{\mathrm{6}} \\ $$
Commented by Kunal12588 last updated on 05/Apr/19
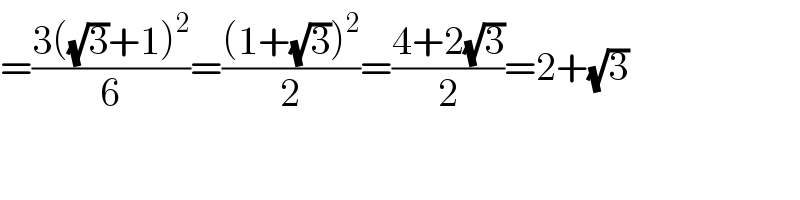
$$=\frac{\mathrm{3}\left(\sqrt{\mathrm{3}}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{6}}=\frac{\left(\mathrm{1}+\sqrt{\mathrm{3}}\right)^{\mathrm{2}} }{\mathrm{2}}=\frac{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{2}+\sqrt{\mathrm{3}} \\ $$
Commented by olalekan2 last updated on 22/Apr/19

$${thankz}\:{sir} \\ $$
