8-1-4-2-1-8-1-4-2-1-8-1-4-2-1-

Question Number 96505 by bemath last updated on 02/Jun/20

\frac{\sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} + 1}}}{\sqrt{\sqrt[4]{8} + \sqrt{\sqrt{2} - 1}} - \sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} - 1}}} ?

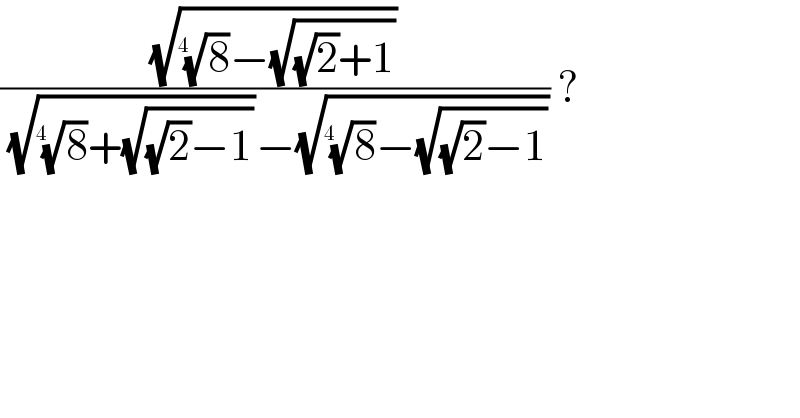
Commented by bemath last updated on 02/Jun/20

yes sir . you are right

Commented by bobhans last updated on 02/Jun/20

maybe it \frac{\sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} + 1}}}{\sqrt{\sqrt[4]{8} + \sqrt{\sqrt{2} - 1}} - \sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} - 1}}}

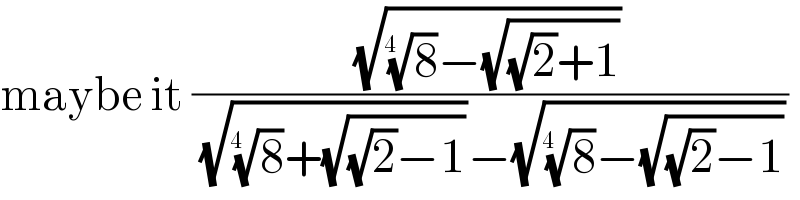
Answered by bobhans last updated on 02/Jun/20

w = \frac{\sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} + 1}}}{\sqrt{\sqrt[4]{8} + \sqrt{\sqrt{2} - 1}} - \sqrt{\sqrt[4]{8} - \sqrt{\sqrt{2} - 1}}}

w^{2} = \frac{\sqrt[4]{8} - \sqrt{\sqrt{2} - 1}}{2 \sqrt[4]{8} - 2 \sqrt{\sqrt[4]{64} - (\sqrt{2} - 1)}}

w^{2} = \frac{\sqrt[4]{8} - \sqrt{\sqrt{2} - 1}}{2 (\sqrt[4]{8} - \sqrt{\sqrt{2} - 1})} = \frac{1}{2}

w = \frac{1}{\sqrt{2}}

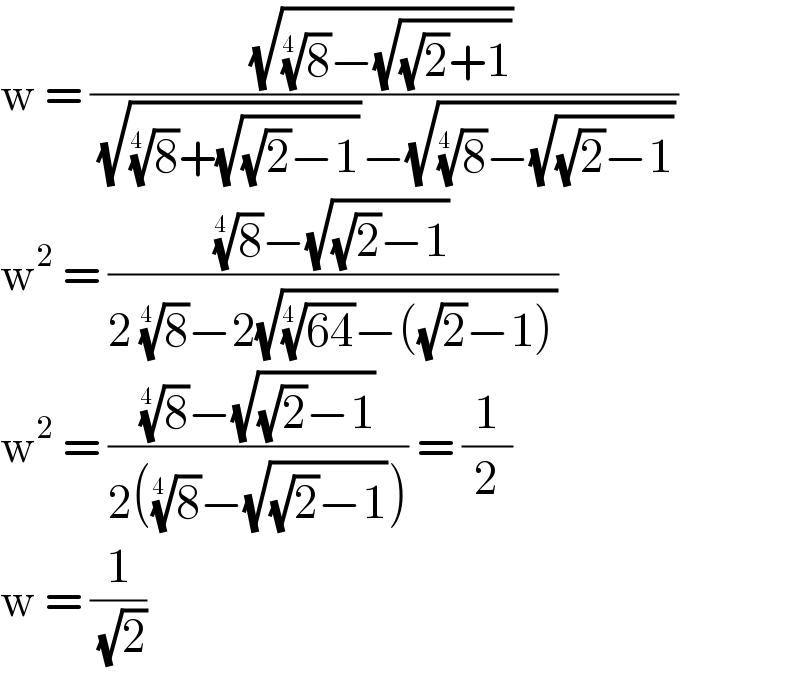
Answered by 1549442205 last updated on 02/Jun/20

Putting^{4} \sqrt{8} = \sqrt{2 \sqrt{2}} = a, \sqrt{\sqrt{2} - 1} = b

we have a^{2} - b^{2} = \sqrt{2} + 1 . Hence,

A = \frac{\sqrt{a - \sqrt{a^{2} - b^{2}}}}{\sqrt{a + b} - \sqrt{a - b}} = \frac{\sqrt{a - \sqrt{a^{2} - b^{2}}}}{{\sqrt{(\sqrt{a + b} - \sqrt{a - b}})}^{2}}

= \frac{\sqrt{a - \sqrt{a^{2} - b^{2}}}}{\sqrt{2 a - 2 \sqrt{a^{2} - b^{2}}}} = \frac{\sqrt{a - \sqrt{a^{2} - b^{2}}}}{\sqrt{2} (\sqrt{a - \sqrt{a^{2} - b^{2}}}}

= \frac{1}{\sqrt{2}} . Thus, A = \frac{1}{\sqrt{2}}