Menu Close

9-4-5-1-3-9-4-5-1-3-

Tinku Tara 0 Comments
Pin




Question Number 170478 by balirampatel last updated on 24/May/22
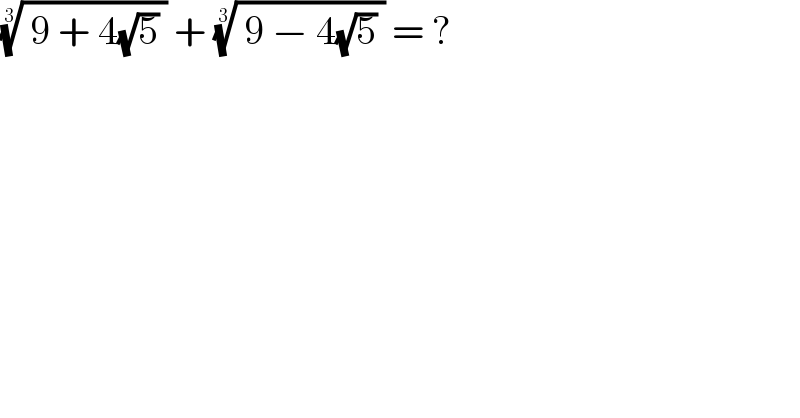
(( 9 + 4(√5) ))^(1/3) + (( 9 − 4(√5) ))^(1/3) = ?
$$\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:+\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:=\:? \\ $$
Answered by Rasheed.Sindhi last updated on 24/May/22
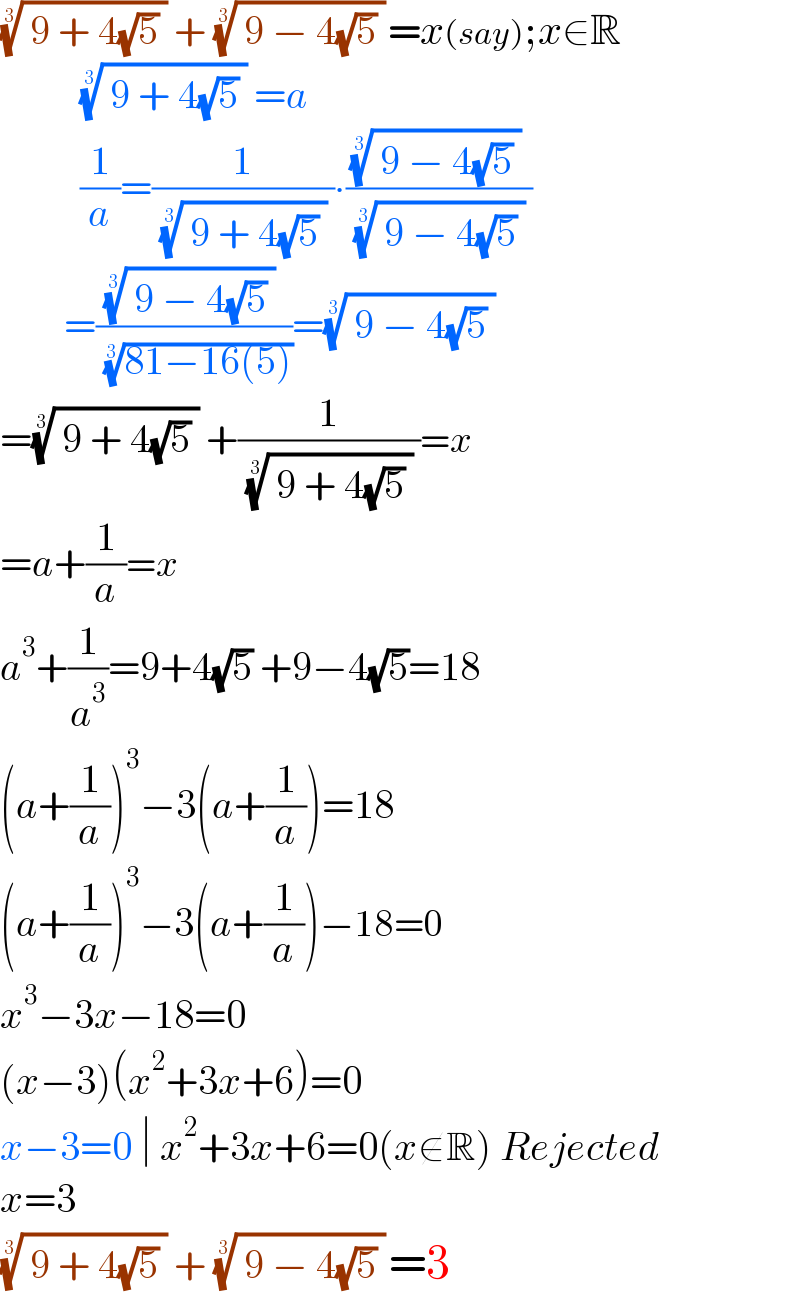
(( 9 + 4(√5) ))^(1/3) + (( 9 − 4(√5) ))^(1/3) =x(say);x∈R (( 9 + 4(√5) ))^(1/3) =a (1/a)=(1/( (( 9 + 4(√5) ))^(1/3) ))∙(((( 9 − 4(√5) ))^(1/3) )/( (( 9 − 4(√5) ))^(1/3) )) =(((( 9 − 4(√5) ))^(1/3) )/( ((81−16(5)))^(1/3) ))=(( 9 − 4(√5) ))^(1/3) =(( 9 + 4(√5) ))^(1/3) +(1/( (( 9 + 4(√5) ))^(1/3) ))=x =a+(1/a)=x a^3 +(1/a^3 )=9+4(√5) +9−4(√5)=18 (a+(1/a))^3 −3(a+(1/a))=18 (a+(1/a))^3 −3(a+(1/a))−18=0 x^3 −3x−18=0 (x−3)(x^2 +3x+6)=0 x−3=0 ∣ x^2 +3x+6=0(x∉R) Rejected x=3 (( 9 + 4(√5) ))^(1/3) + (( 9 − 4(√5) ))^(1/3) =3
$$\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:+\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:={x}\left({say}\right);{x}\in\mathbb{R} \\ $$$$\:\:\:\:\:\:\:\:\:\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:={a} \\ $$$$\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{{a}}=\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:}\centerdot\frac{\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:}{\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:} \\ $$$$\:\:\:\:\:\:\:\:=\frac{\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:}{\:\sqrt[{\mathrm{3}}]{\mathrm{81}−\mathrm{16}\left(\mathrm{5}\right)}}=\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\: \\ $$$$=\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:+\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:}={x} \\ $$$$={a}+\frac{\mathrm{1}}{{a}}={x}\: \\ $$$${a}^{\mathrm{3}} +\frac{\mathrm{1}}{{a}^{\mathrm{3}} }=\mathrm{9}+\mathrm{4}\sqrt{\mathrm{5}}\:+\mathrm{9}−\mathrm{4}\sqrt{\mathrm{5}}=\mathrm{18} \\ $$$$\left({a}+\frac{\mathrm{1}}{{a}}\right)^{\mathrm{3}} −\mathrm{3}\left({a}+\frac{\mathrm{1}}{{a}}\right)=\mathrm{18} \\ $$$$\left({a}+\frac{\mathrm{1}}{{a}}\right)^{\mathrm{3}} −\mathrm{3}\left({a}+\frac{\mathrm{1}}{{a}}\right)−\mathrm{18}=\mathrm{0} \\ $$$${x}^{\mathrm{3}} −\mathrm{3}{x}−\mathrm{18}=\mathrm{0} \\ $$$$\left({x}−\mathrm{3}\right)\left({x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{6}\right)=\mathrm{0} \\ $$$${x}−\mathrm{3}=\mathrm{0}\:\mid\:{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{6}=\mathrm{0}\left({x}\notin\mathbb{R}\right)\:{Rejected} \\ $$$${x}=\mathrm{3} \\ $$$$\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:+\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:+\:\sqrt[{\mathrm{3}}]{\:\mathrm{9}\:−\:\mathrm{4}\sqrt{\mathrm{5}}\:}\:=\mathrm{3} \\ $$
Answered by mr W last updated on 24/May/22
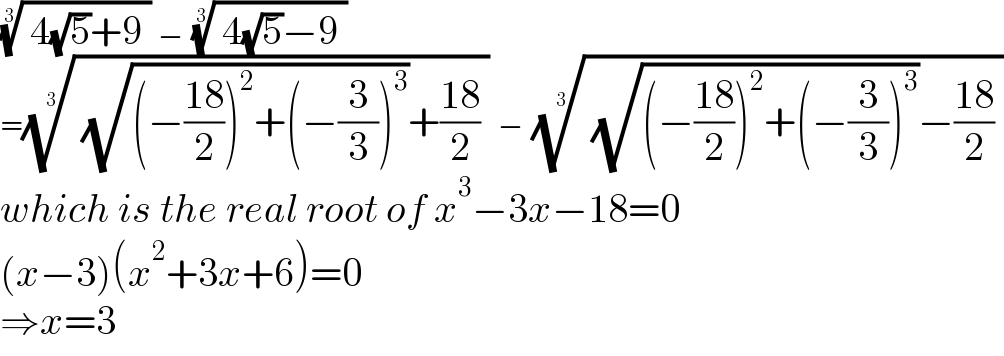
(( 4(√5)+9 ))^(1/3) − (( 4(√5)−9 ))^(1/3) =(( (√((−((18)/2))^2 +(−(3/3))^3 ))+((18)/2) ))^(1/3) − (( (√((−((18)/2))^2 +(−(3/3))^3 ))−((18)/2) ))^(1/3) which is the real root of x^3 −3x−18=0 (x−3)(x^2 +3x+6)=0 ⇒x=3
$$\sqrt[{\mathrm{3}}]{\:\mathrm{4}\sqrt{\mathrm{5}}+\mathrm{9}\:}\:−\:\sqrt[{\mathrm{3}}]{\:\mathrm{4}\sqrt{\mathrm{5}}−\mathrm{9}\:} \\ $$$$=\sqrt[{\mathrm{3}}]{\:\sqrt{\left(−\frac{\mathrm{18}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(−\frac{\mathrm{3}}{\mathrm{3}}\right)^{\mathrm{3}} }+\frac{\mathrm{18}}{\mathrm{2}}\:}\:−\:\sqrt[{\mathrm{3}}]{\:\sqrt{\left(−\frac{\mathrm{18}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(−\frac{\mathrm{3}}{\mathrm{3}}\right)^{\mathrm{3}} }−\frac{\mathrm{18}}{\mathrm{2}}\:} \\ $$$${which}\:{is}\:{the}\:{real}\:{root}\:{of}\:{x}^{\mathrm{3}} −\mathrm{3}{x}−\mathrm{18}=\mathrm{0} \\ $$$$\left({x}−\mathrm{3}\right)\left({x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{6}\right)=\mathrm{0} \\ $$$$\Rightarrow{x}=\mathrm{3} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *