A-0-0-B-2-4-and-C-6-14-if-the-triangle-has-vertices-find-the-lenght-of-the-median-drawn-from-the-vertx-C-

Question Number 148550 by mathdanisur last updated on 29/Jul/21

A (0; 0); B (- 2; 4) a n d C (- 6; 14)

i f t h e t r i a n g l e h a s v e r t i c e s, f i n d t h e

l e n g h t o f t h e m e d i a n d r a w n f r o m

t h e v e r t x C

Commented by MJS_new last updated on 29/Jul/21

first show us a triangle which has n o vertices

Commented by Ar Brandon last updated on 29/Jul/21

��

Answered by Rasheed.Sindhi last updated on 29/Jul/21

M i d p o i n t o f A B = M = (\frac{- 2 + 0}{2}, \frac{4 + 0}{2}) = (- 1, 2)

T h e m e d i a n t h r o u g h C

C M = \sqrt{{(- 6 - (- 1))}^{2} + (14 - 2)}

= \sqrt{25 + 144} = \sqrt{169} = 13

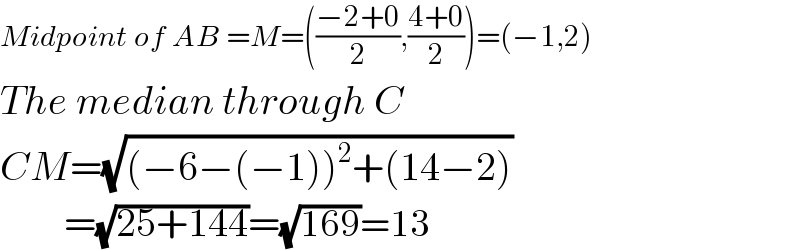
Commented by mathdanisur last updated on 29/Jul/21

T h a n k y o u S e r, c o o l