a-1-1-a-2-1-and-a-n-a-n-1-2a-n-2-Find-a-n-

Question Number 164956 by Jamshidbek last updated on 24/Jan/22

a_{1} = 1 a_{2} = - 1 and a_{n} = - a_{n - 1} - {2 a}_{n - 2}

Find a_{n}

Answered by mr W last updated on 24/Jan/22

1, - 1, 0, 1, - 1, 0, 1, - 1, 0, \dots

t h r o u g h “ {o b s e r v a t i o n}^{″} :

\Rightarrow a_{3 n} = 0

\Rightarrow a_{3 n + 1} = 1

\Rightarrow a_{3 n + 2} = - 1

g e n e r a l m e t h o d :

r^{2} + r + 2 = 0

r = \frac{- 1 \pm i \sqrt{3}}{2} = - e^{\pm \frac{π i}{3}}

a_{n} = {(- 1)}^{n} {{A e}^{\frac{n π i}{3}} + {B e}^{- \frac{n π i}{3}}}

a_{2} = - a_{1} - 2 a_{0} \Rightarrow - 1 = - 1 - 2 a_{0} \Rightarrow a_{0} = 0

a_{0} = A + B = 0

a_{1} = - {A \frac{1 + i \sqrt{3}}{2} + B \frac{1 - i \sqrt{3}}{2}} = 1

A {\frac{1 + i \sqrt{3}}{2} - \frac{1 - i \sqrt{3}}{2}} = - 1

A = \frac{i}{\sqrt{3}} = \frac{1}{\sqrt{3}} e^{\frac{π i}{2}} = - B

a_{n} = \frac{{(- 1)}^{n}}{\sqrt{3}} {e^{\frac{n π i}{3} + \frac{π i}{2}} - e^{- \frac{n π i}{3} + \frac{π i}{2}}}

a_{n} = \frac{{(- 1)}^{n}}{\sqrt{3}} {\cos (\frac{n π}{3} + \frac{π}{2}) - \cos (- \frac{n π}{3} + \frac{π}{2}) + i [\sin (\frac{n π}{3} + \frac{π}{2}) - \sin (- \frac{n π}{3} + \frac{π}{2})]}

a_{n} = \frac{{(- 1)}^{n}}{\sqrt{3}} {- 2 \sin (\frac{n π}{3}) + i [\cos (\frac{n π}{3}) - \cos (\frac{n π}{3})}

a_{n} = \frac{{(- 1)}^{n + 1} 2 \sin (\frac{n π}{3})}{\sqrt{3}}

Commented by solihin last updated on 24/Jan/22

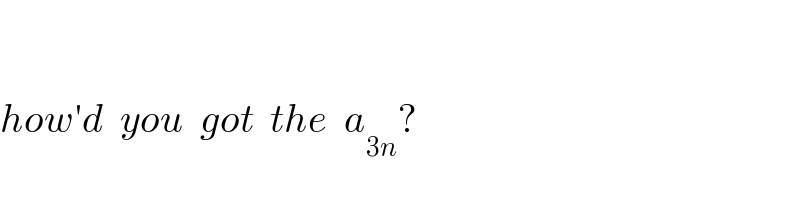
{h o w}^{'} d y o u g o t t h e a_{3 n} ?

Commented by solihin last updated on 24/Jan/22

i m e a n t h e s e q u e n c e

Commented by mr W last updated on 24/Jan/22

w i t h a_{1} = 1, a_{2} = - 1 a n d a_{n} = - a_{n - 1} - 2 a_{n - 2}

y o u g e t

a_{3} = 0

a_{4} = 1

a_{5} = - 1

a_{6} = 0

a_{7} = 1

a_{8} = - 1

a_{9} = 0

\dots .

t h e n y o u c a n s e e

a_{3 n} = 0

a_{3 n + 1} = 1

a_{3 n + 2} = - 1

t h i s i s n o t a n “ {e x a c t}^{″} m e t h o d . i t

i s b a s e d o n “ {o b s e r v a t i o n}^{″} .

t h e r e f o r e i h a v e g i v e n a s e c o n d a n d

m o r e g e n e r a l m e t h o d .

Commented by Tawa11 last updated on 24/Jan/22

Great sir .

Commented by Jamshidbek last updated on 25/Jan/22

Mistake

Commented by mr W last updated on 25/Jan/22

y o u s h o u l d b e m o r e c l e a r s i r! w h a t d o

y o u m e a n ? i s t h e r e a m i s t a k e i n t h e

q u e s t i o n o r i s t h e r e a m i s t a k e i n m y

s o l u t i o n o r w h a t e l s e ?

Commented by aleks041103 last updated on 25/Jan/22

I t h i n k t h a t y o u c a n a l s o p r o v e t h i s

b y i n d u c t i o n .