Question Number 50913 by peter frank last updated on 22/Dec/18

$${A}\:{ball}\:{is}\:{dropped}\:{from} \\ $$$${height}\:{h}.{it}\:{strikes}\:{the}\:{ground} \\ $$$${and}\:{rises},{the}\:{coefficiate} \\ $$$${of}\:{restitution}\:{being}\:\:{e} \\ $$$${what}\:{is}\:{the}\:{total}\:{distance} \\ $$$${it}\:{moves}\:\:{and}\:\:{the}\:{time}\: \\ $$$${before}\:{it}\:{comes}\:{to}\:{rest}? \\ $$$$ \\ $$$$ \\ $$
Answered by mr W last updated on 22/Dec/18
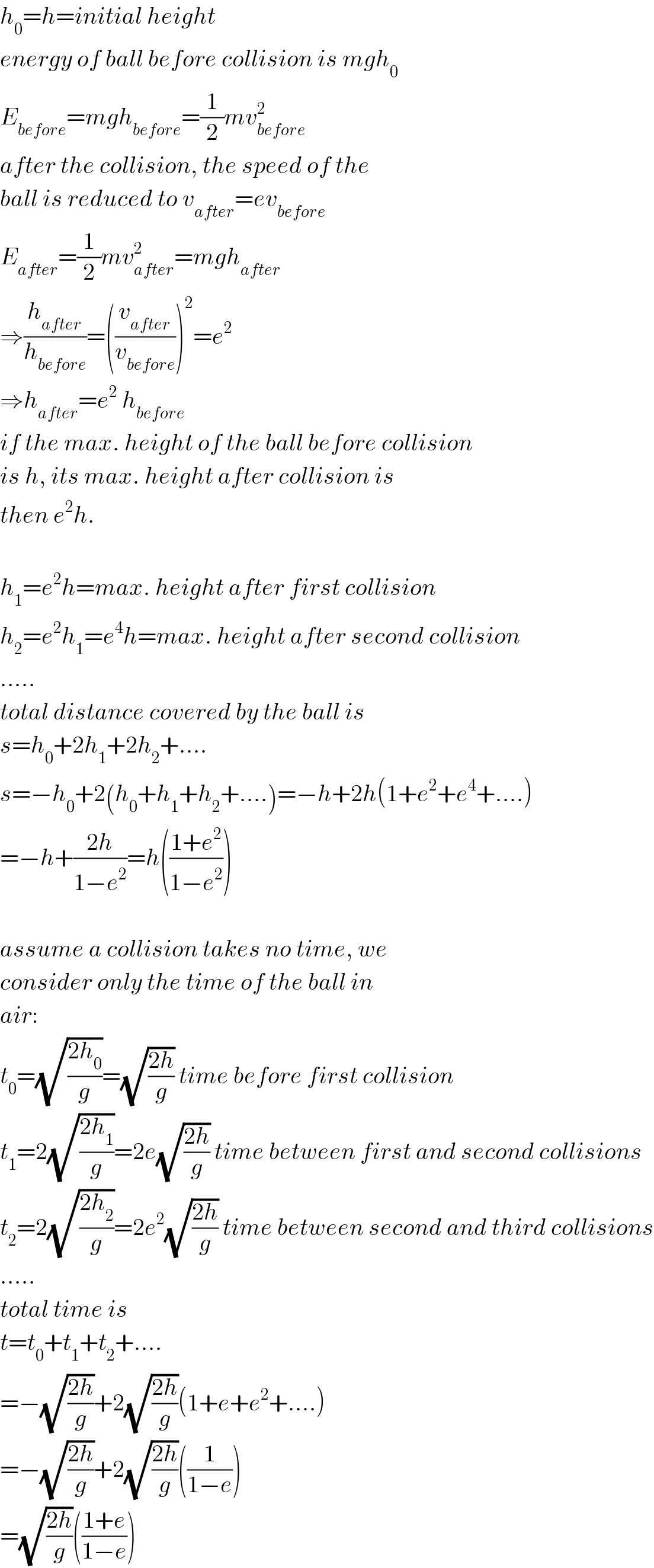
$${h}_{\mathrm{0}} ={h}={initial}\:{height} \\ $$$${energy}\:{of}\:{ball}\:{before}\:{collision}\:{is}\:{mgh}_{\mathrm{0}} \\ $$$${E}_{{before}} ={mgh}_{{before}} =\frac{\mathrm{1}}{\mathrm{2}}{mv}_{{before}} ^{\mathrm{2}} \\ $$$${after}\:{the}\:{collision},\:{the}\:{speed}\:{of}\:{the} \\ $$$${ball}\:{is}\:{reduced}\:{to}\:{v}_{{after}} ={ev}_{{before}} \\ $$$${E}_{{after}} =\frac{\mathrm{1}}{\mathrm{2}}{mv}_{{after}} ^{\mathrm{2}} ={mgh}_{{after}} \\ $$$$\Rightarrow\frac{{h}_{{after}} }{{h}_{{before}} }=\left(\frac{{v}_{{after}} }{{v}_{{before}} }\right)^{\mathrm{2}} ={e}^{\mathrm{2}} \\ $$$$\Rightarrow{h}_{{after}} ={e}^{\mathrm{2}} \:{h}_{{before}} \\ $$$${if}\:{the}\:{max}.\:{height}\:{of}\:{the}\:{ball}\:{before}\:{collision} \\ $$$${is}\:{h},\:{its}\:{max}.\:{height}\:{after}\:{collision}\:{is} \\ $$$${then}\:{e}^{\mathrm{2}} {h}. \\ $$$$ \\ $$$${h}_{\mathrm{1}} ={e}^{\mathrm{2}} {h}={max}.\:{height}\:{after}\:{first}\:{collision} \\ $$$${h}_{\mathrm{2}} ={e}^{\mathrm{2}} {h}_{\mathrm{1}} ={e}^{\mathrm{4}} {h}={max}.\:{height}\:{after}\:{second}\:{collision} \\ $$$$….. \\ $$$${total}\:{distance}\:{covered}\:{by}\:{the}\:{ball}\:{is} \\ $$$${s}={h}_{\mathrm{0}} +\mathrm{2}{h}_{\mathrm{1}} +\mathrm{2}{h}_{\mathrm{2}} +…. \\ $$$${s}=−{h}_{\mathrm{0}} +\mathrm{2}\left({h}_{\mathrm{0}} +{h}_{\mathrm{1}} +{h}_{\mathrm{2}} +….\right)=−{h}+\mathrm{2}{h}\left(\mathrm{1}+{e}^{\mathrm{2}} +{e}^{\mathrm{4}} +….\right) \\ $$$$=−{h}+\frac{\mathrm{2}{h}}{\mathrm{1}−{e}^{\mathrm{2}} }={h}\left(\frac{\mathrm{1}+{e}^{\mathrm{2}} }{\mathrm{1}−{e}^{\mathrm{2}} }\right) \\ $$$$ \\ $$$${assume}\:{a}\:{collision}\:{takes}\:{no}\:{time},\:{we} \\ $$$${consider}\:{only}\:{the}\:{time}\:{of}\:{the}\:{ball}\:{in} \\ $$$${air}: \\ $$$${t}_{\mathrm{0}} =\sqrt{\frac{\mathrm{2}{h}_{\mathrm{0}} }{{g}}}=\sqrt{\frac{\mathrm{2}{h}}{{g}}}\:{time}\:{before}\:{first}\:{collision} \\ $$$${t}_{\mathrm{1}} =\mathrm{2}\sqrt{\frac{\mathrm{2}{h}_{\mathrm{1}} }{{g}}}=\mathrm{2}{e}\sqrt{\frac{\mathrm{2}{h}}{{g}}}\:{time}\:{between}\:{first}\:{and}\:{second}\:{collisions} \\ $$$${t}_{\mathrm{2}} =\mathrm{2}\sqrt{\frac{\mathrm{2}{h}_{\mathrm{2}} }{{g}}}=\mathrm{2}{e}^{\mathrm{2}} \sqrt{\frac{\mathrm{2}{h}}{{g}}}\:{time}\:{between}\:{second}\:{and}\:{third}\:{collisions} \\ $$$$….. \\ $$$${total}\:{time}\:{is} \\ $$$${t}={t}_{\mathrm{0}} +{t}_{\mathrm{1}} +{t}_{\mathrm{2}} +…. \\ $$$$=−\sqrt{\frac{\mathrm{2}{h}}{{g}}}+\mathrm{2}\sqrt{\frac{\mathrm{2}{h}}{{g}}}\left(\mathrm{1}+{e}+{e}^{\mathrm{2}} +….\right) \\ $$$$=−\sqrt{\frac{\mathrm{2}{h}}{{g}}}+\mathrm{2}\sqrt{\frac{\mathrm{2}{h}}{{g}}}\left(\frac{\mathrm{1}}{\mathrm{1}−{e}}\right) \\ $$$$=\sqrt{\frac{\mathrm{2}{h}}{{g}}}\left(\frac{\mathrm{1}+{e}}{\mathrm{1}−{e}}\right) \\ $$
Commented by peter frank last updated on 22/Dec/18
$${sorry}\:{sir}\:{more}\:{explanation}\:{with}\: \\ $$$${diagram}\:{if}\:{possible} \\ $$
Commented by peter frank last updated on 22/Dec/18
![the ans given is total distance=((h+he^2 )/(1−e^2 )) total Time=(√((2h)/g)) [((1+e)/(1−e))]](https://www.tinkutara.com/question/Q50935.png)
$${the}\:{ans}\:{given}\:{is} \\ $$$${total}\:{distance}=\frac{{h}+{he}^{\mathrm{2}} }{\mathrm{1}−{e}^{\mathrm{2}} } \\ $$$${total}\:{Time}=\sqrt{\frac{\mathrm{2}{h}}{{g}}}\:\left[\frac{\mathrm{1}+{e}}{\mathrm{1}−{e}}\right] \\ $$
Commented by peter frank last updated on 22/Dec/18
$${yes}\:{sir}\:\:{its}\:{true}.{thank}\:{you} \\ $$$${very}\:{much} \\ $$
Commented by mr W last updated on 23/Dec/18

$${it}'{s}\:{interesting}\:{to}\:{know}\:{that}\:{the}\:{ball} \\ $$$${will}\:{infinitely}\:{move}\:{down}\:{and}\:{up}, \\ $$$${but}\:{all}\:{this}\:{happens}\:{within}\:{a}\:{limited}\:{time} \\ $$$${and}\:{limited}\:{distance}. \\ $$
