Question Number 29144 by tawa tawa last updated on 04/Feb/18

Answered by ajfour last updated on 04/Feb/18
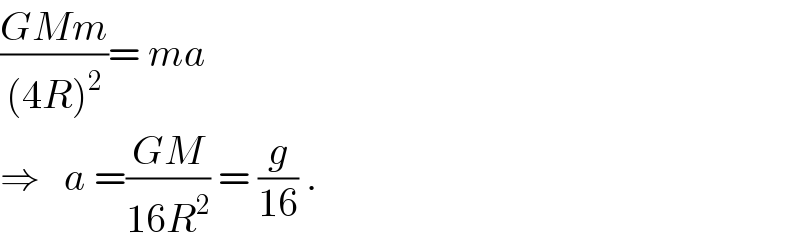
Commented by tawa tawa last updated on 04/Feb/18

Commented by tawa tawa last updated on 04/Feb/18
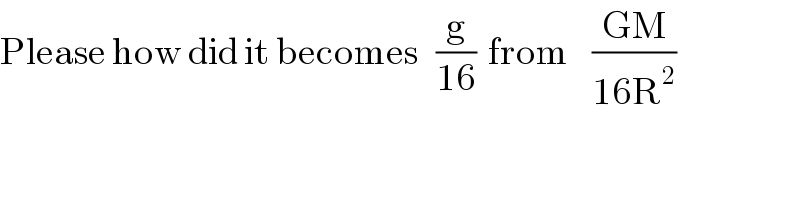
Commented by tawa tawa last updated on 04/Feb/18

Commented by ajfour last updated on 04/Feb/18

Commented by tawa tawa last updated on 04/Feb/18

