Menu Close

A-body-starts-rotating-about-a-stationary-axis-with-an-angular-acceleration-b-2t-rad-s-2-How-soon-after-the-beginning-of-rotation-will-the-total-acceleration-vector-of-an-arbitrary-point-on-the-bo

Tinku Tara 0 Comments
Pin




Question Number 20916 by Tinkutara last updated on 07/Sep/17
A body starts rotating about a stationary axis with an angular acceleration b = 2t rad/s^2 . How soon after the beginning of rotation will the total acceleration vector of an arbitrary point on the body forms an angle of 60° with its velocity vector? (1) (2(√3))^(1/3) s (2) (2(√3))^(1/2) s (3) (2(√3)) s (4) (2(√3))^2 s
$$\mathrm{A}\:\mathrm{body}\:\mathrm{starts}\:\mathrm{rotating}\:\mathrm{about}\:\mathrm{a} \\ $$$$\mathrm{stationary}\:\mathrm{axis}\:\mathrm{with}\:\mathrm{an}\:\mathrm{angular} \\ $$$$\mathrm{acceleration}\:{b}\:=\:\mathrm{2}{t}\:\mathrm{rad}/\mathrm{s}^{\mathrm{2}} .\:\mathrm{How}\:\mathrm{soon} \\ $$$$\mathrm{after}\:\mathrm{the}\:\mathrm{beginning}\:\mathrm{of}\:\mathrm{rotation}\:\mathrm{will}\:\mathrm{the} \\ $$$$\mathrm{total}\:\mathrm{acceleration}\:\mathrm{vector}\:\mathrm{of}\:\mathrm{an}\:\mathrm{arbitrary} \\ $$$$\mathrm{point}\:\mathrm{on}\:\mathrm{the}\:\mathrm{body}\:\mathrm{forms}\:\mathrm{an}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{60}° \\ $$$$\mathrm{with}\:\mathrm{its}\:\mathrm{velocity}\:\mathrm{vector}? \\ $$$$\left(\mathrm{1}\right)\:\left(\mathrm{2}\sqrt{\mathrm{3}}\right)^{\mathrm{1}/\mathrm{3}} \:\mathrm{s} \\ $$$$\left(\mathrm{2}\right)\:\left(\mathrm{2}\sqrt{\mathrm{3}}\right)^{\mathrm{1}/\mathrm{2}} \:\mathrm{s} \\ $$$$\left(\mathrm{3}\right)\:\left(\mathrm{2}\sqrt{\mathrm{3}}\right)\:\mathrm{s} \\ $$$$\left(\mathrm{4}\right)\:\left(\mathrm{2}\sqrt{\mathrm{3}}\right)^{\mathrm{2}} \:\mathrm{s} \\ $$
Answered by ajfour last updated on 08/Sep/17
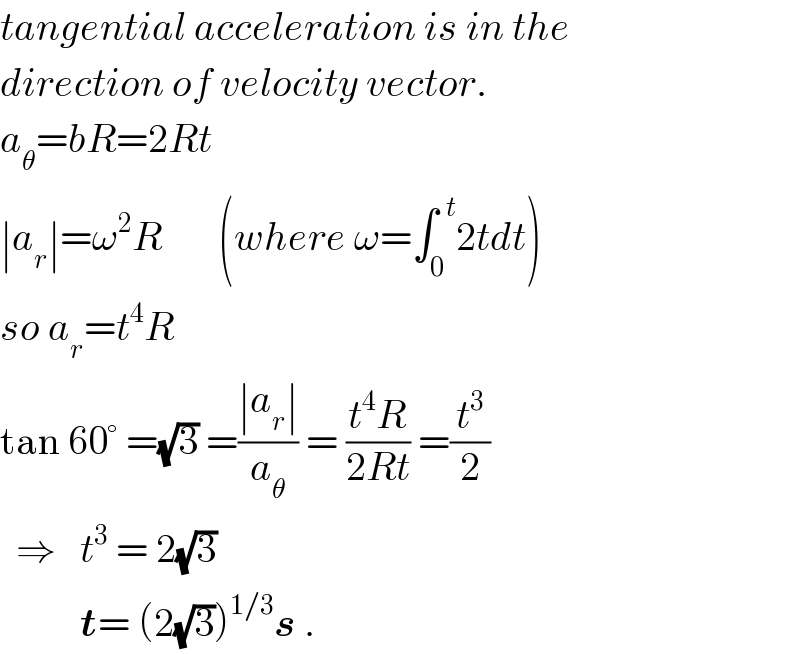
tangential acceleration is in the direction of velocity vector. a_θ =bR=2Rt ∣a_r ∣=ω^2 R (where ω=∫_0 ^( t) 2tdt) so a_r =t^4 R tan 60° =(√3) =((∣a_r ∣)/a_θ ) = ((t^4 R)/(2Rt)) =(t^3 /2) ⇒ t^3 = 2(√3) t= (2(√3))^(1/3) s .
$${tangential}\:{acceleration}\:{is}\:{in}\:{the} \\ $$$${direction}\:{of}\:{velocity}\:{vector}. \\ $$$${a}_{\theta} ={bR}=\mathrm{2}{Rt} \\ $$$$\mid{a}_{{r}} \mid=\omega^{\mathrm{2}} {R}\:\:\:\:\:\:\:\left({where}\:\omega=\int_{\mathrm{0}} ^{\:\:{t}} \mathrm{2}{tdt}\right) \\ $$$${so}\:{a}_{{r}} ={t}^{\mathrm{4}} {R} \\ $$$$\mathrm{tan}\:\mathrm{60}°\:=\sqrt{\mathrm{3}}\:=\frac{\mid{a}_{{r}} \mid}{{a}_{\theta} }\:=\:\frac{{t}^{\mathrm{4}} {R}}{\mathrm{2}{Rt}}\:=\frac{{t}^{\mathrm{3}} }{\mathrm{2}} \\ $$$$\:\:\Rightarrow\:\:\:{t}^{\mathrm{3}} \:=\:\mathrm{2}\sqrt{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\boldsymbol{{t}}=\:\left(\mathrm{2}\sqrt{\mathrm{3}}\right)^{\mathrm{1}/\mathrm{3}} \boldsymbol{{s}}\:. \\ $$
Commented by Tinkutara last updated on 08/Sep/17
Thank you very much Sir!
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *