Question Number 102076 by pete last updated on 06/Jul/20
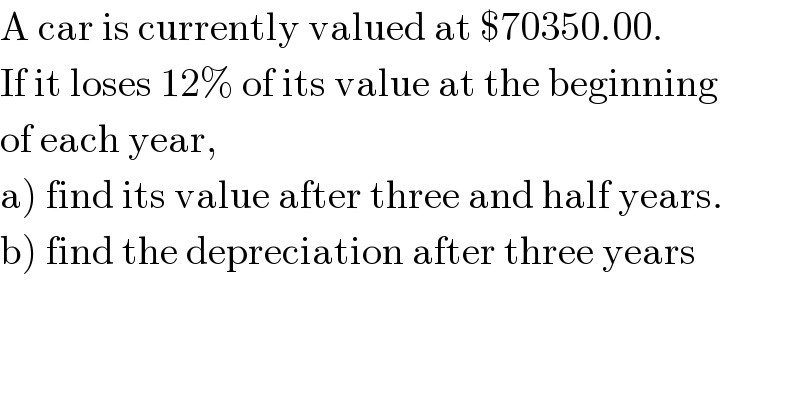
Commented by PRITHWISH SEN 2 last updated on 06/Jul/20

Commented by pete last updated on 06/Jul/20
Commented by PRITHWISH SEN 2 last updated on 06/Jul/20

Commented by pete last updated on 07/Jul/20

