Question Number 105037 by Dwaipayan Shikari last updated on 25/Jul/20

$${A}\:{heavy}\:{man}\:,\:{slips}\:\:{from}\:{a}\:{roof}\:{of}\:{a}\:{high}\:{building}\:{of} \\ $$$${height}\:'{H}'.\:{When}\:{he}\:{was}\:{at}\:{a}\:{height}\:{of}\:'{h}'\:\:{he}\:{realised}\:{that}\:{the} \\ $$$${ground}\:{beneath}\:{him}\:{was}\:{pretty}\:{hard}.\:{To}\:{avoid}\:{injury},\:{he}\:{thrown} \\ $$$${away}\:{his}\:{suitcase}\:{of}\:{mass}\:'{m}'.\:{After}\:{doing}\:{this},{he}\:{prevented}\:{his} \\ $$$${falling}\:{on}\:{the}\:{hard}\:{ground}\:{and}\:{fell}\:{on}\:{a}\:{pond}.\:{The}\:{mass}\:{of}\:{the} \\ $$$${man}\:{was}\:'{M}'.\:{The}\:{distance}\:{of}\:{the}\:{pond}\:{from}\:{the}\:{hard}\:{ground}\:{was}\: \\ $$$$'{x}'.{And}\:{he}\:{just}\:{avoided}\:{from}\:{falling}\:{on}\:{the}\:{hard}\:{ground}. \\ $$$$ \\ $$$${Find}\:{the}\:{velocity}\:{required}\:{to}\:{throw}\:{away}\:{the}\:{suitcase}\:{as}\:{the}\:{man} \\ $$$${don}'{t}\:{fall}\:{on}\:{the}\:{hard}\:{ground}.\left({There}\:{was}\:{no}\:{horizontal}\:{velocity}\:{of}\:{the}\right. \\ $$$$\left.{suitcase}−{man}\:{system}\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Answered by mr W last updated on 25/Jul/20
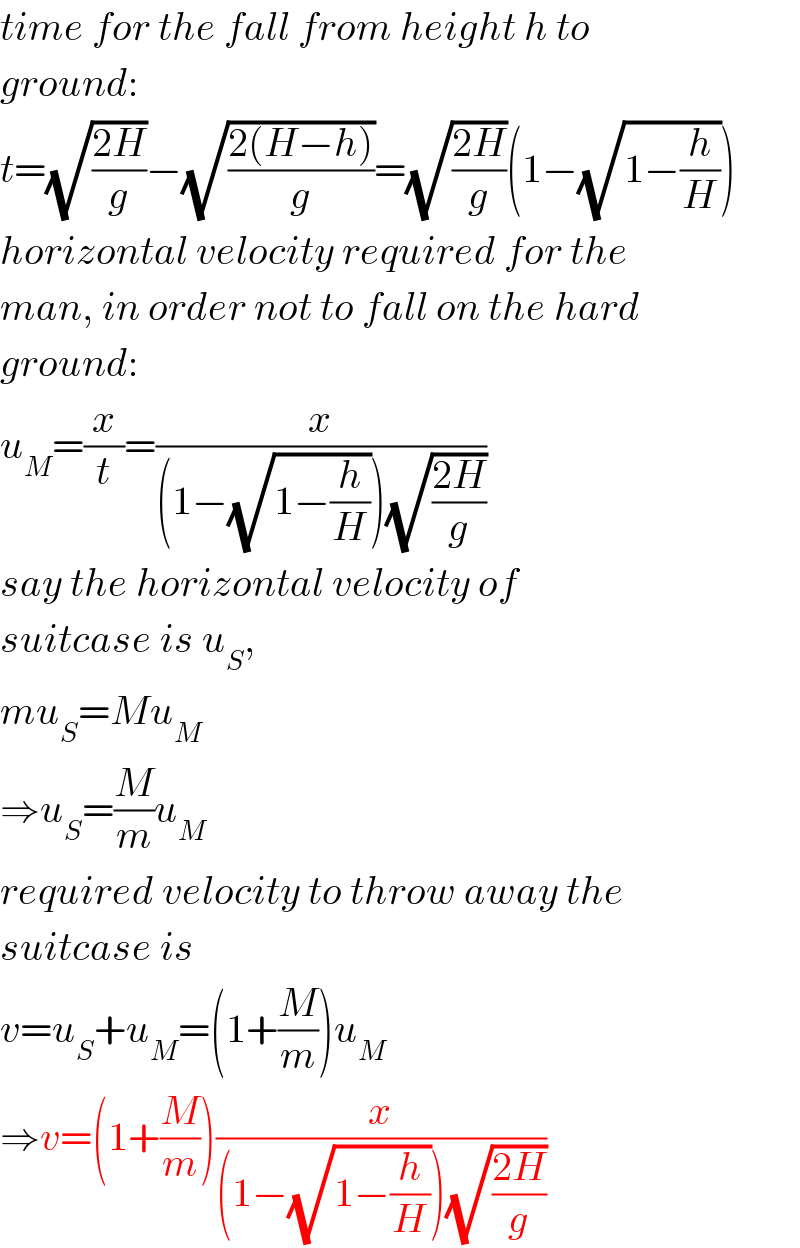
$${time}\:{for}\:{the}\:{fall}\:{from}\:{height}\:{h}\:{to} \\ $$$${ground}: \\ $$$${t}=\sqrt{\frac{\mathrm{2}{H}}{{g}}}−\sqrt{\frac{\mathrm{2}\left({H}−{h}\right)}{{g}}}=\sqrt{\frac{\mathrm{2}{H}}{{g}}}\left(\mathrm{1}−\sqrt{\mathrm{1}−\frac{{h}}{{H}}}\right) \\ $$$${horizontal}\:{velocity}\:{required}\:{for}\:{the} \\ $$$${man},\:{in}\:{order}\:{not}\:{to}\:{fall}\:{on}\:{the}\:{hard} \\ $$$${ground}: \\ $$$${u}_{{M}} =\frac{{x}}{{t}}=\frac{{x}}{\left(\mathrm{1}−\sqrt{\mathrm{1}−\frac{{h}}{{H}}}\right)\sqrt{\frac{\mathrm{2}{H}}{{g}}}} \\ $$$${say}\:{the}\:{horizontal}\:{velocity}\:{of} \\ $$$${suitcase}\:{is}\:{u}_{{S}} , \\ $$$${mu}_{{S}} ={Mu}_{{M}} \\ $$$$\Rightarrow{u}_{{S}} =\frac{{M}}{{m}}{u}_{{M}} \\ $$$${required}\:{velocity}\:{to}\:{throw}\:{away}\:{the} \\ $$$${suitcase}\:{is} \\ $$$${v}={u}_{{S}} +{u}_{{M}} =\left(\mathrm{1}+\frac{{M}}{{m}}\right){u}_{{M}} \\ $$$$\Rightarrow{v}=\left(\mathrm{1}+\frac{{M}}{{m}}\right)\frac{{x}}{\left(\mathrm{1}−\sqrt{\mathrm{1}−\frac{{h}}{{H}}}\right)\sqrt{\frac{\mathrm{2}{H}}{{g}}}} \\ $$
Commented by Dwaipayan Shikari last updated on 25/Jul/20

$${Great}\:{sir}! \\ $$
