Question Number 24177 by NECx last updated on 13/Nov/17
$${A}\:{lorry}\:{goes}\:{round}\:{an}\:{unbanked} \\ $$$${curve}.{If}\:{the}\:{radius}\:{of}\:{the}\:{curve} \\ $$$${is}\:\mathrm{30}{m}\:{and}\:{the}\:{coefficient}\:{of} \\ $$$${friction}\:{between}\:{the}\:{ground}\:{and} \\ $$$${the}\:{tyre}\:{is}\:\mathrm{0}.\mathrm{6}.\:{Calculate}\:{the} \\ $$$${maximum}\:{speed}\:{of}\:{the}\:{lorry}. \\ $$$$\left({g}=\mathrm{10}{m}/{s}^{\mathrm{2}} \right) \\ $$$$ \\ $$$$ \\ $$$${please}\:{buddies}\:{help} \\ $$
Commented by NECx last updated on 14/Nov/17
$${please}\:{help} \\ $$
Answered by mrW1 last updated on 14/Nov/17
$$\frac{{mv}^{\mathrm{2}} }{{r}}\leqslant\mu{mg} \\ $$$$\Rightarrow{v}\leqslant\sqrt{\mu{gr}}=\sqrt{\mathrm{0}.\mathrm{6}×\mathrm{10}×\mathrm{30}}=\mathrm{13}.\mathrm{4}\:{m}/{m}=\mathrm{48}\:{km}/{h} \\ $$
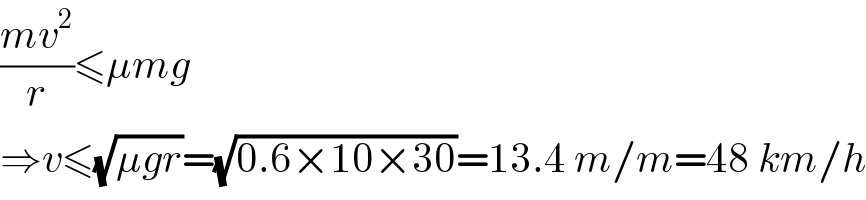
Commented by NECx last updated on 14/Nov/17
$${but}\:{I}\:{thought}\:{that}\:{the}\:{centripetal} \\ $$$${force}\:{was}\:{going}\:{to}\:{be}\:{greater}\:{than} \\ $$$${the}\:{frictional}\:{or}\:{equal}\:{not}\:{less}\: \\ $$$${because}\:{it}\:{is}\:{already}\:{in}\:{motion} \\ $$$${thus}\:{overcoming}\:{the}\:{frictional} \\ $$$${force}. \\ $$$$ \\ $$$${Please}\:{explain}\:{why}\:\frac{{mv}^{\mathrm{2}} }{{r}}\leqslant\mu{mg} \\ $$
Commented by mrW1 last updated on 14/Nov/17
$${The}\:{centripetal}\:{force}\:\frac{{mv}^{\mathrm{2}} }{\mathrm{2}}\:{is}\: \\ $$$${provided}\:{by}\:{the}\:{friction}\:{force}\:{f}, \\ $$$${and}\:{the}\:{friction}\:{force}\:{f}\:{may}\:{not} \\ $$$${be}\:{more}\:{than}\:\mu{mg},\:{thus} \\ $$$$\frac{{mv}^{\mathrm{2}} }{\mathrm{2}}\leqslant\mu{mg} \\ $$
Commented by NECx last updated on 15/Nov/17
$${I}\:{now}\:{understand}….\:{Thanks}\:{sir}. \\ $$
Answered by ajfour last updated on 14/Nov/17
$$\mu{mg}\:=\:{mv}^{\mathrm{2}} /{r} \\ $$$$\Rightarrow\:\:{v}\:=\sqrt{\mu{gr}}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:=\sqrt{\mathrm{0}.\mathrm{6}×\mathrm{10}×\mathrm{30}}\:=\mathrm{6}\sqrt{\mathrm{5}}\:{m}/{s}\:. \\ $$