Question Number 23865 by Tinkutara last updated on 08/Nov/17

$$\mathrm{A}\:\mathrm{man}\:\mathrm{of}\:\mathrm{mass}\:{m},\:\mathrm{standing}\:\mathrm{at}\:\mathrm{the} \\ $$$$\mathrm{bottom}\:\mathrm{of}\:\mathrm{the}\:\mathrm{staircase}\:\mathrm{of}\:\mathrm{height}\:{L} \\ $$$$\mathrm{climbs}\:\mathrm{it}\:\mathrm{and}\:\mathrm{stands}\:\mathrm{at}\:\mathrm{its}\:\mathrm{top}. \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Work}\:\mathrm{done}\:\mathrm{by}\:\mathrm{all}\:\mathrm{forces}\:\mathrm{on}\:\mathrm{man}\:\mathrm{is} \\ $$$$\mathrm{equal}\:\mathrm{to}\:\mathrm{the}\:\mathrm{rise}\:\mathrm{in}\:\mathrm{potential}\:\mathrm{energy} \\ $$$${mgL}. \\ $$$$\left(\mathrm{2}\right)\:\mathrm{Work}\:\mathrm{done}\:\mathrm{by}\:{all}\:\mathrm{forces}\:\mathrm{on}\:\mathrm{man}\:\mathrm{is} \\ $$$$\mathrm{zero}. \\ $$$$\left(\mathrm{3}\right)\:\mathrm{Work}\:\mathrm{done}\:\mathrm{by}\:\mathrm{the}\:\mathrm{gravitational} \\ $$$$\mathrm{force}\:\mathrm{on}\:\mathrm{man}\:\mathrm{is}\:{mgL}. \\ $$$$\left(\mathrm{4}\right)\:\mathrm{The}\:\mathrm{reaction}\:\mathrm{force}\:\mathrm{from}\:\mathrm{a}\:\mathrm{step}\:\mathrm{does} \\ $$$$\mathrm{not}\:\mathrm{do}\:\mathrm{work}\:\mathrm{because}\:\mathrm{the}\:\mathrm{point}\:\mathrm{of} \\ $$$$\mathrm{application}\:\mathrm{of}\:\mathrm{the}\:\mathrm{force}\:\mathrm{does}\:\mathrm{not}\:\mathrm{move} \\ $$$$\mathrm{while}\:\mathrm{the}\:\mathrm{force}\:\mathrm{exists}. \\ $$
Commented by Tinkutara last updated on 09/Nov/17
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$
Commented by ajfour last updated on 08/Nov/17
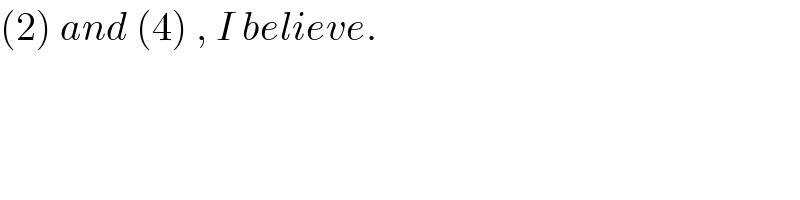
$$\left(\mathrm{2}\right)\:{and}\:\left(\mathrm{4}\right)\:,\:{I}\:{believe}. \\ $$
Commented by Tinkutara last updated on 08/Nov/17

$$\mathrm{Yes}!\:\mathrm{But}\:\mathrm{why}? \\ $$
Commented by ajfour last updated on 08/Nov/17

$${let}\:{us}\:{choose}\:{to}\:{include}\:{in}\:{our} \\ $$$${system}\:{boundary}-\:{just}\:{the}\:{man}: \\ $$$${the}\:{stairs}\:{do}\:{no}\:{work}\:{since}\:{the} \\ $$$${point}\:{of}\:{application}\:{of}\:{force}\:{is} \\ $$$${there}\:{to}\:{receive}\:{the}\:{force}\:{and} \\ $$$${doesn}'{t}\:{move}\:\:{as}\:{long}\:{as}\:{the}\:{force} \\ $$$${is}\:{acting}\:{there}\:\left({while}\:{the}\:{centre}\:\right. \\ $$$$\left.\:{of}\:{mass}\:{of}\:{man}\:{moves}\right). \\ $$$${Force}\:{of}\:{gravity}\:{does}\:{negative} \\ $$$${work}\:{since}\:{displacement}\:{of} \\ $$$${centre}\:{of}\:{mass}\:{of}\:{man}\:{is}\:{upwards} \\ $$$${while}\:{the}\:{force}\:{of}\:{gravity}\:\left({weight}\right) \\ $$$${is}\:{downwards}.\:{So}\:\left({W}_{{g}} =\:−{mgL}\right). \\ $$$${As}\:{our}\:{system}\:{that}\:{includes} \\ $$$${only}\:{the}\:{man}\:{has}\:\:{no}\:{change}\:{in} \\ $$$${its}\:{kinetic}\:{energy},\:{so}\:{work}\:{done} \\ $$$${by}\:{all}\:{forces}\:{on}\:{man}\:{is}\:{zero}. \\ $$$${Work}\:{done}\:{by}\:{forces}\:\left({muscular}\right) \\ $$$${of}\:{man}\:{is}\:{positive}.\:{It}\:{leads}\:{to}\:{a} \\ $$$${decrease}\:{in}\:{his}\:{store}\:{of}\:{internal} \\ $$$${energy}\:. \\ $$$$\bigtriangleup{U}_{{internal}} +\bigtriangleup{K}_{{man}} ={W}_{{g}} +{W}_{{stairs}} \\ $$$$\bigtriangleup{U}_{{internal}} +\mathrm{0}\:=\:−{mgL}+\mathrm{0} \\ $$$${Also}\:\bigtriangleup{U}_{{internal}} =\:−{W}_{{man}'{s}\:{muscular}\:{forces}} \:. \\ $$
Commented by Tinkutara last updated on 08/Nov/17

$$\mathrm{So}\:\mathrm{point}\:\mathrm{of}\:\mathrm{application}\:\mathrm{must}\:\mathrm{move} \\ $$$$\mathrm{along}\:\mathrm{with}\:\mathrm{COM}\:\mathrm{of}\:\mathrm{man}\:\mathrm{to}\:\mathrm{do}\:\mathrm{some} \\ $$$$\mathrm{work}? \\ $$
Commented by ajfour last updated on 08/Nov/17

$${yes}\:{strictly}\:{work}\:{done}\:{by}\:{a}\:{force} \\ $$$${is}\:=\int\:\bar {{F}}.{d}\bar {{s}}\:\:\:\:\:{where}\:{d}\bar {{s}}\:{is}\:{the}\:{little} \\ $$$${displacement}\:{that}\:{occurs}\:{of}\:{the} \\ $$$${point}\:{where}\:{the}\:{force}\:\bar {{F}}\:{acted} \\ $$$${and}\:{remained}\:\bar {{F}}\:{as}\:{long}\:\:{as}\: \\ $$$${displacement}\:{d}\bar {{s}}\:\:{took}\:{place}. \\ $$
Commented by Physics lover last updated on 09/Nov/17
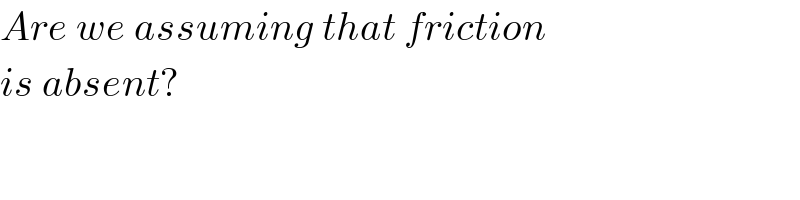
$${Are}\:{we}\:{assuming}\:{that}\:{friction} \\ $$$${is}\:{absent}? \\ $$
