Question Number 119645 by aurpeyz last updated on 26/Oct/20

$${A}\:{particle}\:{of}\:{mass}\:{m}_{\mathrm{1}} \:{lies}\:{on}\:{a}\:{smooth} \\ $$$${horizontal}\:{table}\:{and}\:{its}\:{connected}\:{to} \\ $$$${a}\:{freely}\:{hanging}\:{particle}\:{of}\:{mass}\:{m}_{\mathrm{2}} \\ $$$${by}\:{a}\:{light}\:{inextensible}\:{string}\:{passing} \\ $$$${over}\:{a}\:{smooth}\:{fixed}\:{pulley}\:{situated} \\ $$$${at}\:{the}\:{edge}\:{of}\:{the}\:{table}.\:{obtain}\:{the} \\ $$$${expression}\:{for}\:{the}\:{time}\:{taken}\:{for} \\ $$$${mass}\:{m}_{\mathrm{1}\:} {to}\:{reach}\:{the}\:{edge}\:{of}\:{the}\:{table}. \\ $$
Commented by aurpeyz last updated on 26/Oct/20

$${pls}\:{help} \\ $$
Answered by TANMAY PANACEA last updated on 26/Oct/20
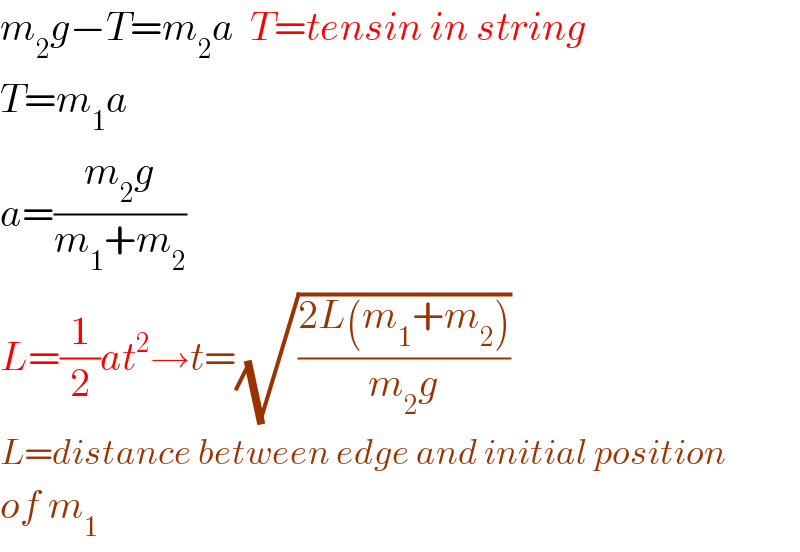
$${m}_{\mathrm{2}} {g}−{T}={m}_{\mathrm{2}} {a}\:\:{T}={tensin}\:{in}\:{string} \\ $$$${T}={m}_{\mathrm{1}} {a} \\ $$$${a}=\frac{{m}_{\mathrm{2}} {g}}{{m}_{\mathrm{1}} +{m}_{\mathrm{2}} } \\ $$$${L}=\frac{\mathrm{1}}{\mathrm{2}}{at}^{\mathrm{2}} \rightarrow{t}=\sqrt{\frac{\mathrm{2}{L}\left({m}_{\mathrm{1}} +{m}_{\mathrm{2}} \right)}{{m}_{\mathrm{2}} {g}}}\: \\ $$$${L}={distance}\:{between}\:{edge}\:{and}\:{initial}\:{position} \\ $$$${of}\:{m}_{\mathrm{1}} \\ $$
Commented by aurpeyz last updated on 28/Oct/20

$${thanks} \\ $$
Commented by TANMAY PANACEA last updated on 28/Oct/20

$${most}\:{welcome} \\ $$
