A-polynomial-satisfies-f-x-2-2-f-x-f-x-Assuming-that-f-x-0-for-2-x-2-what-is-the-value-of-f-2-f-1-

Question Number 110594 by Aina Samuel Temidayo last updated on 29/Aug/20

A polynomial satisfies

f (x^{2} - 2) = f (x) f (- x) . Assuming that

f (x) \neq 0 for - 2 ⩽ x ⩽ 2, what is the value

of f (- 2) + f (1) ?

Answered by Aziztisffola last updated on 29/Aug/20

f (- 2) = {(f (0))}^{2}

f (- 1) = f (1) f (- 1) \Rightarrow f (1) = 1 (f (- 1) \neq 0)

f (2) = f (2) f (- 2) \Rightarrow f (- 2) = 1 (f (- 2) \neq 0)

f (- 2) + f (1) = 1 + 1 = 2

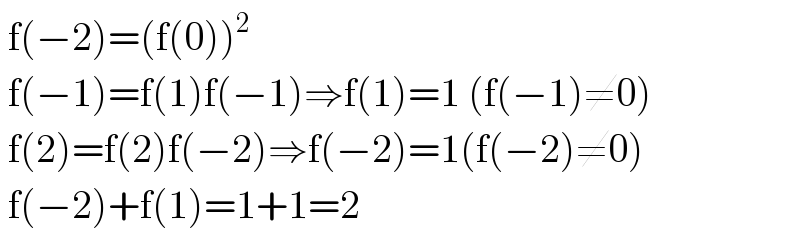
Commented by Aina Samuel Temidayo last updated on 29/Aug/20

Please I {don}^{'} t understand . Try

explaining everything in details .

Commented by Aziztisffola last updated on 29/Aug/20

x = 0 \Rightarrow f (- 2) = f (0) f (0) = f^{2} (0)

x = 1 \Rightarrow f (- 1) = f (1) f (- 1)

\Rightarrow f (1) = \frac{f (- 1)}{f (- 1)} = 1 (f (- 1) \neq 0)

x = 2 \Rightarrow f (2) = f (2) f (- 2)

\Rightarrow f (- 2) = \frac{f (2)}{f (2)} = 1 (f (- 2) \neq 0)

then f (- 2) + f (1) = 1 + 1 = 2

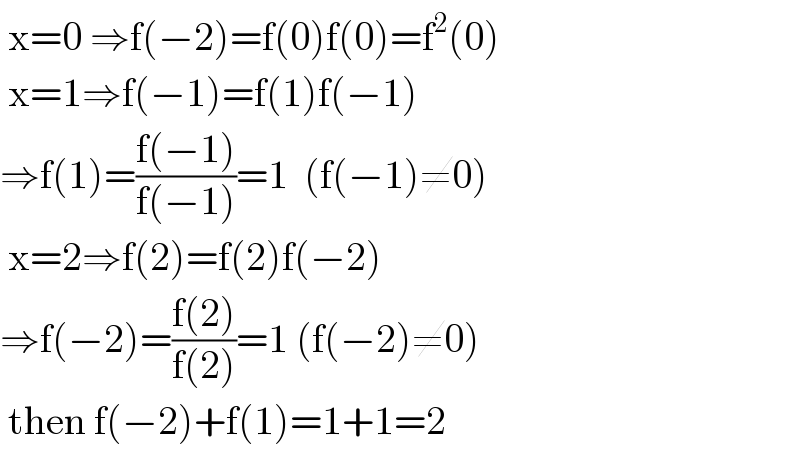
Commented by Aina Samuel Temidayo last updated on 03/Sep/20

Thanks .