Menu Close

A-semicircle-is-tangent-to-both-legs-of-a-right-triangle-and-has-its-centre-on-the-hypotenuse-The-hypotenuse-is-partitioned-into-4-segments-with-lengths-3-12-12-and-x-as-shown-in-the-figure-Det

Tinku Tara 0 Comments
Pin




Question Number 19150 by Tinkutara last updated on 06/Aug/17
A semicircle is tangent to both legs of a right triangle and has its centre on the hypotenuse. The hypotenuse is partitioned into 4 segments, with lengths 3, 12, 12, and x, as shown in the figure. Determine the value of ′x′.
$$\mathrm{A}\:\mathrm{semicircle}\:\mathrm{is}\:\mathrm{tangent}\:\mathrm{to}\:\mathrm{both}\:\mathrm{legs}\:\mathrm{of}\:\mathrm{a} \\ $$$$\mathrm{right}\:\mathrm{triangle}\:\mathrm{and}\:\mathrm{has}\:\mathrm{its}\:\mathrm{centre}\:\mathrm{on}\:\mathrm{the} \\ $$$$\mathrm{hypotenuse}.\:\mathrm{The}\:\mathrm{hypotenuse}\:\mathrm{is} \\ $$$$\mathrm{partitioned}\:\mathrm{into}\:\mathrm{4}\:\mathrm{segments},\:\mathrm{with}\:\mathrm{lengths} \\ $$$$\mathrm{3},\:\mathrm{12},\:\mathrm{12},\:\mathrm{and}\:{x},\:\mathrm{as}\:\mathrm{shown}\:\mathrm{in}\:\mathrm{the}\:\mathrm{figure}. \\ $$$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:'{x}'. \\ $$
Commented by Tinkutara last updated on 06/Aug/17
Commented by ajfour last updated on 06/Aug/17
Commented by ajfour last updated on 06/Aug/17
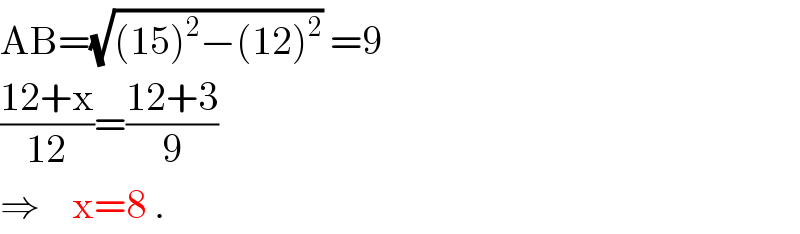
AB=(√((15)^2 −(12)^2 )) =9 ((12+x)/(12))=((12+3)/9) ⇒ x=8 .
$$\mathrm{AB}=\sqrt{\left(\mathrm{15}\right)^{\mathrm{2}} −\left(\mathrm{12}\right)^{\mathrm{2}} }\:=\mathrm{9} \\ $$$$\frac{\mathrm{12}+\mathrm{x}}{\mathrm{12}}=\frac{\mathrm{12}+\mathrm{3}}{\mathrm{9}} \\ $$$$\Rightarrow\:\:\:\:\mathrm{x}=\mathrm{8}\:. \\ $$
Commented by Tinkutara last updated on 06/Aug/17
Thank you very much Sir!
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$
Answered by sandy_suhendra last updated on 06/Aug/17
Commented by allizzwell23 last updated on 06/Aug/17
FD = BF = 12 (radii) AB = (√((3+12)^2 −12^2 )) (Pythagoras theorem) AB = (√(15^2 −12^2 )) = 9 ∴ AB = 9 BCDF is a square of side 12 ⇒ ((DE)/(DF)) = ((EC)/(AC)) (ΔDEF is similar to ΔACE) ⇒ ((DE)/(12)) = ((DE+12)/(12+9)) ⇒ ((DE)/(12)) = ((DE+12)/(21)) ⇒ 21×DE = 12×(DE+12) ⇒ (21−12)×DE = 12×12 ⇒ 9×DF = 12×12 ∴ DE = 16 Similarly, (12+x)^2 = 12^2 +16^2 (Pythagoras theorem) 12+x = (√(400)) = 20 ∴ x = 8
$$\:\:\:\mathrm{FD}\:=\:\mathrm{BF}\:=\:\mathrm{12}\:\left(\mathrm{radii}\right) \\ $$$$\:\:\:\mathrm{AB}\:=\:\sqrt{\left(\mathrm{3}+\mathrm{12}\right)^{\mathrm{2}} −\mathrm{12}^{\mathrm{2}} }\:\:\:\left(\mathrm{Pythagoras}\:\mathrm{theorem}\right) \\ $$$$\:\:\:\mathrm{AB}\:\:=\:\sqrt{\mathrm{15}^{\mathrm{2}} −\mathrm{12}^{\mathrm{2}} }\:=\:\mathrm{9} \\ $$$$\:\:\:\therefore\:\:\:\:\:\:\:\mathrm{AB}\:=\:\mathrm{9} \\ $$$$\:\:\:\mathrm{BCDF}\:\mathrm{is}\:\mathrm{a}\:\mathrm{square}\:\mathrm{of}\:\:\mathrm{side}\:\mathrm{12} \\ $$$$\:\:\:\Rightarrow\:\:\:\frac{\mathrm{DE}}{\mathrm{DF}}\:=\:\frac{\mathrm{EC}}{\mathrm{AC}}\:\:\left(\Delta\mathrm{DEF}\:\mathrm{is}\:\mathrm{similar}\:\mathrm{to}\:\Delta\mathrm{ACE}\right) \\ $$$$\:\:\:\Rightarrow\:\:\:\frac{\mathrm{DE}}{\mathrm{12}}\:=\:\frac{\mathrm{DE}+\mathrm{12}}{\mathrm{12}+\mathrm{9}} \\ $$$$\:\:\:\Rightarrow\:\:\:\frac{\mathrm{DE}}{\mathrm{12}}\:=\:\frac{\mathrm{DE}+\mathrm{12}}{\mathrm{21}} \\ $$$$\:\:\:\Rightarrow\:\:\:\mathrm{21}×\mathrm{DE}\:=\:\mathrm{12}×\left(\mathrm{DE}+\mathrm{12}\right) \\ $$$$\:\:\:\Rightarrow\:\:\:\left(\mathrm{21}−\mathrm{12}\right)×\mathrm{DE}\:=\:\mathrm{12}×\mathrm{12} \\ $$$$\:\:\:\Rightarrow\:\:\:\mathrm{9}×\mathrm{DF}\:=\:\mathrm{12}×\mathrm{12} \\ $$$$\:\:\:\therefore\:\:\:\:\:\:\:\mathrm{DE}\:=\:\mathrm{16} \\ $$$$\:\:\:\mathrm{Similarly}, \\ $$$$\:\:\:\:\left(\mathrm{12}+{x}\right)^{\mathrm{2}} \:=\:\mathrm{12}^{\mathrm{2}} +\mathrm{16}^{\mathrm{2}} \:\:\:\left(\mathrm{Pythagoras}\:\mathrm{theorem}\right) \\ $$$$\:\:\:\:\mathrm{12}+{x}\:=\:\sqrt{\mathrm{400}}\:=\:\mathrm{20} \\ $$$$\:\:\:\therefore\:\:\:\:\:{x}\:=\:\mathrm{8} \\ $$
Commented by sandy_suhendra last updated on 06/Aug/17
Commented by Tinkutara last updated on 06/Aug/17
Thank you very much Sir!
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *