Question Number 18264 by Tinkutara last updated on 17/Jul/17

$$\mathrm{A}\:\mathrm{sky}\:\mathrm{diver}\:\mathrm{of}\:\mathrm{mass}\:{m}\:\mathrm{drops}\:\mathrm{out}\:\mathrm{with} \\ $$$$\mathrm{an}\:\mathrm{initial}\:\mathrm{velocity}\:{v}_{\mathrm{0}} \:=\:\mathrm{0}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{law} \\ $$$$\mathrm{by}\:\mathrm{which}\:\mathrm{the}\:\mathrm{sky}\:\mathrm{diver}'\mathrm{s}\:\mathrm{speed}\:\mathrm{varies} \\ $$$$\mathrm{before}\:\mathrm{the}\:\mathrm{parachute}\:\mathrm{is}\:\mathrm{opened}\:\mathrm{if}\:\mathrm{the} \\ $$$$\mathrm{drag}\:\mathrm{is}\:\mathrm{proportional}\:\mathrm{to}\:\mathrm{the}\:\mathrm{sky}\:\mathrm{diver}'\mathrm{s} \\ $$$$\mathrm{speed}.\:\mathrm{Also}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{problem}\:\mathrm{when}\:\mathrm{the} \\ $$$$\mathrm{sky}\:\mathrm{diver}'\mathrm{s}\:\mathrm{initial}\:\mathrm{velocity}\:\mathrm{has}\:\mathrm{horizontal} \\ $$$$\mathrm{component}\:{v}_{\mathrm{0}} \:\mathrm{and}\:\mathrm{vertical}\:\mathrm{component} \\ $$$$\mathrm{zero}. \\ $$
Answered by ajfour last updated on 16/Aug/17

Commented by ajfour last updated on 16/Aug/17
![let drag force be F^� =−kv^� As ΣF_y =((mdv_y )/dt) −kv_y −mg=m(dv_y /dt) ∫_0 ^( v_y ) ((kdv_y )/(kv_y +mg))=−(k/m)∫_0 ^( t) dt ⇒ ln (((kv_y +mg)/(mg)))=−((kt)/m) kv_y +mg=mge^(−kt/m) ⇒ v_y =−((mg)/k)(1−e^(−kt/m) ) ...(i) Further As ΣF_x =m(dv_x /dt) ⇒ −kv_x =((mdv_x )/dt) ∫_( v_0 ) ^( v_x ) (dv_x /v_x )=−((kt)/m)∫_0 ^( t) dt ⇒ ln (v_x /v_0 )=e^(−kt/m) ⇒ v_x =v_0 e^(−kt/m) ....(ii) As v^2 =v_x ^2 +v_y ^2 , so from (i)&(ii) v={v_0 ^2 e^(−2kt/m) +(((mg)/k))^2 [1−e^(−kt/m) ]^2 }^(1/2) If v_0 =0, v=((mg)/k)(1−e^(−kt/m) ) .](https://www.tinkutara.com/question/Q19864.png)
$$\:\:\:{let}\:{drag}\:{force}\:{be}\:\bar {{F}}=−{k}\bar {{v}} \\ $$$${As}\:\:\:\:\Sigma{F}_{{y}} =\frac{{mdv}_{{y}} }{{dt}} \\ $$$$\:\:\:\:\:−{kv}_{{y}} −{mg}={m}\frac{{dv}_{{y}} }{{dt}} \\ $$$$\:\:\:\:\int_{\mathrm{0}} ^{\:\:\boldsymbol{{v}}_{\boldsymbol{{y}}} } \frac{{kdv}_{{y}} }{{kv}_{{y}} +{mg}}=−\frac{{k}}{{m}}\int_{\mathrm{0}} ^{\:\:{t}} {dt} \\ $$$$\Rightarrow\:\:\:\:\mathrm{ln}\:\left(\frac{{kv}_{{y}} +{mg}}{{mg}}\right)=−\frac{{kt}}{{m}} \\ $$$$\:\:\:\:\:\:{kv}_{{y}} +{mg}={mge}^{−{kt}/{m}} \\ $$$$\Rightarrow\:\:\:\:\:\:\:{v}_{{y}} =−\frac{{mg}}{{k}}\left(\mathrm{1}−{e}^{−{kt}/{m}} \right)\:\:\:…\left(\boldsymbol{{i}}\right) \\ $$$${Further}\:{As}\:\:\Sigma{F}_{{x}} ={m}\frac{{dv}_{{x}} }{{dt}} \\ $$$$\Rightarrow\:\:\:\:\:−{kv}_{{x}} =\frac{{mdv}_{{x}} }{{dt}} \\ $$$$\:\:\:\:\:\:\int_{\:{v}_{\mathrm{0}} } ^{\:\:\boldsymbol{{v}}_{\boldsymbol{{x}}} } \:\frac{{dv}_{{x}} }{{v}_{{x}} }=−\frac{{kt}}{{m}}\int_{\mathrm{0}} ^{\:\:{t}} {dt} \\ $$$$\Rightarrow\:\:\:\:\mathrm{ln}\:\frac{{v}_{{x}} }{{v}_{\mathrm{0}} }={e}^{−{kt}/{m}} \\ $$$$\Rightarrow\:\:\:\:\:\:{v}_{{x}} ={v}_{\mathrm{0}} {e}^{−{kt}/{m}} \:\:\:\:….\left(\boldsymbol{{ii}}\right) \\ $$$$\:{As}\:\:\:{v}^{\mathrm{2}} ={v}_{{x}} ^{\mathrm{2}} +{v}_{{y}} ^{\mathrm{2}} \:\:\:,\:{so}\:{from}\:\left({i}\right)\&\left({ii}\right) \\ $$$$\:\boldsymbol{{v}}=\left\{\boldsymbol{{v}}_{\mathrm{0}} ^{\mathrm{2}} \boldsymbol{{e}}^{−\mathrm{2}\boldsymbol{{kt}}/\boldsymbol{{m}}} +\left(\frac{\boldsymbol{{mg}}}{\boldsymbol{{k}}}\right)^{\mathrm{2}} \left[\mathrm{1}−\boldsymbol{{e}}^{−\boldsymbol{{kt}}/\boldsymbol{{m}}} \right]^{\mathrm{2}} \:\right\}^{\mathrm{1}/\mathrm{2}} \\ $$$${If}\:{v}_{\mathrm{0}} =\mathrm{0},\:\:\:{v}=\frac{{mg}}{{k}}\left(\mathrm{1}−{e}^{−{kt}/{m}} \right)\:. \\ $$
Commented by Tinkutara last updated on 16/Aug/17
$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much}\:\mathrm{Sir}! \\ $$
Commented by Tinkutara last updated on 30/Aug/17
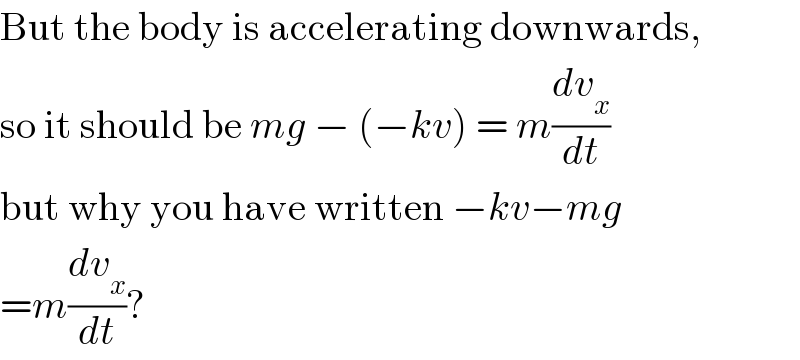
$$\mathrm{But}\:\mathrm{the}\:\mathrm{body}\:\mathrm{is}\:\mathrm{accelerating}\:\mathrm{downwards}, \\ $$$$\mathrm{so}\:\mathrm{it}\:\mathrm{should}\:\mathrm{be}\:{mg}\:−\:\left(−{kv}\right)\:=\:{m}\frac{{dv}_{{x}} }{{dt}} \\ $$$$\mathrm{but}\:\mathrm{why}\:\mathrm{you}\:\mathrm{have}\:\mathrm{written}\:−{kv}−{mg} \\ $$$$={m}\frac{{dv}_{{x}} }{{dt}}? \\ $$
Commented by ajfour last updated on 30/Aug/17

$${do}\:{notice}\:{that}\:\boldsymbol{{v}}_{\boldsymbol{{y}}} \:<\:\mathrm{0}\:{for}\:{the} \\ $$$${situation}\:{marked}\:{in}\:{diagram} \\ $$$${the}\:{eqation}\:{agrees}\:{to}\:{both}\:{when} \\ $$$${body}\:{is}\:{going}\:{up}\:{or}\:{coming}\:{down}. \\ $$$${drag}\:{force}\:{and}\:{v}_{{y}} \:{are}\:{oppositely} \\ $$$${directed}\:,\:{force}\:{of}\:{gravity} \\ $$$${downwards}.\:{i}\:{have}\:{solved}\: \\ $$$${for}\:{the}\:{two}\:{dimensional}\:{case} \\ $$$${right}\:{from}\:{the}\:{beginning}; \\ $$$$\Sigma{F}_{{y}} =−{mg}−{kv}_{{y}} ={m}\frac{{dv}_{{y}} }{{dt}} \\ $$$${i}\:{have}\:{written}.\:{when}\:{v}_{{y}} <\mathrm{0} \\ $$$${drag}\:{force}\:{component}\:−{kv}_{{y}} >\mathrm{0}\:. \\ $$
Commented by Tinkutara last updated on 31/Aug/17

$$\mathrm{OK}.\:\mathrm{Thanks}! \\ $$
