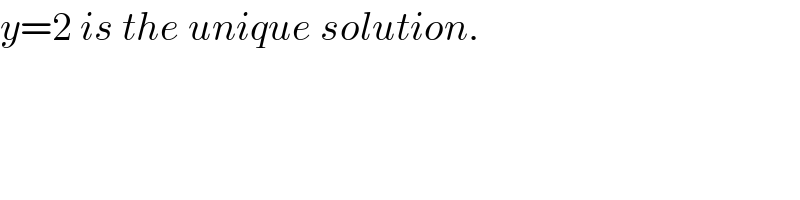Question Number 47457 by rahul 19 last updated on 10/Nov/18
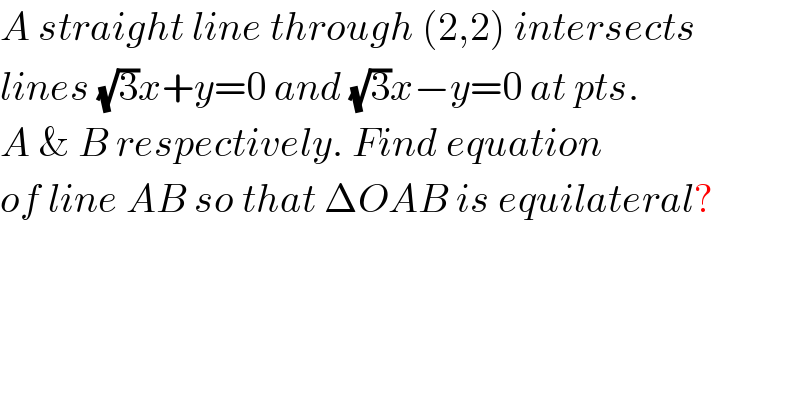
Answered by rahul 19 last updated on 10/Nov/18
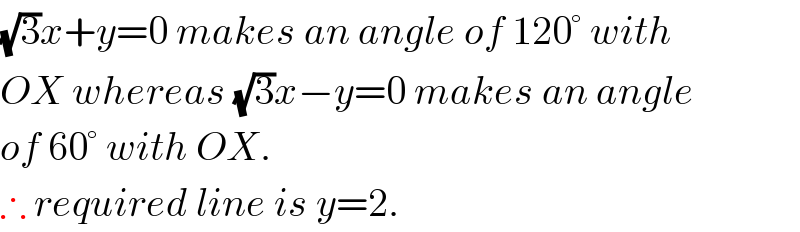
Commented by rahul 19 last updated on 10/Nov/18

Commented by rahul 19 last updated on 10/Nov/18

Commented by mr W last updated on 10/Nov/18