A-tangent-to-ellipse-x-2-a-2-y-2-b-2-1-at-point-p-meets-the-minor-axis-at-L-if-the-normal-at-p-meets-the-major-axis-at-m-find-the-locus-of-midpoint-LM-

Question Number 44988 by peter frank last updated on 07/Oct/18

A tangent to ellipse \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1 at point p meets the minor axis

at L if the normal at p meets the major axis at m . find the locus of midpoint LM

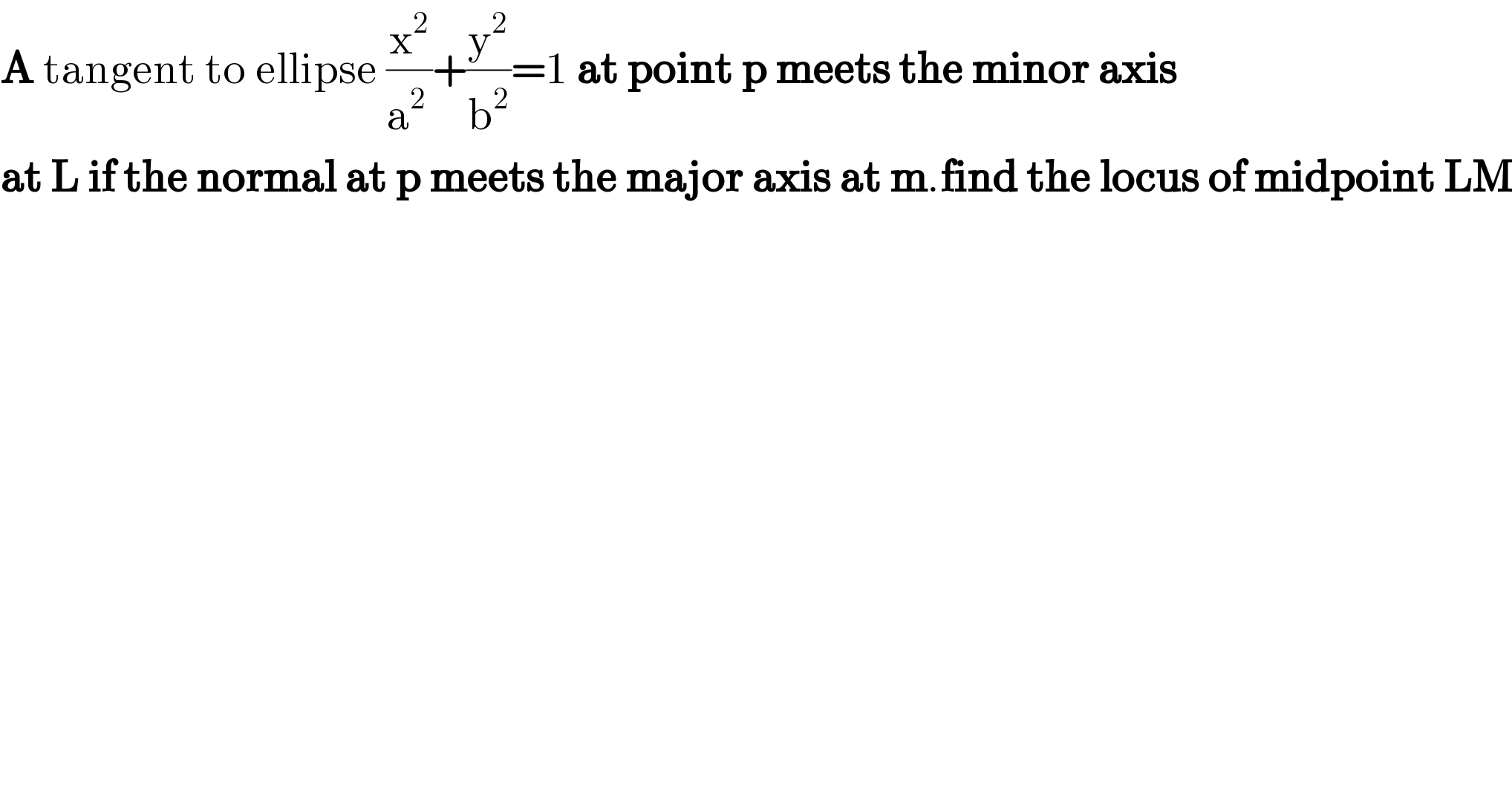
Answered by MrW3 last updated on 07/Oct/18

\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1

\frac{2 x}{a^{2}} + \frac{2 y}{b^{2}} \times \frac{d y}{d x} = 1

\frac{d y}{d x} = - \frac{b^{2} x}{a^{2} y}

P o i n t P (u, v)

\frac{u^{2}}{a^{2}} + \frac{v^{2}}{b^{2}} = 1 \dots (i)

e q n . o f t a n g e n t P L :

\frac{y - v}{x - u} = - \frac{b^{2} u}{a^{2} v}

P o i n t L (0, y_{1})

\frac{y_{1} - v}{- u} = - \frac{b^{2} u}{a^{2} v}

\Rightarrow y_{1} = v (1 + \frac{b^{2} u^{2}}{a^{2} v^{2}})

e q n . o f n o r m a l P M :

\frac{y - v}{x - u} = \frac{a^{2} v}{b^{2} u}

P o i n t M (x_{2}, 0)

\frac{- v}{x_{2} - u} = \frac{a^{2} v}{b^{2} u}

\Rightarrow x_{2} = u (1 - \frac{b^{2}}{a^{2}})

M i d p o i n t o f L M i s (p, q) w i t h

p = \frac{x_{2}}{2} = \frac{u}{2} (1 - \frac{b^{2}}{a^{2}}) = \frac{u (a^{2} - b^{2})}{2 a^{2}}

q = \frac{y_{1}}{2} = \frac{v}{2} (1 + \frac{b^{2} u^{2}}{a^{2} v^{2}}) = \frac{v}{2} (\frac{v^{2}}{b^{2}} + \frac{u^{2}}{a^{2}}) \frac{b^{2}}{v^{2}} = \frac{b^{2}}{2 v}

\Rightarrow u = \frac{2 a^{2} p}{a^{2} - b^{2}}

\Rightarrow v = \frac{b^{2}}{2 q}

p u t t i n g t h i s i n t o (i) :

\frac{4 a^{4} p^{2}}{(a^{2} - b^{2}) a^{2}} + \frac{b^{4}}{4 q^{2} b^{2}} = 1

\Rightarrow \frac{4 a^{2} p^{2}}{a^{2} - b^{2}} + \frac{b^{2}}{4 q^{2}} = 1

o r

\Rightarrow \frac{4 a^{2} x^{2}}{a^{2} - b^{2}} + \frac{b^{2}}{4 y^{2}} = 1

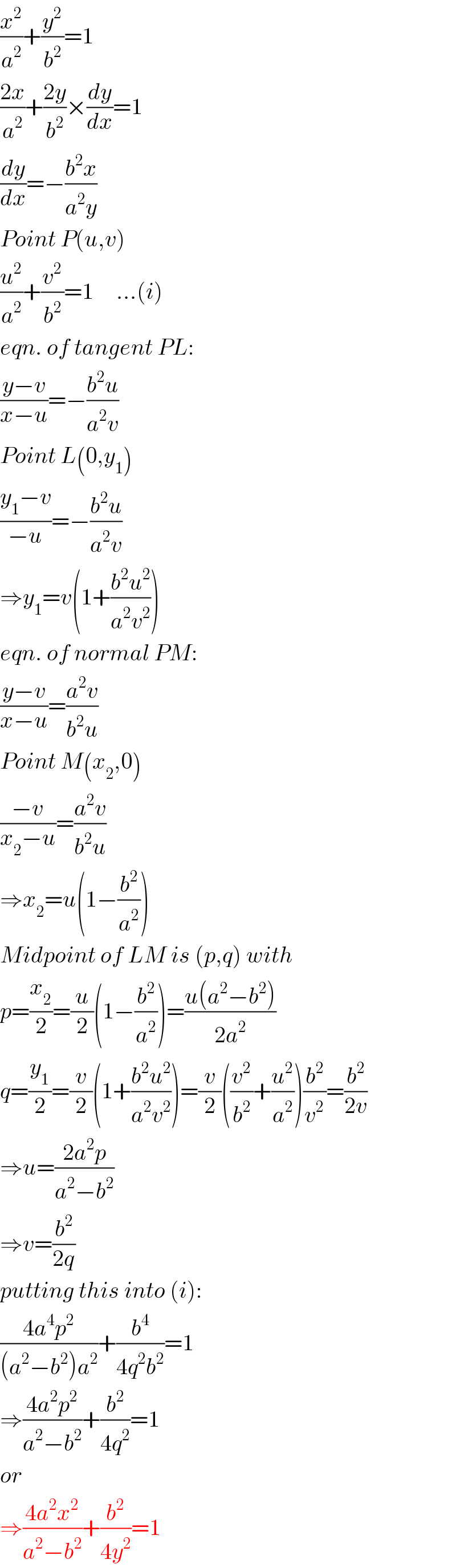
Commented by peter frank last updated on 07/Oct/18

thank you once gain sir . i really appreciate your effort

thank you once gain sir . i really appreciate your effort

Commented by MrW3 last updated on 07/Oct/18

Commented by MrW3 last updated on 07/Oct/18

Facebook Tweet Pin