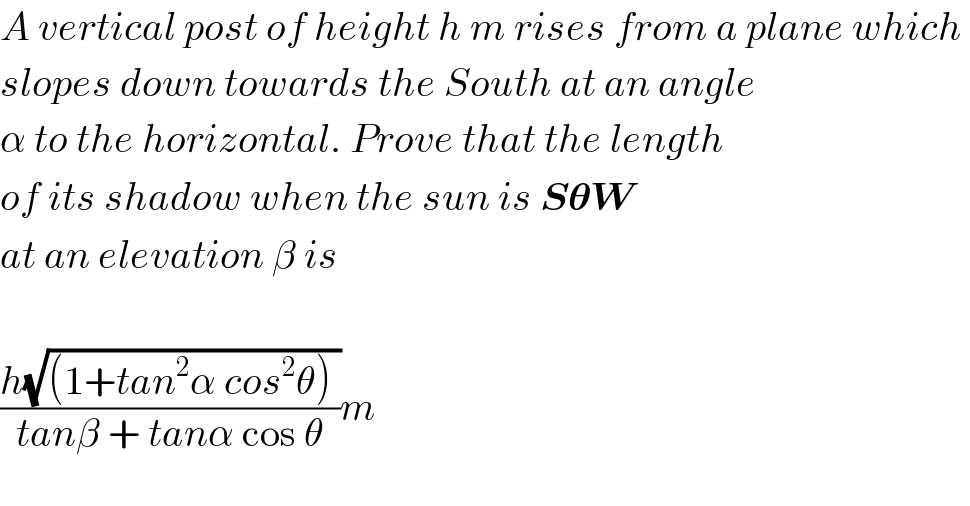
A-vertical-post-of-height-h-m-rises-from-a-plane-which-slopes-down-towards-the-South-at-an-angle-to-the-horizontal-Prove-that-the-length-of-its-shadow-when-the-sun-is-S-W-at-an-elevation-is-
Question Number 115642 by 24224 Opiyo Kamuki last updated on 27/Sep/20