Question Number 169145 by MathsFan last updated on 24/Apr/22

$$\boldsymbol{{ABC}}\:\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{a}}\:\boldsymbol{\mathrm{triangle}}\:\boldsymbol{\mathrm{in}}\:\boldsymbol{\mathrm{which}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{bisector}} \\ $$$$\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{angle}}\:\boldsymbol{\mathrm{at}}\:\boldsymbol{{B}}\:\boldsymbol{\mathrm{meet}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{side}}\:\boldsymbol{{AC}}\:\boldsymbol{\mathrm{at}}\:\boldsymbol{{D}}, \\ $$$$\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{bisector}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{angle}}\:\boldsymbol{{BDC}}\:\boldsymbol{\mathrm{is}} \\ $$$$\:\boldsymbol{\mathrm{parallel}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{side}}\:\boldsymbol{{AB}}.\:\boldsymbol{\mathrm{Prove}}\:\boldsymbol{\mathrm{that}} \\ $$$$\:\boldsymbol{\mathrm{the}}\:\bigtriangleup\boldsymbol{{ABC}}\:\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{issoceles}}\:\boldsymbol{\mathrm{triangle}}. \\ $$$$ \\ $$
Answered by som(math1967) last updated on 25/Apr/22
![let bisector of ∠BDC meet the side BC at E ∠ABD=∠DBE=α [BD bisector] ∠ABD=∠BDE [alternate ] ∠BDE=∠EDC=α [DE bisector] AB∥DE ∴ ∠EDC=∠BAC=α AC=b,AB=c, BC=a ∴ (a/(sinα))=(b/(sin2α)) ⇒(a/(sinα))=(b/(2sinαcosα)) ⇒cosα=(b/(2a)) ⇒((b^2 +c^2 −a^2 )/(2bc))=(b/(2a)) ⇒b^2 a+c^2 a−a^3 −b^2 c=0 ⇒b^2 (a−c) −a(a^2 −c^2 )=0 ⇒(a−c)(b^2 −ac−a^2 )=0 a−c=0 ⇒a=c triangle is isoscale](https://www.tinkutara.com/question/Q169152.png)
$${let}\:{bisector}\:{of}\:\angle{BDC}\:{meet}\:{the} \\ $$$${side}\:{BC}\:{at}\:{E} \\ $$$$\angle{ABD}=\angle{DBE}=\alpha\:\left[{BD}\:{bisector}\right] \\ $$$$\angle{ABD}=\angle{BDE}\:\left[{alternate}\:\right] \\ $$$$\angle{BDE}=\angle{EDC}=\alpha\:\left[{DE}\:{bisector}\right] \\ $$$${AB}\parallel{DE}\:\therefore\:\angle{EDC}=\angle{BAC}=\alpha \\ $$$$\:{AC}={b},{AB}={c},\:{BC}={a} \\ $$$$\therefore\:\frac{{a}}{{sin}\alpha}=\frac{{b}}{{sin}\mathrm{2}\alpha} \\ $$$$\Rightarrow\frac{{a}}{{sin}\alpha}=\frac{{b}}{\mathrm{2}{sin}\alpha{cos}\alpha} \\ $$$$\Rightarrow{cos}\alpha=\frac{{b}}{\mathrm{2}{a}} \\ $$$$\Rightarrow\frac{{b}^{\mathrm{2}} +{c}^{\mathrm{2}} −{a}^{\mathrm{2}} }{\mathrm{2}{bc}}=\frac{{b}}{\mathrm{2}{a}} \\ $$$$\Rightarrow{b}^{\mathrm{2}} {a}+{c}^{\mathrm{2}} {a}−{a}^{\mathrm{3}} −{b}^{\mathrm{2}} {c}=\mathrm{0} \\ $$$$\Rightarrow{b}^{\mathrm{2}} \left({a}−{c}\right)\:−{a}\left({a}^{\mathrm{2}} −{c}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\Rightarrow\left({a}−{c}\right)\left({b}^{\mathrm{2}} −{ac}−{a}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$${a}−{c}=\mathrm{0}\:\Rightarrow{a}={c} \\ $$$${triangle}\:{is}\:{isoscale}\: \\ $$
Commented by som(math1967) last updated on 25/Apr/22
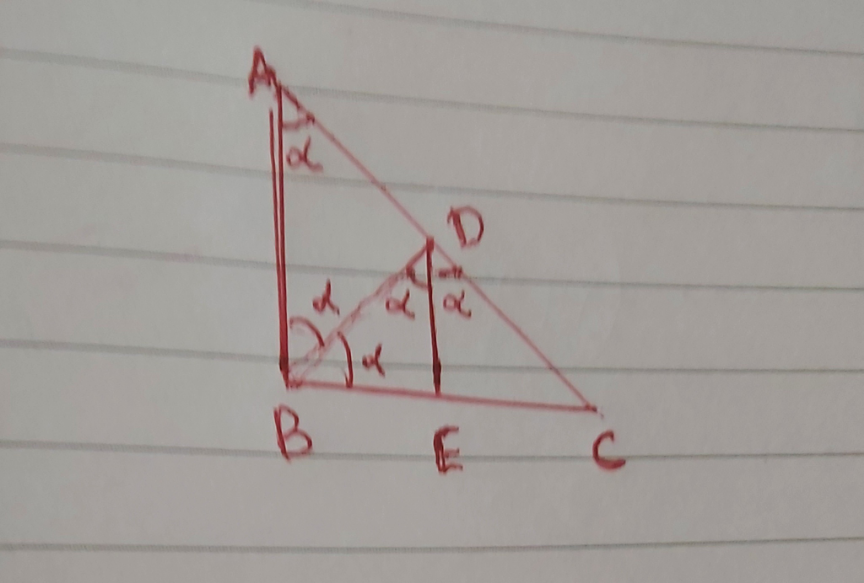
Commented by som(math1967) last updated on 25/Apr/22
$$\boldsymbol{{any}}\:\boldsymbol{{other}}\:\boldsymbol{{method}}\:\boldsymbol{{without}} \\ $$$$\boldsymbol{{trigonometry}}\:? \\ $$
Commented by MathsFan last updated on 25/Apr/22

$${thank}\:{you}\:{sir} \\ $$
