Menu Close

advanced-calculus-evaluate-0-cos-x-2-ln-x-dx-

Tinku Tara 0 Comments
Pin




Question Number 125922 by mnjuly1970 last updated on 15/Dec/20
...advanced calculus... evaluate ::: Ω=^(???) ∫_0 ^( ∞) cos(x^2 )ln(x)dx :::::::::
$$\:\:\:\:\:\:\:\:\:…{advanced}\:\:\:{calculus}… \\ $$$$\:\:\:\:\:\:\:{evaluate}\::::\:\:\:\Omega\overset{???} {=}\int_{\mathrm{0}} ^{\:\infty} {cos}\left({x}^{\mathrm{2}} \right){ln}\left({x}\right){dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\::::::::::\:\:\:\:\:\: \\ $$
Answered by mathmax by abdo last updated on 15/Dec/20
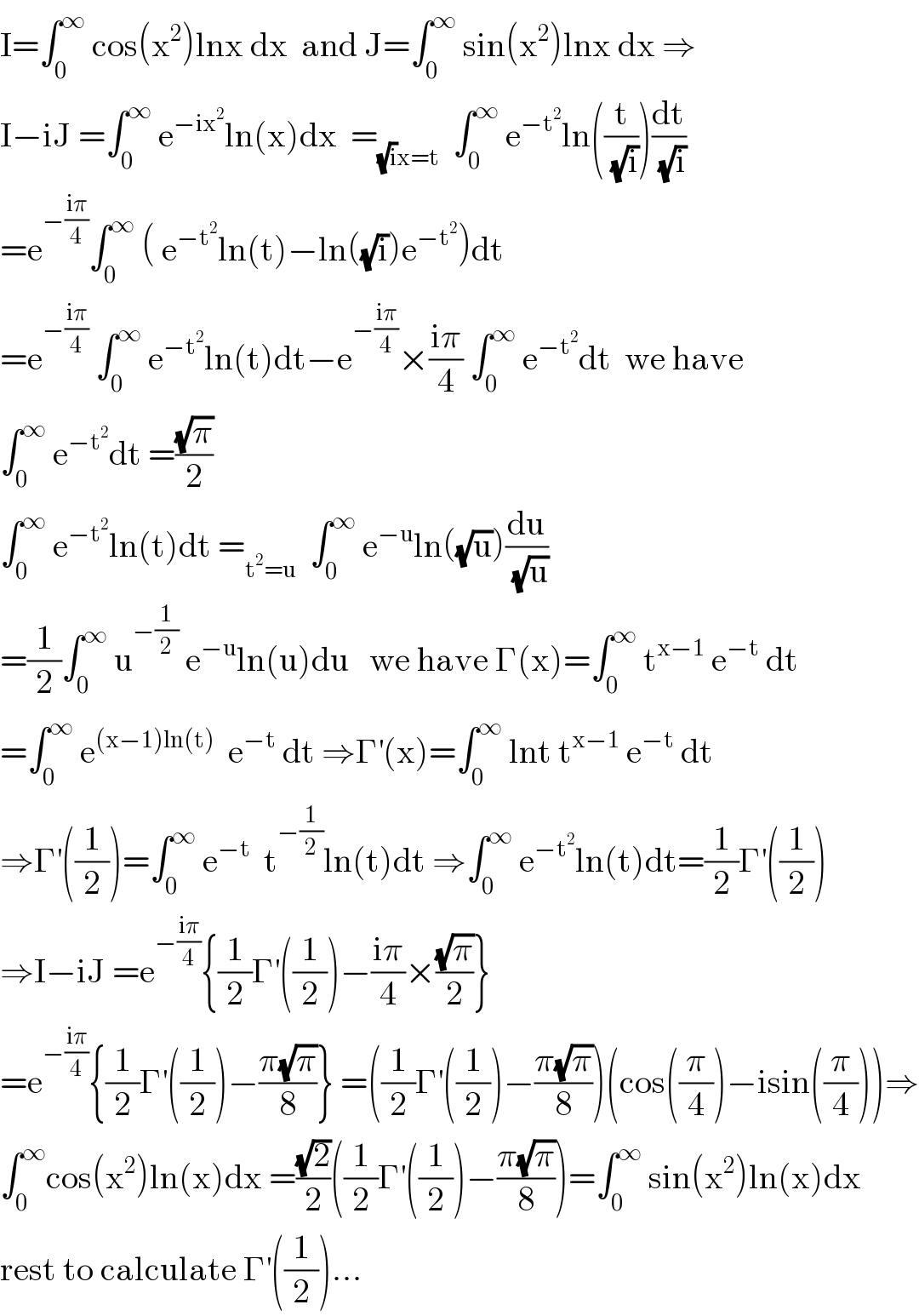
I=∫_0 ^∞ cos(x^2 )lnx dx and J=∫_0 ^∞ sin(x^2 )lnx dx ⇒ I−iJ =∫_0 ^∞ e^(−ix^2 ) ln(x)dx =_((√i)x=t) ∫_0 ^∞ e^(−t^2 ) ln((t/( (√i))))(dt/( (√i))) =e^(−((iπ)/4)) ∫_0 ^∞ ( e^(−t^2 ) ln(t)−ln((√i))e^(−t^2 ) )dt =e^(−((iπ)/4)) ∫_0 ^∞ e^(−t^2 ) ln(t)dt−e^(−((iπ)/4)) ×((iπ)/4) ∫_0 ^∞ e^(−t^2 ) dt we have ∫_0 ^∞ e^(−t^2 ) dt =((√π)/2) ∫_0 ^∞ e^(−t^2 ) ln(t)dt =_(t^2 =u) ∫_0 ^∞ e^(−u) ln((√u))(du/( (√u))) =(1/2)∫_0 ^∞ u^(−(1/2)) e^(−u) ln(u)du we have Γ(x)=∫_0 ^∞ t^(x−1) e^(−t) dt =∫_0 ^∞ e^((x−1)ln(t)) e^(−t) dt ⇒Γ^′ (x)=∫_0 ^∞ lnt t^(x−1) e^(−t) dt ⇒Γ^′ ((1/2))=∫_0 ^∞ e^(−t) t^(−(1/2)) ln(t)dt ⇒∫_0 ^∞ e^(−t^2 ) ln(t)dt=(1/2)Γ^′ ((1/2)) ⇒I−iJ =e^(−((iπ)/4)) {(1/2)Γ^′ ((1/2))−((iπ)/4)×((√π)/2)} =e^(−((iπ)/4)) {(1/2)Γ^′ ((1/2))−((π(√π))/8)} =((1/2)Γ^′ ((1/2))−((π(√π))/8))(cos((π/4))−isin((π/4)))⇒ ∫_0 ^∞ cos(x^2 )ln(x)dx =((√2)/2)((1/2)Γ^′ ((1/2))−((π(√π))/8))=∫_0 ^∞ sin(x^2 )ln(x)dx rest to calculate Γ^′ ((1/2))...
$$\mathrm{I}=\int_{\mathrm{0}} ^{\infty} \:\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{lnx}\:\mathrm{dx}\:\:\mathrm{and}\:\mathrm{J}=\int_{\mathrm{0}} ^{\infty} \:\mathrm{sin}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{lnx}\:\mathrm{dx}\:\Rightarrow \\ $$$$\mathrm{I}−\mathrm{iJ}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{ix}^{\mathrm{2}} } \mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx}\:\:=_{\sqrt{\mathrm{i}}\mathrm{x}=\mathrm{t}} \:\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{ln}\left(\frac{\mathrm{t}}{\:\sqrt{\mathrm{i}}}\right)\frac{\mathrm{dt}}{\:\sqrt{\mathrm{i}}} \\ $$$$=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \int_{\mathrm{0}} ^{\infty} \:\left(\:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{ln}\left(\mathrm{t}\right)−\mathrm{ln}\left(\sqrt{\mathrm{i}}\right)\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \right)\mathrm{dt} \\ $$$$=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{ln}\left(\mathrm{t}\right)\mathrm{dt}−\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} ×\frac{\mathrm{i}\pi}{\mathrm{4}}\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}\:\:\mathrm{we}\:\mathrm{have}\: \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{dt}\:=\frac{\sqrt{\pi}}{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{ln}\left(\mathrm{t}\right)\mathrm{dt}\:=_{\mathrm{t}^{\mathrm{2}} =\mathrm{u}} \:\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{u}} \mathrm{ln}\left(\sqrt{\mathrm{u}}\right)\frac{\mathrm{du}}{\:\sqrt{\mathrm{u}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \:\mathrm{u}^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\mathrm{e}^{−\mathrm{u}} \mathrm{ln}\left(\mathrm{u}\right)\mathrm{du}\:\:\:\mathrm{we}\:\mathrm{have}\:\Gamma\left(\mathrm{x}\right)=\int_{\mathrm{0}} ^{\infty} \:\mathrm{t}^{\mathrm{x}−\mathrm{1}} \:\mathrm{e}^{−\mathrm{t}} \:\mathrm{dt} \\ $$$$=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{\left(\mathrm{x}−\mathrm{1}\right)\mathrm{ln}\left(\mathrm{t}\right)} \:\:\mathrm{e}^{−\mathrm{t}} \:\mathrm{dt}\:\Rightarrow\Gamma^{'} \left(\mathrm{x}\right)=\int_{\mathrm{0}} ^{\infty} \:\mathrm{lnt}\:\mathrm{t}^{\mathrm{x}−\mathrm{1}} \:\mathrm{e}^{−\mathrm{t}} \:\mathrm{dt} \\ $$$$\Rightarrow\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}} \:\:\mathrm{t}^{−\frac{\mathrm{1}}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{t}\right)\mathrm{dt}\:\Rightarrow\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{t}^{\mathrm{2}} } \mathrm{ln}\left(\mathrm{t}\right)\mathrm{dt}=\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\Rightarrow\mathrm{I}−\mathrm{iJ}\:=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \left\{\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\mathrm{i}\pi}{\mathrm{4}}×\frac{\sqrt{\pi}}{\mathrm{2}}\right\} \\ $$$$=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \left\{\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{8}}\right\}\:=\left(\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{8}}\right)\left(\mathrm{cos}\left(\frac{\pi}{\mathrm{4}}\right)−\mathrm{isin}\left(\frac{\pi}{\mathrm{4}}\right)\right)\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\infty} \mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx}\:=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{8}}\right)=\int_{\mathrm{0}} ^{\infty} \:\mathrm{sin}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx} \\ $$$$\mathrm{rest}\:\mathrm{to}\:\mathrm{calculate}\:\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)… \\ $$
Commented by mnjuly1970 last updated on 15/Dec/20
great sir...
$${great}\:{sir}… \\ $$
Commented by mathmax by abdo last updated on 16/Dec/20
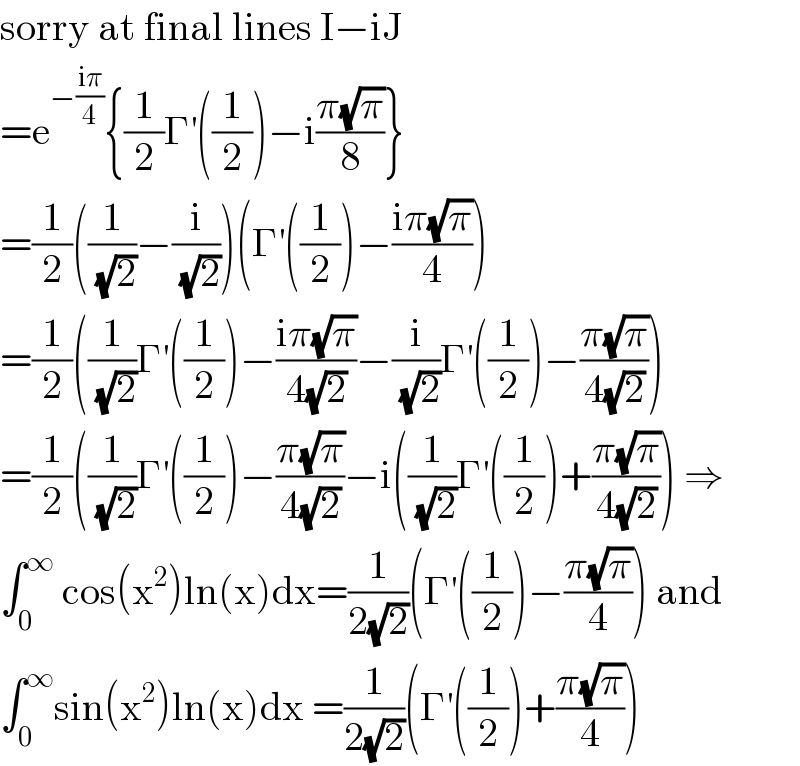
sorry at final lines I−iJ =e^(−((iπ)/4)) {(1/2)Γ^′ ((1/2))−i((π(√π))/8)} =(1/2)((1/( (√2)))−(i/( (√2))))(Γ^′ ((1/2))−((iπ(√π))/4)) =(1/2)((1/( (√2)))Γ^′ ((1/2))−((iπ(√π))/(4(√2)))−(i/( (√2)))Γ^′ ((1/2))−((π(√π))/(4(√2)))) =(1/2)((1/( (√2)))Γ^′ ((1/2))−((π(√π))/(4(√2)))−i((1/( (√2)))Γ^′ ((1/2))+((π(√π))/(4(√2)))) ⇒ ∫_0 ^∞ cos(x^2 )ln(x)dx=(1/(2(√2)))(Γ^′ ((1/2))−((π(√π))/4)) and ∫_0 ^∞ sin(x^2 )ln(x)dx =(1/(2(√2)))(Γ^′ ((1/2))+((π(√π))/4))
$$\mathrm{sorry}\:\mathrm{at}\:\mathrm{final}\:\mathrm{lines}\:\mathrm{I}−\mathrm{iJ} \\ $$$$=\mathrm{e}^{−\frac{\mathrm{i}\pi}{\mathrm{4}}} \left\{\frac{\mathrm{1}}{\mathrm{2}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\mathrm{i}\frac{\pi\sqrt{\pi}}{\mathrm{8}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\right)\left(\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\mathrm{i}\pi\sqrt{\pi}}{\mathrm{4}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\mathrm{i}\pi\sqrt{\pi}}{\mathrm{4}\sqrt{\mathrm{2}}}−\frac{\mathrm{i}}{\:\sqrt{\mathrm{2}}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{4}\sqrt{\mathrm{2}}}\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{4}\sqrt{\mathrm{2}}}−\mathrm{i}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\frac{\pi\sqrt{\pi}}{\mathrm{4}\sqrt{\mathrm{2}}}\right)\:\Rightarrow\right. \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\mathrm{cos}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left(\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)−\frac{\pi\sqrt{\pi}}{\mathrm{4}}\right)\:\mathrm{and} \\ $$$$\int_{\mathrm{0}} ^{\infty} \mathrm{sin}\left(\mathrm{x}^{\mathrm{2}} \right)\mathrm{ln}\left(\mathrm{x}\right)\mathrm{dx}\:=\frac{\mathrm{1}}{\mathrm{2}\sqrt{\mathrm{2}}}\left(\Gamma^{'} \left(\frac{\mathrm{1}}{\mathrm{2}}\right)+\frac{\pi\sqrt{\pi}}{\mathrm{4}}\right) \\ $$
Commented by mnjuly1970 last updated on 16/Dec/20
grateful sir max..
$${grateful}\:\:{sir}\:\:{max}.. \\ $$
Commented by mathmax by abdo last updated on 16/Dec/20
thankx sir
$$\mathrm{thankx}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *