Menu Close

asin-bcos-c-acosec-bsec-c-prove-that-sin2-2ab-c-2-a-2-b-2-

Tinku Tara 0 Comments
Pin




Question Number 102390 by Dwaipayan Shikari last updated on 08/Jul/20
asinθ+bcosθ=c acosecθ+bsecθ=c prove that sin2θ=((2ab)/(c^2 −a^2 −b^2 ))
$${asin}\theta+{bcos}\theta={c} \\ $$$${acosec}\theta+{bsec}\theta={c} \\ $$$$ \\ $$$${prove}\:{that}\:\:\:\:{sin}\mathrm{2}\theta=\frac{\mathrm{2}{ab}}{{c}^{\mathrm{2}} −{a}^{\mathrm{2}} −{b}^{\mathrm{2}} } \\ $$
Commented by PRITHWISH SEN 2 last updated on 08/Jul/20
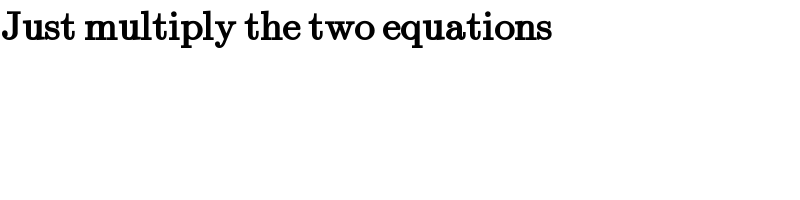
Just multiply the two equations
$$\boldsymbol{\mathrm{Just}}\:\boldsymbol{\mathrm{multiply}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{two}}\:\boldsymbol{\mathrm{equations}}\: \\ $$
Commented by bobhans last updated on 09/Jul/20
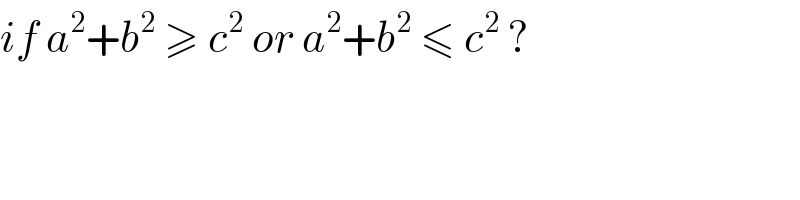
if a^2 +b^2 ≥ c^2 or a^2 +b^2 ≤ c^2 ?
$${if}\:{a}^{\mathrm{2}} +{b}^{\mathrm{2}} \:\geqslant\:{c}^{\mathrm{2}} \:{or}\:{a}^{\mathrm{2}} +{b}^{\mathrm{2}} \:\leqslant\:{c}^{\mathrm{2}} \:? \\ $$
Commented by bemath last updated on 09/Jul/20
(2)(a/(sin θ))+(b/(cos θ)) = c ((acos θ+bsin θ)/((1/2)sin 2θ)) = c ⇒acos θ+bsin θ=(1/2)c sin 2θ (1)×(2) (asin θ+bcos θ)(acos θ+bsin θ)=(1/2)c^2 sin 2θ (1/2)a^2 sin 2θ+absin^2 θ+abcos^2 θ+(1/2)b^2 sin 2θ=(1/2)c^2 sin 2θ {(1/2)a^2 +(1/2)b^2 }sin 2θ+ab=(1/2)c^2 sin 2θ ab = {(1/2)c^2 −(1/2)a^2 −(1/2)b^2 } sin 2θ sin 2θ = ((2ab)/(c^2 −a^2 −b^2 ))
$$\left(\mathrm{2}\right)\frac{{a}}{\mathrm{sin}\:\theta}+\frac{{b}}{\mathrm{cos}\:\theta}\:=\:{c}\: \\ $$$$\frac{{a}\mathrm{cos}\:\theta+{b}\mathrm{sin}\:\theta}{\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\:\mathrm{2}\theta}\:=\:{c}\:\Rightarrow{a}\mathrm{cos}\:\theta+{b}\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\mathrm{2}}{c}\:\mathrm{sin}\:\mathrm{2}\theta \\ $$$$\left(\mathrm{1}\right)×\left(\mathrm{2}\right)\: \\ $$$$\left({a}\mathrm{sin}\:\theta+{b}\mathrm{cos}\:\theta\right)\left({a}\mathrm{cos}\:\theta+{b}\mathrm{sin}\:\theta\right)=\frac{\mathrm{1}}{\mathrm{2}}{c}^{\mathrm{2}} \:\mathrm{sin}\:\mathrm{2}\theta \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}{a}^{\mathrm{2}} \mathrm{sin}\:\mathrm{2}\theta+{ab}\mathrm{sin}\:^{\mathrm{2}} \theta+{ab}\mathrm{cos}\:^{\mathrm{2}} \theta+\frac{\mathrm{1}}{\mathrm{2}}{b}^{\mathrm{2}} \mathrm{sin}\:\mathrm{2}\theta=\frac{\mathrm{1}}{\mathrm{2}}{c}^{\mathrm{2}} \:\mathrm{sin}\:\mathrm{2}\theta \\ $$$$\left\{\frac{\mathrm{1}}{\mathrm{2}}{a}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}{b}^{\mathrm{2}} \right\}\mathrm{sin}\:\mathrm{2}\theta+{ab}=\frac{\mathrm{1}}{\mathrm{2}}{c}^{\mathrm{2}} \:\mathrm{sin}\:\mathrm{2}\theta \\ $$$${ab}\:=\:\left\{\frac{\mathrm{1}}{\mathrm{2}}{c}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}{a}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}{b}^{\mathrm{2}} \right\}\:\mathrm{sin}\:\mathrm{2}\theta \\ $$$$\mathrm{sin}\:\mathrm{2}\theta\:=\:\frac{\mathrm{2}{ab}}{{c}^{\mathrm{2}} −{a}^{\mathrm{2}} −{b}^{\mathrm{2}} } \\ $$
Commented by 1549442205 last updated on 09/Jul/20
$$ \\ $$
Answered by Dwaipayan Shikari last updated on 08/Jul/20
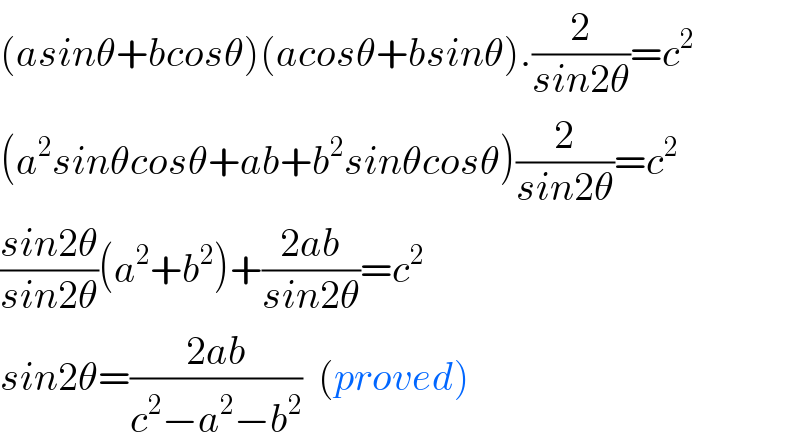
(asinθ+bcosθ)(acosθ+bsinθ).(2/(sin2θ))=c^2 (a^2 sinθcosθ+ab+b^2 sinθcosθ)(2/(sin2θ))=c^2 ((sin2θ)/(sin2θ))(a^2 +b^2 )+((2ab)/(sin2θ))=c^2 sin2θ=((2ab)/(c^2 −a^2 −b^2 )) (proved)
$$\left({asin}\theta+{bcos}\theta\right)\left({acos}\theta+{bsin}\theta\right).\frac{\mathrm{2}}{{sin}\mathrm{2}\theta}={c}^{\mathrm{2}} \\ $$$$\left({a}^{\mathrm{2}} {sin}\theta{cos}\theta+{ab}+{b}^{\mathrm{2}} {sin}\theta{cos}\theta\right)\frac{\mathrm{2}}{{sin}\mathrm{2}\theta}={c}^{\mathrm{2}} \\ $$$$\frac{{sin}\mathrm{2}\theta}{{sin}\mathrm{2}\theta}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)+\frac{\mathrm{2}{ab}}{{sin}\mathrm{2}\theta}={c}^{\mathrm{2}} \\ $$$${sin}\mathrm{2}\theta=\frac{\mathrm{2}{ab}}{{c}^{\mathrm{2}} −{a}^{\mathrm{2}} −{b}^{\mathrm{2}} }\:\:\left({proved}\right) \\ $$
Commented by bobhans last updated on 09/Jul/20
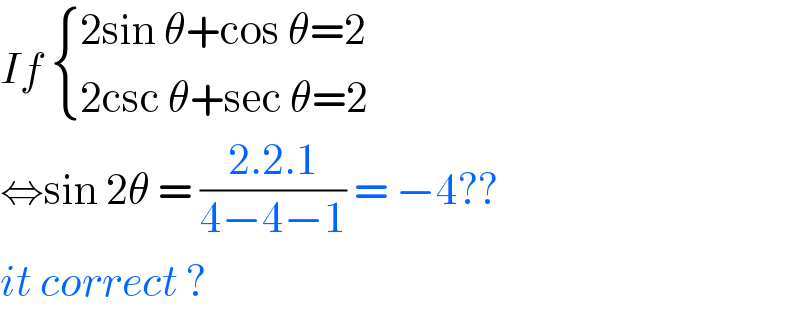
If { ((2sin θ+cos θ=2)),((2csc θ+sec θ=2)) :} ⇔sin 2θ = ((2.2.1)/(4−4−1)) = −4?? it correct ?
$${If}\:\begin{cases}{\mathrm{2sin}\:\theta+\mathrm{cos}\:\theta=\mathrm{2}}\\{\mathrm{2csc}\:\theta+\mathrm{sec}\:\theta=\mathrm{2}}\end{cases} \\ $$$$\Leftrightarrow\mathrm{sin}\:\mathrm{2}\theta\:=\:\frac{\mathrm{2}.\mathrm{2}.\mathrm{1}}{\mathrm{4}−\mathrm{4}−\mathrm{1}}\:=\:−\mathrm{4}??\: \\ $$$${it}\:{correct}\:?\: \\ $$
Commented by 1549442205 last updated on 09/Jul/20
but the your system has no roots ! the first eqs implies that sin2θ=((24)/(25))!
$$\mathrm{but}\:\mathrm{the}\:\mathrm{your}\:\mathrm{system}\:\mathrm{has}\:\mathrm{no}\:\mathrm{roots}\:! \\ $$$$\mathrm{the}\:\mathrm{first}\:\mathrm{eqs}\:\mathrm{implies}\:\mathrm{that}\:\mathrm{sin2}\theta=\frac{\mathrm{24}}{\mathrm{25}}! \\ $$
Commented by PRITHWISH SEN 2 last updated on 09/Jul/20
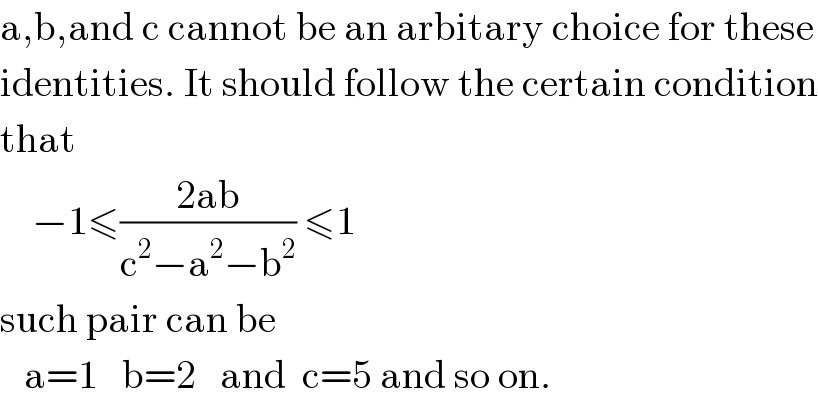
a,b,and c cannot be an arbitary choice for these identities. It should follow the certain condition that −1≤((2ab)/(c^2 −a^2 −b^2 )) ≤1 such pair can be a=1 b=2 and c=5 and so on.
$$\mathrm{a},\mathrm{b},\mathrm{and}\:\mathrm{c}\:\mathrm{cannot}\:\mathrm{be}\:\mathrm{an}\:\mathrm{arbitary}\:\mathrm{choice}\:\mathrm{for}\:\mathrm{these} \\ $$$$\mathrm{identities}.\:\mathrm{It}\:\mathrm{should}\:\mathrm{follow}\:\mathrm{the}\:\mathrm{certain}\:\mathrm{condition} \\ $$$$\mathrm{that} \\ $$$$\:\:\:\:−\mathrm{1}\leqslant\frac{\mathrm{2ab}}{\mathrm{c}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} −\mathrm{b}^{\mathrm{2}} }\:\leqslant\mathrm{1} \\ $$$$\mathrm{such}\:\mathrm{pair}\:\mathrm{can}\:\mathrm{be} \\ $$$$\:\:\:\mathrm{a}=\mathrm{1}\:\:\:\mathrm{b}=\mathrm{2}\:\:\:\mathrm{and}\:\:\mathrm{c}=\mathrm{5}\:\mathrm{and}\:\mathrm{so}\:\mathrm{on}. \\ $$
Commented by bemath last updated on 09/Jul/20
it does meant c^2 ≥ a^2 +b^2
$${it}\:{does}\:{meant}\:{c}^{\mathrm{2}} \:\geqslant\:{a}^{\mathrm{2}} +{b}^{\mathrm{2}} \\ $$
Answered by 1549442205 last updated on 09/Jul/20
(1)⇔(a/( (√(a^2 +b^2 ))))sinθ+(b/( (√(a^2 +b^2 ))))cosθ=(c/( (√(a^2 +b^2 )))) ⇔sin(ϕ+θ)=sin[sin^(−1) ((c/( (√(a^2 +b^2 )))))](where ϕ=arccos(a/( (√(a^2 +b^2 ))))) (with the condition a^2 +b^2 ≥c^2 ) ⇒ϕ+θ=sin^(−1) ((c/( (√(a^2 +b^2 )))))+2kπ ⇒θ=sin^(−1) ((c/( (√(a^2 +b^2 )))))+2kπ−ϕ ⇒sinθ=sin(sin^(−1) ((c/( (√(a^2 +b^2 )))))−ϕ) =(c/( (√(a^2 +b^2 ))))cosϕ−((√(a^2 +b^2 −c^2 ))/( (√(a^2 +b^2 ))))sinϕ =(c/( (√(a^2 +b^2 )))).(a/( (√(a^2 +b^2 ))))−((√(a^2 +b^2 −c^2 ))/( (√(a^2 +b^2 )))).(b/( (√(a^2 +b^2 )))) =((ac−b(√(a^2 +b^2 −c^2 )))/(a^2 +b^2 )) ⇒cosθ=(1/(a^2 +b^2 ))(√((a^2 +b^2 )^2 −(ac−b(√(a^2 +b^2 −c^2 )^2 )))) ⇒sin2θ=2sinθcosθ=2(((ac−b(√(a^2 +b^2 −c^2 )))/(a^2 +b^2 )))((1/(a^2 +b^2 ))(√((a^2 +b^2 )^2 −(ac−b(√(a^2 +b^2 −c^2 )^2 )))) )=f(a,b,c)
$$\left(\mathrm{1}\right)\Leftrightarrow\frac{\mathrm{a}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\mathrm{sin}\theta+\frac{\mathrm{b}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\mathrm{cos}\theta=\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }} \\ $$$$\Leftrightarrow\mathrm{sin}\left(\varphi+\theta\right)=\mathrm{sin}\left[\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\right)\right]\left(\mathrm{where}\:\varphi=\mathrm{arccos}\frac{\mathrm{a}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\right) \\ $$$$\left(\mathrm{with}\:\mathrm{the}\:\mathrm{condition}\:\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \geqslant\mathrm{c}^{\mathrm{2}} \right) \\ $$$$\Rightarrow\varphi+\theta=\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\right)+\mathrm{2k}\pi \\ $$$$\Rightarrow\theta=\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\right)+\mathrm{2k}\pi−\varphi \\ $$$$\Rightarrow\mathrm{sin}\theta=\mathrm{sin}\left(\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\right)−\varphi\right) \\ $$$$=\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\mathrm{cos}\varphi−\frac{\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} }}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}\mathrm{sin}\varphi \\ $$$$=\frac{\mathrm{c}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}.\frac{\mathrm{a}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}−\frac{\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} }}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }}.\frac{\mathrm{b}}{\:\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }} \\ $$$$=\frac{\mathrm{ac}−\mathrm{b}\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} }}{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} } \\ $$$$\Rightarrow\mathrm{cos}\theta=\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }\sqrt{\left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \right)^{\mathrm{2}} −\left(\mathrm{ac}−\mathrm{b}\sqrt{\left.\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} \right)^{\mathrm{2}} }\right.} \\ $$$$\Rightarrow\mathrm{sin2}\theta=\mathrm{2sin}\theta\mathrm{cos}\theta=\mathrm{2}\left(\frac{\mathrm{ac}−\mathrm{b}\sqrt{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} }}{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }\right)\left(\frac{\mathrm{1}}{\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} }\sqrt{\left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \right)^{\mathrm{2}} −\left(\mathrm{ac}−\mathrm{b}\sqrt{\left.\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} −\mathrm{c}^{\mathrm{2}} \right)^{\mathrm{2}} }\right.}\:\right)=\mathrm{f}\left(\mathrm{a},\mathrm{b},\mathrm{c}\right) \\ $$$$ \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *